4
$\begingroup$

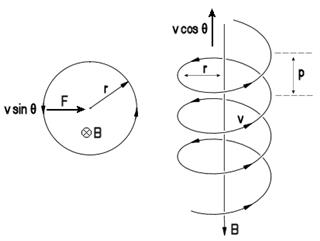
A charged particle $q$ enters a uniform magnetic field $\vec{B}$ with velocity $\vec{v}$ making an angle $\theta$ with it. Since the Lorentz force is perpendicular to the velocity, the particle will move along a circular path of radius $r$, which my textbook derives as follows:

$$\frac{mv^2}{r}=qvB \sin\theta$$ $$r=\frac{mv}{qB\sin\theta}.$$

But I think the correct formula for $r$ should be derived as follows:

$$\frac{m(v\sin\theta)^2}{r}=qvB \sin\theta$$ $$r=\frac{mv\sin\theta}{qB}.$$

This should be because we only consider the perpendicular component of velocity when we calculate magnetic force and therefore the velocity to which the force is perpendicular is the component of velocity perpendicular to $\vec{B}$ and not $\vec{v}$.

Which is the correct formula?

Improve this question
$\endgroup$

3 Answers 3

Reset to default
4
$\begingroup$

Your derivation is correct and your book is incorrect unless the $v$ in their equation is the component of velocity perpendicular to the magnetic field?
The diagram below assumes a positive charge.

enter image description here

The radius of the circular motion is given by the equation $r=\dfrac{mv\sin\theta}{qB}$ and the pitch of the helix is $p = \dfrac{2\pi mv\cos \theta}{qB}$

Improve this answer
$\endgroup$
5
  • 2
    $\begingroup$ Why then does the particle describe helical motion? The velocity at any point in this case would not be parallel to the plane of circular motion. $\endgroup$
    – Samama Fahim
    Commented Feb 13, 2017 at 16:31
  • 1
    $\begingroup$ What makes you think that the motion is helical as the only force on the charge is the one that produces the centripetal acceleration of the charge? $\endgroup$
    – Farcher
    Commented Feb 13, 2017 at 16:32
  • 2
    $\begingroup$ If the particle has a component of its motion along the field direction, that motion is constant, since there can be no component of the magnetic force in the direction of the field. The general motion of a particle in a uniform magnetic field is a constant velocity parallel to $\vec{B}$ and a circular motion at right angles to $\vec{B}$—the trajectory is a cylindrical helix. - Feynman Lectures. Where do I misunderstand this? $\endgroup$
    – Samama Fahim
    Commented Feb 13, 2017 at 16:47
  • 2
    $\begingroup$ @OmarAbdullah I am sorry. You (and Feynman) are correct and I have amended my answer. $\endgroup$
    – Farcher
    Commented Feb 13, 2017 at 17:18
  • 1
    $\begingroup$ The $\vec{v}$ in the equation in my book is the actual velocity. Thanks for confirmation. $\endgroup$
    – Samama Fahim
    Commented Feb 13, 2017 at 18:01
1
$\begingroup$

It is easy to see that the book answer r = mv/qBsin θ is correct.

Ask yourself what happens to the radius as the strength of the magnetic field decreases. Since the component of the magnetic field producing a force on the charge is smaller than 1.0 for any θ less than 90 degrees the book's formula correctly describes what happens for angles other than 90 degrees.

The error in multiplying the velocity by sin θ, rather than the magnetic field is that you are only considering the component of the velocity that is perpendicular to the field. You have erroneously ignored the effect that the total velocity has on the circumference of the circle.

Also the sin θ factor only applies to the force on a charge that is moving perpendicular to a magnetic field. It does not apply to the force required to change the direction of a moving mass that happens to have a charge.

Improve this answer
$\endgroup$
1
-2
$\begingroup$

enter image description here.this is the ans for the question

Improve this answer
$\endgroup$
1
  • 4
    $\begingroup$ Please type out your answer, rather than just posting a picture. $\endgroup$
    – Chris ♦
    Commented Jul 21, 2019 at 21:55

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.