However, while the round trip time $t_1'+t_2'=2\gamma=\gamma(t_1+t_2)$
is as expected from time dilation, the time differences
$t_1'-t_2'\neq\gamma(t_1-t_2)=0$ where the opposite is true in the
Sagnac circular setting. What is amiss? I suspect it is the simultaneity
which is related to precisely the time sum t′1+t′2
that is the culprit.
The problem is to do with conventions used for synchronising. In the linear setting, the Einstein clock synchronisation convention assumes the speed of light is isotropic. This is fine in the linear setting as there is no way to measure the the one way speed of light in the linear case. In the circular case, the speed of light is not isotropic in the rotating reference frame. A signal going all the way round clockwise takes longer to return to the start than a signal going anti-clockwise, if the disc is rotating clockwise. You can however use Einstein synchronisation in the circular setting and then get $\gamma(t_1-t_2) = 0 $ as you would expect. The only problem is that you need one sets of clocks with an advanced offset around the perimeter to measure clockwise signals and another set of clocks with retarded offsets to measure anticlockwise signals. We end up with the strange situation of clocks that are adjacent to each other with different offsets. This situation arises from trying to maintain the illusion that the light is isotropic in a rotating reference frame, when it is not.
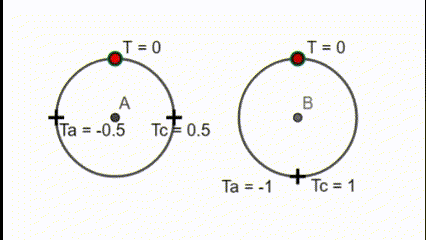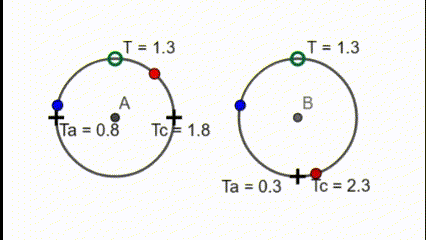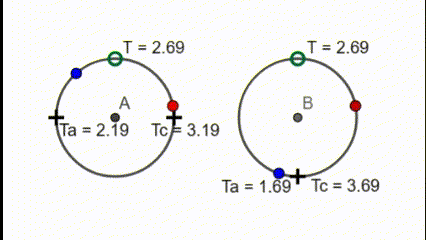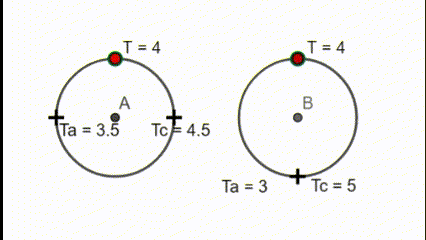
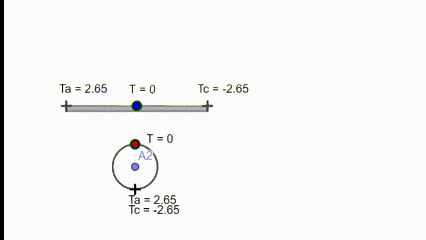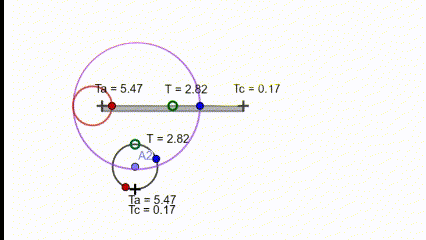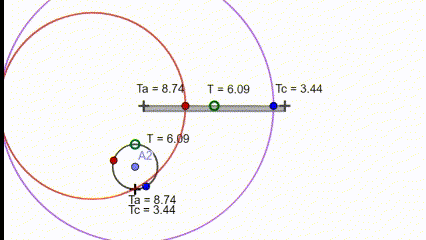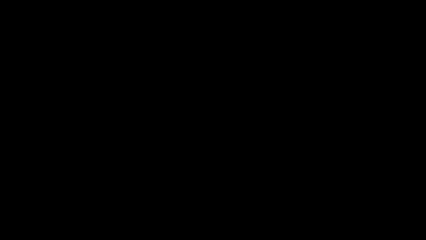
In the above animation, the clocks are synchronised exactly as per the Einstein convention, by setting the clocks at the mirrors to half the round trip time. The disc is rotating anticlockwise, so its takes longer for anticlockwise signal to reach the mirror, so the anticlockwise clocks (Ta) have a negative offset and the clockwise clocks have a positive offset (Tc). This means Ta=Tc or equivalently, $\gamma(t_1-t_2) = 0 $. Everything is as per the linear case, but when the signals cross each other, the different offsets become obvious. You can imagine cutting the ring in half at the lower section and bending the circle into a straight line. You would end up with the linear case. An alternative method of synchronising the clocks is what I call the lighthouse method. All the clocks are set to zero simultaneously by a signal from the centre. It would become obvious then the light signals are not isotropic in the rotating reference frame. This is not too radical a concept. In the Schwarzschild metric, the speed of light is not isotropic either to a stationary observer in the gravitational field, except very locally.
(Just in case it not clear, the two clocks at the lower part of the right hand animation are at the same location.) The signals arrive 'simultaneously' at that location, if you read the right clock, but of course it is obvious that they do not really arrive simultaneously, which is a consequence of the reality, that the speed of light is direction dependent in a rotating reference frame.
The above animation more directly demonstrates the equivalence of the linear and Sagnac forms. Note that despite the one way speed of light being c in both directions in the linear case and effectively 3/4 c clockwise and 2 c anti-clockwise in the rotating case, the measured times are identical. This demonstrates the complete insensitivity off Einstein synchronisation to the one way speed of light.
How would one actually compute t1 and t2 in the comoving frame in the
circular setting? – Hans
As shown above if Einstein synchronisation is used in the circular setting, the calculations and results are exactly the same as in the linear case. If the rotating clocks are synchronised by a central signal then what appears to be simultaneous to the co-rotating obverses also appear s synchronised to a none rotating observer that is stationary relative to the centre the rotating frame.
Using this method,
$t_1 = \frac{L_0}{c\gamma (1-\beta)}$
$t_2 = \frac{L_0}{c\gamma (1+\beta)}$
where $L_0$ = the proper length of the rod or the proper distance along the perimeter.
$$t'_1-t'_2 = \left[\frac{L_0}{c\gamma (1-\beta)}\right] -\left[ \frac{L_0}{c\gamma (1+\beta)}\right] = 2L_O \frac{\beta \gamma}{c}= (t_1+t_2) \beta \gamma $$
where $L_0 = c(t1+t_2)/2$ (The there and back time is halved)
You made a small error or omission in your addition calculation where you got $t_1'+t_2'=2\gamma\ $ which should be $$t_1'+t_2'=2L_0\frac{\gamma}{c}=2L_0\frac{\gamma}{c}$$
which is now dimensionally correct.


