Is the current induced in coil in Faraday's experiment displacement current?

Question

In Faraday's experiment-

Only if we have a imaginary coil rather than a real coil, then change of magnetic flux through that imaginary coil and electric field induced in the imaginary coil/region should be related by- $$\oint \vec{E}.\vec{dl}= -\frac{d\phi_B}{dt}$$ This will give rise to induced emf in imaginary coil and hence current (I) in the imaginary region.

The Question is can i call this current I as displacement current or rather is this current displacement current?

Background

I developed this doubt because of a problem where displacement current was to be found r distance(in perpendicular direction) away from a infinite long solenoid through which a time varying current was passing producing a time varing $B$ inside the solenoid ($B \propto t^2)$

Answer 1

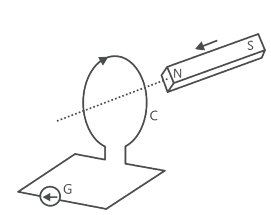
This will give rise to induced emf in imaginary coil [...]

Yes, induced EMF due to magnet is there, defined for any closed path $\gamma$ as

$$ \oint_\gamma \mathbf E\cdot d\mathbf s, $$ whether that path goes through a conductor, or a vacuum.

[...] and hence current (I) in the imaginary region.

No, there won't be a current $I$, because the path is entirely in a vacuum, which does not conduct current.

There will be a displacement current, but:

1. "displacement current" is just a weird name (used for historical reasons) for the surface integral $$ \int_S d\mathbf S \cdot \frac{\partial (\epsilon_0 \mathbf E + \mathbf P)}{\partial t} $$ for some surface $S$;

2. this is not a current in the "circuit" sense of the word (motion of electric charge along a closed path);

3. from definition, we can see that in your imaginary coil example, the displacement current does not go along the imaginary coil curve, but it goes through the surface we consider. We can consider a surface which ends on the imaginary circuit closed path, but the displacement current still goes through the surface, not along the closed path.

Maxwell called it a displacement current, because he thought primarily of a dielectric medium where in presence of electric field, electric particles can be displaced from their normal position, and even in vacuum he imagined movable particles (the explanation for why he chose the name "displacement current" is difficult and tedious). In vacuum, there is no actual displacement of electric particles going on.

The Question is can i call this current I as displacement current or rather is this current displacement current?

Again, there is no current going along the imaginary closed path. There is a displacement current going through the surface defined by the closed path. It depends on the surface; different surface may give different displacement current.

Background

I developed this doubt because of a problem where ?>displacement current was to be found r distance(in >perpendicular direction) away from a infinite long >solenoid through which a time varying current was >passing producing a time varing B inside the solenoid (B∝t2)

Perhaps ask about this in a new question, so it's clear what the system and the problem is.

Answer 2

In order to have displacement current equal to the real current in a real conductor, the voltage integral along the conductor given by derivative of the area flux wrt. time has to be equal to resistance $\times$ current.

But that real induced current reduces the B-field by Lenz's law.

So its definition is the product of $I\times R$ in the limit $R\to \infty, I\to 0$.

As always, an ideal measurement method induces minimal change of the defining parameters of the experiment.

Fortunately, coils of some 1000 windings of hair thin copper wires made the experiments possible; coils of the same quality were used as pointers in $\mu A$ -meters at the end of the 19th century.