The trouble with EMF induced in the coil of wire

Question

For anyone viewing this question who is not familiar with the notion of Induced EMF in the coil of wire let me briefly explain what i understood by it.

EMF is induced inside a coil of wire whenever you change the environment of coil-magnetic field system. That means that if you change the magnetic field that it sits in over time or move or otherwise distort the coil inside a static ( or changing ) magnetic field you will induce an electro-magnetic force inside the coil of wire and make current run through it. So the EMF is defined as $e_{ind}=-\frac {d\phi} {dt}$ That sums it up. Now the bug that's biting me:

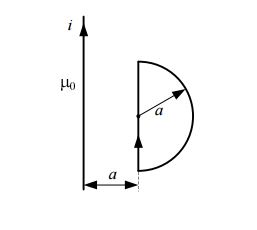
The image shows a system of a very long (considered infinite) straight wire with current through it and a half-circe wire shape. The current through the straight part is given as $i(t)=I_a\sin {\omega t}$ and the problem asks for a EMF induced inside a half-circle wire. For purposes of simplification I am to ignore the self-induction caused by the current that is induced in that same system.

Now I will guide you through the process I took to achieve my answer:

From the given current direction and the shape of the magnetic field lines from the Ampere's law i got that $B_{wire}=\frac{\mu_0i(t)}{2\pi r}$ and the direction is of that into the the drawing plane. By the definition of the induced EMF $e_{ind}=-\frac {d\phi} {dt}$ I need the flux through the surface.

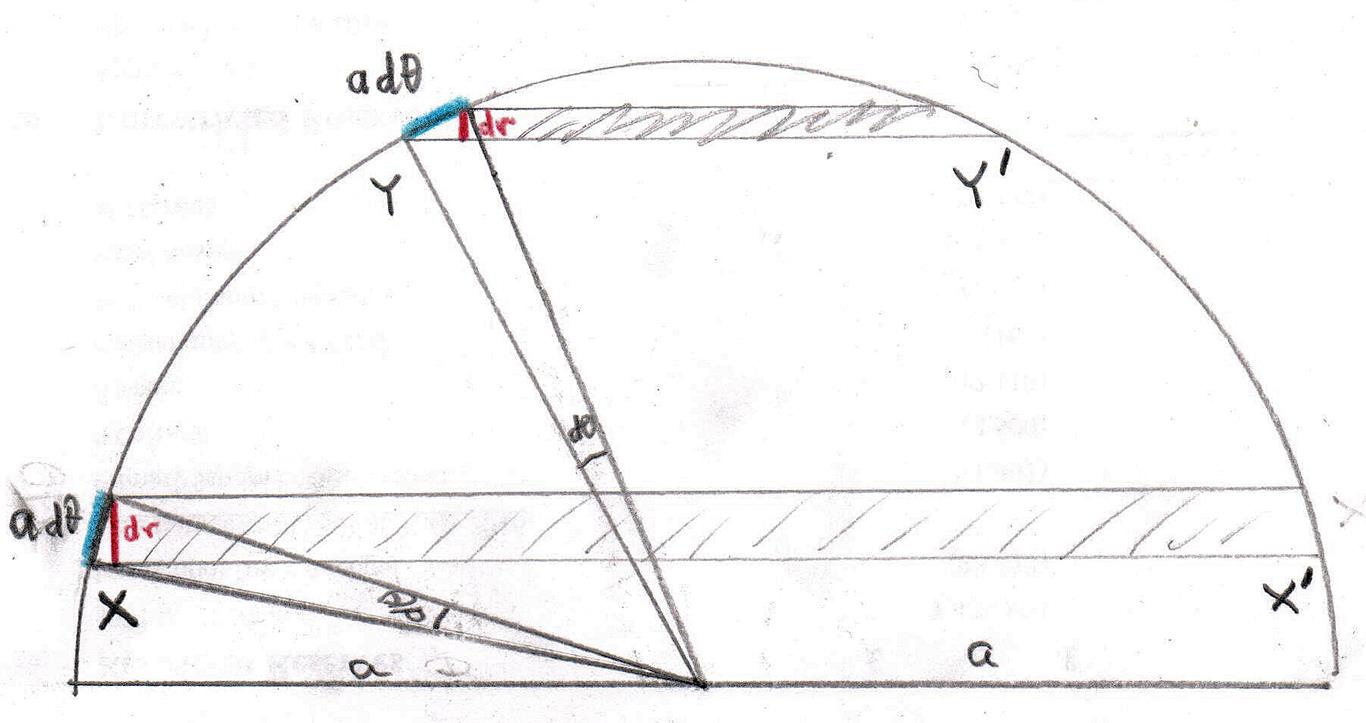
$\int \vec B \, d\vec S$, here is a drawing of my work.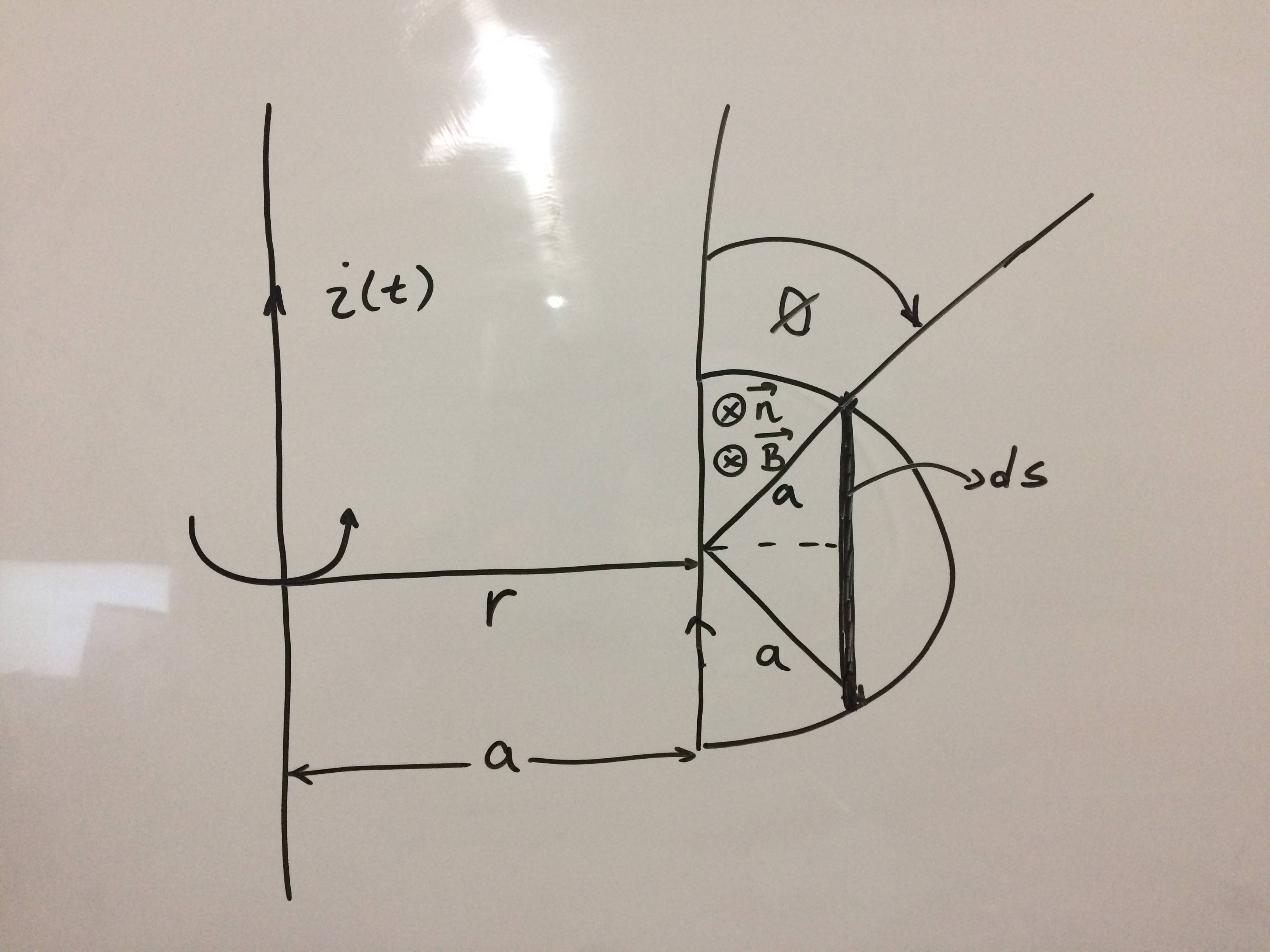

From here I have a disagreement.

It was stated in the notes I got from my friend that the elementary surface of the system is $dS=2a \cos \theta dr$ where $r=a +a \sin \theta$ and $dr=a \cos \theta d \theta$ but my conclusion would have first lead me to say that $dS=2a \cos \theta a d \theta$. Could you help me understand what it is that I got wrong and that I missed in my reasoning.

Also it would be really helpful to comment on my mistakes that were made while posting this question as it's my first post and don't yet know what is not allowed to ask or do.

Thanks :)

Answer 1

You are not the first to make such an error which I have illustrated in the diagram below.

You are trying to find the width $dr$ (red in the diagram) of the strips which you have told are $a \cos \theta \,d\theta$.

What you have done is said that the arc length $a\,d\theta$ is the width of such a strip (blue in my diagram).

For a strip at small angle $\theta$ $XX'$ the approximation is not too bad.

However as the angle $\theta$ gets towards $\frac \pi 2$, strip $YY'$ you can see that there is a very large error between the length of the blue line $a\,d\theta$ and the red line $dr$.