2
$\begingroup$

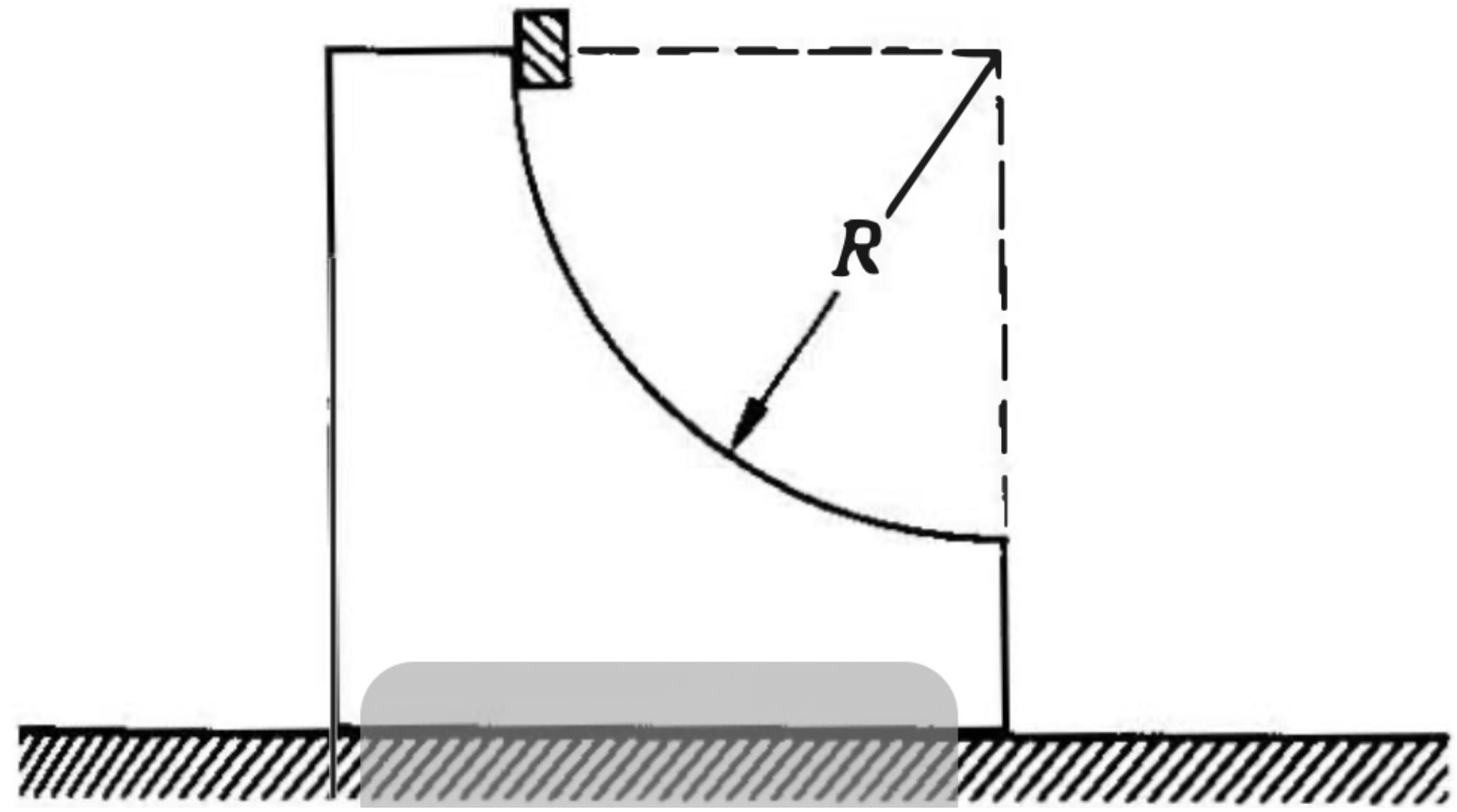
enter image description here

This question is from K&K's intro book on mechanics. The larger block with the quarter circle missing has mass $M$ and the smaller block has mass $m$. The goal is to find the speed $v$ of the smaller block as it leaves the larger block. There is no friction anywhere. The tricky part here is that there is a recoil of the larger block due to the normal force from the smaller block. I am lost as to how to get to the solution.

Resources online give this equation: $$mgR=\frac{1}{2}mv^2+\frac{1}{2}MV^2$$ where $V$ is the speed of the larger block after the smaller block loses contact. I do not see where this equation comes from. It seems to suggest that the mechanical energy of the system is conserved.

I understand that in the frame of the small block, we have that $mgR=\frac{1}{2}mv^2$ But if $$mgR=\frac{1}{2}mv^2+\frac{1}{2}MV^2$$ is also true then $$\frac{1}{2}MV^2=0$$ That is, that the larger block garnered no speed due to the smaller block; which certainly is not the case.

My guess as to why $mgR=\frac{1}{2}mv^2$ is invalid is, since the larger block recoiled, the smaller blocks displacement vector was not truly perpendicular to the normal force. Thus the normal force (nonconservative?) did some work on the small block.

My main question is in the title but it is mostly this: How do we know $$mgR=\frac{1}{2}mv^2+\frac{1}{2}MV^2$$ is true in this case?

I appreciate the help.

Improve this question
$\endgroup$
7
  • 2
    $\begingroup$ The equation for the small mass is wrong, because you are ignoring the work made by the normal force. you need to consider the conservation of mechanical energy for the entire system, and if so you can ignore the normal force as an internal force. $\endgroup$
    – Pato Galmarini
    Commented Mar 22 at 20:48
  • 1
    $\begingroup$ @PatoGalmarini normal forces between the big and small block are internal forces to the system, but this does not imply we can ignore them; they can and do work in this case, most visibly on the larger block. The reason we do not need to consider these internal forces when finding the final velocities is that we can find the solution just from the conservation laws, without careful consideration of the internal forces. $\endgroup$
    – Ján Lalinský
    Commented Mar 22 at 23:54
  • $\begingroup$ @PatoGalmarini But you're right that in the frame of the small block, the equation $mgR=\frac{1}{2}mv^2$ is wrong. This is because in that frame $v=0$. $\endgroup$
    – Ján Lalinský
    Commented Mar 22 at 23:57
  • 1
    $\begingroup$ @JánLalinský yes, that is what I meant, conservation of mechanical energy takes that into account implicitly. I guess I was not that clear. Also, by "frame of the small block" I assumed "I analyze teh block in isolation", otherwise, as you stated, v=0 $\endgroup$
    – Pato Galmarini
    Commented Mar 23 at 0:09
  • 1
    $\begingroup$ Voting to reopen. Obviously a conceptual question rather than a "do my homework for me" question. $\endgroup$
    – gandalf61
    Commented Mar 25 at 9:41

1 Answer 1

Reset to default
2
$\begingroup$

The work energy theorem is essentially a statement that energy is conserved whenever there are no non-conservative external forces acting on a system. This is so because in order for work to be done on an object, there must be a force on the object as the definition of work is: $$W=\int_1^2\vec F\cdot d\vec s.$$If one of the forces in question is non-conservative then it will do work and the system will loose energy by an amount in accordance with the above definition. So if we take as the system in the problem, the combined small and large blocks, then there are no external dissipative forces acting on the system. All of the forces we can draw on a diagram are internal to the system except for the external force of gravity. Thus, we are able to conclude that energy is conserved for the system. Since the initial configuration of the system is such that all of the system's energy is tied up in the gravitational potential energy of the smaller block and the final configuration is such that the small block has fallen by a height of $R$ and both blocks are set in motion, then if the total energy is constant, the gravitational energy of the smaller block has been converted into the kinetic energy of the small and large block system: $$mgR={1\over 2}mv^2+{1\over 2}MV^2.$$

Improve this answer
$\endgroup$
8
  • $\begingroup$ No external net forces yes? Gravity is certainly acting on the system although not doing any work. Thank you for clarification either way. $\endgroup$
    – Chris Christopherson
    Commented Mar 22 at 21:27
  • 2
    $\begingroup$ @ChrisChristopherson gravity is certainly doing work, where do you think mgR comes from $\endgroup$
    – Pato Galmarini
    Commented Mar 22 at 21:30
  • $\begingroup$ Yes you are right, I meant that the only nonconservative forces acting on the system are internal and thus the total mechanical energy of the system is conserved. $\endgroup$
    – Chris Christopherson
    Commented Mar 22 at 21:32
  • 1
    $\begingroup$ @ChrisChristopherson there are no non-conservative forces. If there was friction, that would be a non-conservative force, and it would spoil the conservation of mechanical energy. It does not matter whether the friction is internal or external, it affects mechanical energy conservation. $\endgroup$
    – Ján Lalinský
    Commented Mar 22 at 23:59
  • 1
    $\begingroup$ @AlbertusMagnus the statement about energy conservation is not valid in mechanics in general. Consider a subsystem of particles in Newtonian gravitational system of many particles. Only conservative forces act there, but energy of the subsystem (howsoever we define it, e.g. as kinetic plus potential energy of any subsystem) is not conserved, because it exchanges energy with the rest of the system. $\endgroup$
    – Ján Lalinský
    Commented Mar 23 at 0:10

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.