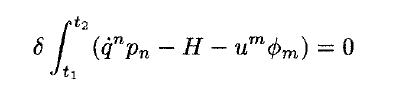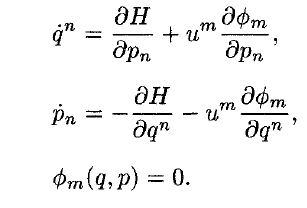Regarding varying the coefficients of constraints in the pre-extended Hamiltonian in Dirac method

Question

consider the following variational principle:

when we vary $p$ and $q$ independently to find the equations of motion, why aren't we explicitly varying the Coeff $u$ which are clearly functions of $p$ and $q$? the authors also either didn't vary $u$ and got the EOM (or) varied $u$ but neglected it since $\phi_m$ are ultimately zero on shell:

so, my question is which one of the above they did and why?

References:

1. M. Henneaux & C. Teitelboim, Quantization of Gauge Systems, 1994; subsection 1.1.4: eqs. (1.12) + (1.13).

Answer 1

The short answer is that at this point in Ref. 1 $u^m$ are new independent variables; not functions of $q$ and $p$. Equivalently, $u^m$ are Lagrange undetermined multipliers.

It is possibly helpful to mention that a field redefinition $u^m\to u^m +U^m(q,p)$ is allowed.

References:

1. M. Henneaux & C. Teitelboim, Quantization of Gauge Systems, 1994; subsection 1.1.4: eqs. (1.12) + (1.13).

2. P.A.M. Dirac, Lectures on QM, 1964; eqs. (1-7)+ (1-8).