1
$\begingroup$

Please clear up this confusion for me:

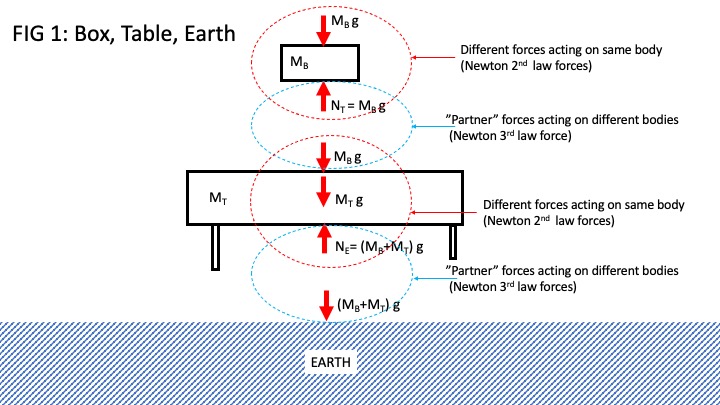
I just watched the video on khan academy @7:30 where the guy explains newton's 3rd law. He explains that for a box on a table, the forces equal out so it's at rest. I also understand that $F=ma$ so an object with less mass may have more acceleration for an equal force.

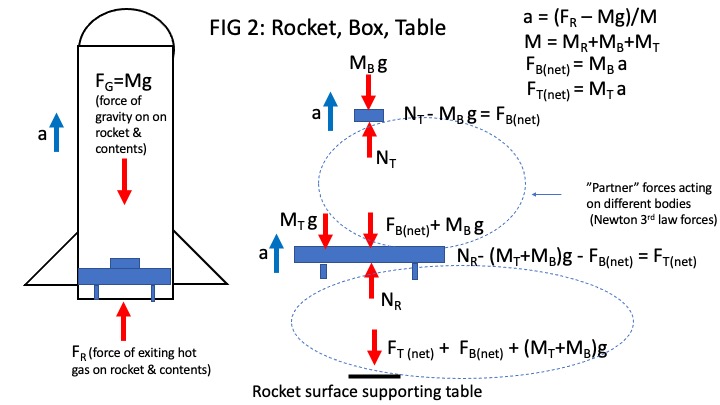
But then he said if you put the table on a rocket ship, the force of gravity is not a partner force to the force moving upward. I'm having a very hard time reconcilling the terminology of what's happening here because I can calculate the force of objects moving sideways along the ground, but am getting lost somewhere in how gravity is not a partner force to the force on a body moving upward.

I'm also confused about a force "pushing down" but at the same time that means a force upward? So then is the force down or up? I know there is an equal and opposite force somewhere in this mess but also that something has to be exceeding gravity or the rocket wouldn't be able to escape?

I'm looking at other stack threads and they're saying it has something to do with different objects relative to each other but that's even more confusing.

Improve this question
$\endgroup$

3 Answers 3

Reset to default
1
$\begingroup$

Newton 3rd law forces, what your link refers to as "partner forces", are equal and opposite forces that act on different objects. The motion of an object is due to the net force acting on that object, per Newton's 2nd law. It is sometimes difficult to distinguish these forces without the aid of free body diagrams.

FIG 1 below shows the forces acting on the box, table and Earth for the "stationary" system. The Newton 3rd law pairs are circled in blue. The forces responsible for the motion of the box and table are circled in red.

Note that the normal reaction force of the table acting up on the box is simply the force of gravity acting down on the box. The net force acting up on the block is the normal reaction force of the table minus the downward force of gravity of the box, for a net force of zero. The same applies to the table except that the force acting down on the table is the sum of the gravitational forces acting downward on the box and table.

FIG 2 shows the rocket with the table and box as its only contents. I didn't actually view the link, but when its says "the force of gravity is not a partner force to the force moving upward" what it probable means is the upward force on the box is no longer simply the force of gravity acting down on the box as in FIG 1. As shown in FIG 2 the upward reaction force of the table acting on the box minus the force of gravity acting downward on the box must equal the net upward force on the box responsible for its acceleration.

Hope this helps.

enter image description here

enter image description here

Improve this answer
$\endgroup$
1
  • $\begingroup$ Good figures! +1 That is very helpful $\endgroup$
    – Dale
    Commented Mar 4 at 22:01
1
$\begingroup$

if you put the table on a rocket ship, the force of gravity is not a partner force to the force moving upward

The force of gravity is not a partner force to the normal force regardless of the situation. Partner forces are always of the same type.

There is a contact force between the box and the table. The partner of the contact force from the table pushing up on the box is the contact force from the box pushing down on the table.

There is a gravitational force between the box and the earth. The partner of the gravitational force from the earth pulling down on the box is the gravitational force from the box pulling up on the earth.

So to figure out a partner force, first identify the kind of force it is and the two objects involved. There will be one force of that kind on each object and they will be equal and opposite.

I know there is an equal and opposite force somewhere in this mess but also that something has to be exceeding gravity or the rocket wouldn't be able to escape?

Consider the table to be part of the rocket.

On the rocket there are three forces: a gravitational force from the earth pulling down, thrust from the exhaust pushing up, and a contact force from the box pushing down. The thrust is greater than the sum of the gravitational force and the contact force, so there is a net force which produces acceleration.

On the box there are two forces: a gravitational force from the earth pulling down, and the contact force from the table pushing up. The contact force from the table pushing up on the box is the partner force of the contact force from the box pushing down on the table. They are therefore equal and opposite. The contact force on the box is greater than the gravitational force, so there is a net force which produces the same acceleration as the rocket.

Improve this answer
$\endgroup$
7
  • $\begingroup$ So my understanding of what you just said looks like this: imgur.com/a/5d2MQld But if we then consider how a rocket lifts off, or perhaps a balloon losing its air, how do the forces act? It's clearly not at rest. Where are the equal forces in that? $\endgroup$
    – skybee
    Commented Mar 4 at 2:20
  • $\begingroup$ Comments are not for follow up questions. If you need a back and forth discussion then you should go to a discussion forum like physicsforums.com otherwise you can post new questions here as separate questions. Be sure to check for duplicates $\endgroup$
    – Dale
    Commented Mar 4 at 2:24
  • $\begingroup$ I'm not supposed to ask for clarification? I can go somewhere else I'd just like to understand. $\endgroup$
    – skybee
    Commented Mar 4 at 2:32
  • $\begingroup$ Correct, your question is supposed to be clear, self contained, and complete. It shouldn’t need any back and forth clarification. Just a straight question and answer. I don’t think that format is actually what you need $\endgroup$
    – Dale
    Commented Mar 4 at 2:46
  • $\begingroup$ If I knew how to make my question that simple then I would have known how to answer it in the first place. Not sure why anyone would ask questions on this site if that's the etiquette here. $\endgroup$
    – skybee
    Commented Mar 4 at 2:49
0
$\begingroup$

To understand this, you have to be careful of what object each force is acting on and what object is exerting the force. Partner forces act on different objects. If I have a force exerted by object A on object B, the 3rd law partner force is the force exerted by object B on object A.

The table pushing up on the box is not a partner force to the earth's gravitational pull downwards on the box. The partner force to the Earth's downward pull on the box is the box's upward gravitational pull on the Earth. Since they are not partner forces, the table's upward force on the box does not necessarily always equal the earth's downward pull on the box.

You can only tell that the forces on the box are equal by noticing that the acceleration of the box is zero. It doesn't have to always be zero, but if you notice it is zero then you can deduce that the forces are equal.

Nothing changes about which forces are partner forces when the system accelerates upward. What changes is the fact that, now, the table's upward force on the box is greater than the earth's downward force on the box. Again, they are not partner forces (they are exerted on the same object) so they don't have to be equal.

As for "is the force down or up?", you say "the" as if there is only one force. The 3rd law deals with pairs of forces. One force is up, one is down. For example, when a ball falls, the Earth pulls down on the ball (force 1) and the ball pulls up on the Earth (force 2).

Again, the most helpful thing to remember is that if I have a force exerted by object A on object B, the 3rd law equal and opposite partner force is the force exerted by object B on object A.

Improve this answer
$\endgroup$
5
  • $\begingroup$ "now, the table's upward force on the box is greater than the earth's downward force on the box." I don't understand how the forces work when in motion. If the partner forces are equal, how do the partner forces between the box and the table being greater than the forces between the box and the ground produce lift? $\endgroup$
    – skybee
    Commented Mar 4 at 2:46
  • $\begingroup$ I think you are just confusing which forces are partner forces. Consider just the box. The only forces on it are the upward force from the table and the downward force from gravity. Those are not partner forces with each other, so they don't have to be equal. In the case where the box is accelerating upwards, the upward force is greater than the downward force. Whenever something accelerates in a particular direction, there must be a net force in that direction. $\endgroup$
    – toomanyfeet
    Commented Mar 4 at 3:15
  • $\begingroup$ If there is a set of partner forces between the box and ground, and another set between the box and table, how are the only forces on the box the upward table and downward gravity? Where do the partner forces go? I'm imagining it like this (imgur.com/a/tDGMCbG), the upward force changes to overcome gravity and its partner changes equally, and the downward force is equal with its partner, but does not change. $\endgroup$
    – skybee
    Commented Mar 4 at 5:43
  • $\begingroup$ The partner forces to the forces acting on the box are not also acting on the box. The only forces acting on the box are the downward gravitational force and the upward table force. The partner force to the gravitational force on the box is exerted on the earth, not the box. The partner force to the upward table force is exerted on the table, not the box. That is where they go. Since they are not exerted on the box, they need not be considered when examining the motion of the box. Only two of the arrows in the picture are actually acting on the box. $\endgroup$
    – toomanyfeet
    Commented Mar 4 at 12:43
  • $\begingroup$ @skybee "If there is a set of partner forces between the box and ground, and another set between the box and table, how are the only forces on the box the upward table and downward gravity?" There are no partner forces between the box and ground. There are only partner forces between the box and the table and between the table and ground. See FIG 1 of my answer. $\endgroup$
    – Bob D
    Commented Mar 4 at 21:41

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.