Understanding the symmetry factors in diagrammatic expansion

Question

Consider the $\phi^4$ scalar field theory. $$ \mathcal{L} = \frac{1}{2} (\partial_\mu \phi)(\partial^\mu \phi) - \frac{1}{2} m^2 \phi^2 - \frac{\lambda}{4!} \phi^4 $$

with the partition function, $$ Z[J] = \int [d\phi] \exp \left( iS[\phi] + i \int d^4 x J(x) \phi(x) \right) $$

When considering diagrammatic expansion for $\mathcal{O}(J^4\lambda^0)$, we have a diagram like

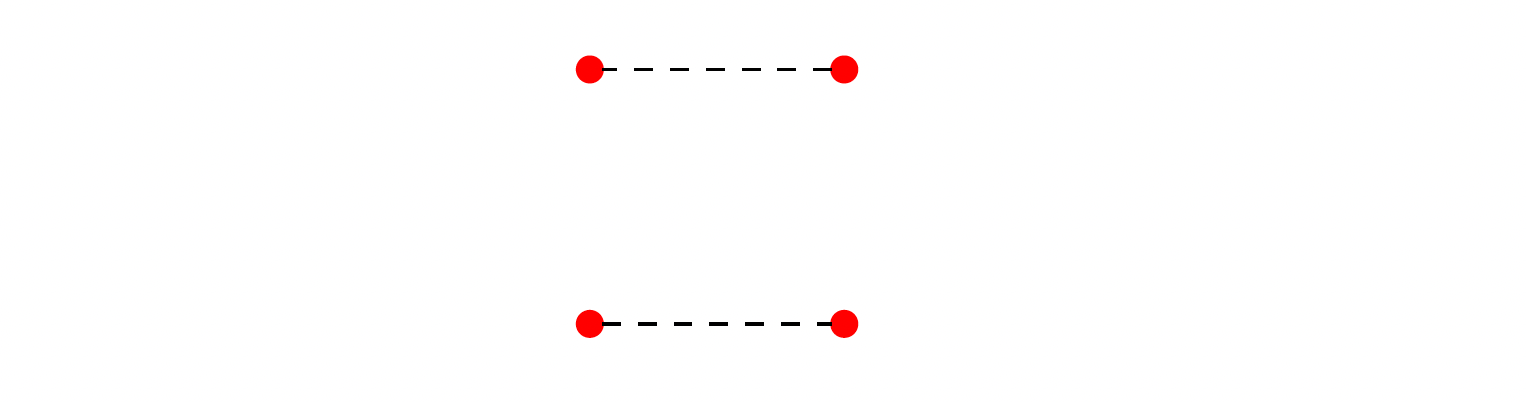
Where each red dot represents an insertion of $J$. My question is why this diagram has a symmetry factor of 8? If we just have one piece of this, the symmetry factor is 2, which makes sense to me as there are two ways to label the two dots. With two pieces, why it doesn't go like $2\times2=4$, or $4! = 24$? Is it because we have an additional horizontal/vertical axis of symmetry?

Also, what are the relations between the symmetry factor of an $n$-point function (which would be $1$ here, without the four dots), and the symmetry factor of diagrammatic expansions? Does it have anything to do with connected diagrams?

Answer 1

Let $E=\{1,2,3,4\}$ be a set of labels/names for the four red dots. Say 1 is the top left one, 2 is the top right, 3 is the bottom left, and 4 is the bottom right.

If one is computing the partition function (vacuum graphs with no labeled external lines) in the presence of sources $J$, then the symmetry factor is the number of permutations of $E$ which preserve the set partition $\{\{1,2\},\{3,4\}\}$. You can exchange 1 and 2, exchange 3 and 4 and also exchange the block $\{1,2\}$ with $\{3,4\}$. The number of such permutations is $2^3=8$.

If one is computing a 4-point function, the red dots are no longer considered as internal vertices of degree/valence one. The four legs are labeled and the permutations to be counted must fix the labels of the external legs, i.e., all the elements of $E$. So the permutation is the identity and the symmetry factor is just 1.