1
$\begingroup$

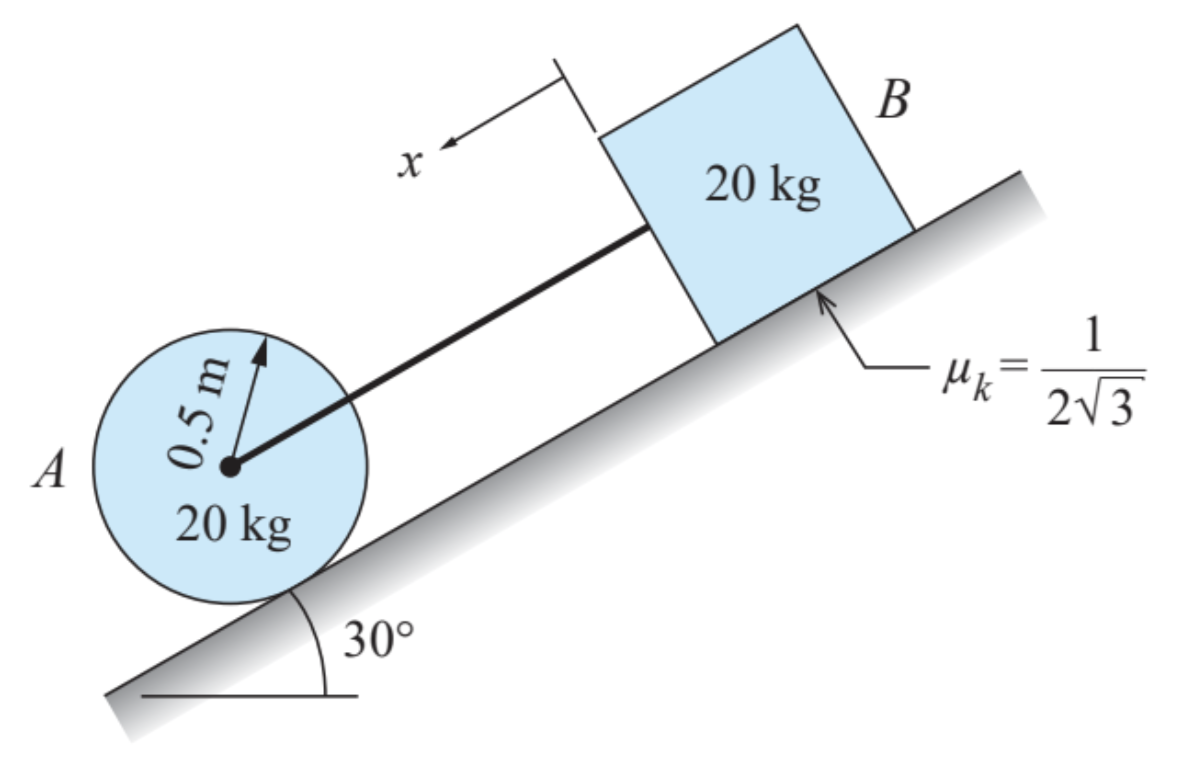
enter image description here

In the exercise described by the attached picture, in which the cylinder A rolls without sliding, I was asked to find what the distance traveled by the system would be when the blocks speed was equal to 3 m/s. My approach to solving it was applying Newtons second law for each object, taking into account the mass and linear acceleration of both objects are equal. This yielded the following equations:

$$F_rr = I\alpha$$ $$mg\sin\theta-T-F_r = ma$$ $$mg\sin\theta+T-F_r = ma$$ $$r\alpha = a$$

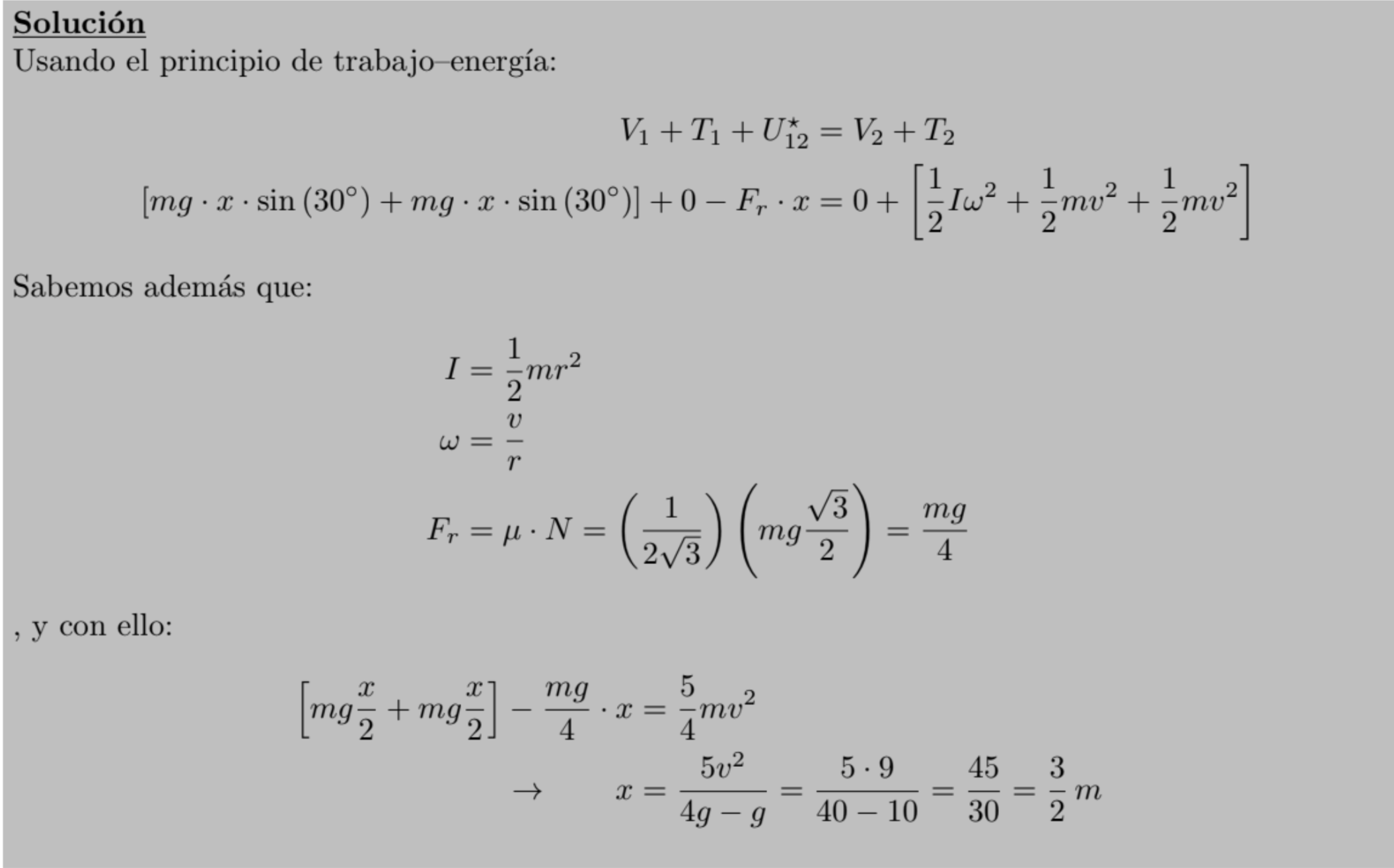
The first two equations correspond to the cylinder, the third one to the block and the forth one to the rolling condition. I have chosen the coordinate system to be positive in the direction the system moves. Solving the system I obtained $a = 4/3 m/s^2$, and using that to calculate distance traveled when $v=3 m/s$ gives an incorrect answer. The correct way to solve the problem, according to the answer, is as follows:

enter image description here

Although it's in Spanish, it is clear the work-energy principle was applied, and distance traveled was calculated using the work friction did on the system. What I don't understand about the answer is, shouldn't work be the sum of the work friction did on the block and the work friction did on the cylinder? Why isn't it multiplied by 2, and why is my initial answer wrong?

Improve this question
$\endgroup$

3 Answers 3

Reset to default
0
$\begingroup$

enter image description here

from the FBD you obtain

$$ I_Z\,\alpha=F_r\,r\\ m\,a_Z=m\,g\sin(\phi)+T-F_r\\ \alpha\,r=a_Z\\ m\,a_K=-T+m\,g\,\sin(\phi)-m\,g\,\cos(\phi)\,\mu_K\\ a_Z=a_K$$

those are 5 equations for the unknowns $~\alpha~,a_Z~,a_K~,T~,F_r$

from here $$v=a_K\,t\quad ,x=\frac 12 a_K\,t^2$$

the solution for x is:

$$x=\frac 12\,{\frac { \left( 2\,\,m{\,r}^{2}+I_{{Z}} \right) {v}^{2}}{\,g\,m{\,r}^ {2} \left( 2\,\sin \left( \phi \right) -\cos \left( \phi \right) \mu_{ {K}} \right) }}\quad,I_z=\frac 12 \,m\,r^2\\ x=\frac 54\frac{v^2}{g\,(2\sin(\phi)-\cos(\phi)\,\mu_k)}=\frac 32~[m]$$

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ So I guess the key takeaway is that rolling friction is separate from regular friction, and should be treated as an unknown. Thank you! $\endgroup$
    – Dollar Store Richard
    Commented Dec 10, 2023 at 19:24
  • $\begingroup$ From the rolling condition, you obtain constraint force, but this is not the rolling friction, analog from the acceleration condition, you obtain the tension force T $\endgroup$
    – Eli
    Commented Dec 10, 2023 at 20:22
  • $\begingroup$ Wait, if it isn't rolling friction then what is the constraint force supposed to represent? $\endgroup$
    – Dollar Store Richard
    Commented Dec 10, 2023 at 20:55
  • 1
    $\begingroup$ en.wikipedia.org/wiki/Rolling_resistance $\endgroup$
    – Eli
    Commented Dec 10, 2023 at 22:19
0
$\begingroup$

Friction between the cylinder and the ground does zero net work in case of pure rolling, since friction force acts on points with zero relative velocity.

Improve this answer
$\endgroup$
1
0
$\begingroup$

The work equation does not include the work done by friction on the cylinder because it is pure rolling, which means that the contact points of the cylinder and wedge relatively stationary. In cases like this the contact points keep changing and while friction applies a force on it it does not diplace it, so it does not do any work on the cylinder.
You can solve it without WEP. Here's how:
Equation of block:
$mgsinθ + T - μN = ma $(Here friction is limiting because we know that the block is moving)
However we don't know frictional force acting on the cylinder. Since, it is pure rolling, it is rotating about the contact points.
Writing Torque Equation;
$3/2 mr²α = mgsinθr - Tr$ (Inertia about the bottom is mr²/2 + mr²)
$a = rα$
On solving, we get $a = 3m/s²$
$v² = 2as$
$9 = 6s$
$s = 3/2 m$

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks for your answer! I'm still a little confused as to when you are supposed to change the pivot point to solve rolling problems, and the implications of doing so. Does it change anything aside from Newton's second law (and the respective torques applied)? And one last thing, wouldn't the solution involving the WEP include the work rolling friction did (which another answer included in their solution)? $\endgroup$
    – Dollar Store Richard
    Commented Dec 10, 2023 at 19:21
  • 1
    $\begingroup$ Whenever a body is rolling such that its contact point is not slipping, then work done by friction on the body is zero because while there is frictional force being exerted at the contact points, they are at rest at any point of time and so by definition friction does not do any work. I chose the axis at the bottom one because frictional force passes through it and so torque of frictional force about it is zero. So, we would not need to find frictional force. Comment for clarifications. $\endgroup$
    – Piyush Lath
    Commented Dec 10, 2023 at 19:34

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.