There are a variety of cloud wave patterns, including radiatus, undulatus, and gravity wave clouds. Their causes are not mysterious, but fluid mechanics is rarely simple. When air rises and falls in a pattern, clouds form at the high points if the air reaches the lifted condensation level. The spatial period of the clouds does not in general depend just on wind speed and height, so they cannot easily be used to estimate the wind speed at height.
Radiatus clouds (also known as "cloud streets" or "horizontal convective rolls") are lines of thermal-updraft-top clouds which form parallel to the wind direction. The wind lines up the convective cells to form horizontal convective rolls as shown in this image from Wikipedia:
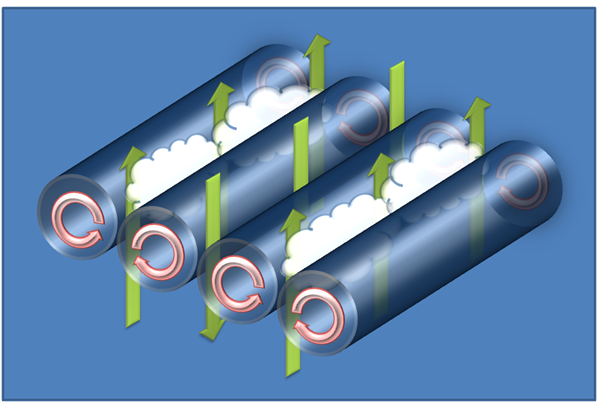
The clouds form if the rising air reaches the lifted condensation level before the updrafts are stopped by an inversion or stable layer. The air is (relatively) clear above the downdrafts. If the convection rolls were perfectly circular, the cloud row spacing would be twice the height of the inversion/stable layer.
Mathematically, there are many wavelength solutions to convection, but the wavelength that dominates is the fastest growing one. In the Boussinesq approximation, which is reasonably valid here, this turns out to have a wavelength of $2\sqrt{2}\sim 3$ times the height of the convecting layer, i.e. slightly flattened. (See, for example, Eq. 21 of Kuettner (1971) "Cloud bands in the earth's atmosphere: Observations and Theory".)
For typical cumulus cloud heights of $\sim 2$ km, we expect typical spacings of about $6$ km.
Wave, lee, or mountain clouds are lines of clouds downwind of an obstacle (such as a mountain range). The lines are parallel to the wind direction. These are buoyancy waves where wind pushes denser air over an obstacle (e.g. a mountain range) and it ends up above less dense air on the other side. This dense air starts to fall but it overshoots into even higher density air at lower altitude, which forces it back up, and the air ends up bouncing up and down until the oscillations die out. If the vertical temperature profile of the air then is known, it is possible to estimate the vertical buoyancy angular frequency
$$N=\sqrt{\frac{g}{\theta}\frac{d\theta}{dz}}$$
where $g$ is the local acceleration due to gravity, $\theta$ is the atmosphere's potential temperature, and $z$ is the height. Since this is the vertical oscillation angular frequency, if the wind speed is $v$, we expect the the spacing between rows to be $\lambda\sim 2\pi v/N$. For example, for $\theta \sim 273$ K, a temperature lapse rate of $\frac{d\theta}{dz}\sim 10$ K/km, and a wind speed of $50$ km/h, we'd expect $\lambda\sim 5$ km, which is consistent with typically observed spacings of $2-8$ km.
Undulatus clouds (or "billow clouds") are usually just the bottom view of waves generated by Kelvin–Helmholtz instabilities which form perpendicular to the wind direction when the wind shear between two layers of air is large enough to overcome the buoyancy forces keeping the layers separate. This image from Wikipedia show how the Kelvin-Helmholtz instability starts.

Such waves are expected when the Richardson number ($\mathrm{Ri}$) is smaller than about 1/4:
$$\mathrm{Ri} = \frac{\text{buoyancy}}{\text{flow shear}} = \frac{g}{\rho} \frac{\partial \rho/\partial z}{(\partial u / \partial z)^2} $$
where $g$ is the local acceleration due to gravity, $\rho$ is the air density, $z$ is vertical height, and $u$ is the air velocity.
The density difference is usually because of a temperature difference, in which case
$$\mathrm{Ri} \approx g \beta \frac{\Delta T/L}{(\Delta V / L)^2} = g \beta L \frac{\Delta T}{(\Delta V)^2}$$
where is $L$ is the thickness of the unstable shear layer over which the temperature $T$ and velocity $V$ are changing and $\beta$ is the coefficient of thermal expansion of air.
Rolls can form in this shear layer, with the the roll spacing expected to be about $3$ times the roll height, i.e. $3L$.
If chemtrail proponents don't accept your explanations that periodic wavey cloud patterns can form naturally, perhaps they might be more willing to believe Fox News: "Crazy Clouds! The wave!". You might also ask them how chemtrails could possibly explain this beautiful NASA image of cloud streets in the Bering Sea. The wind is blowing diagonally from sea ice (upper right) over the warmer sea water in the lower left producing hundreds of parallel stable cloud streets.

You might also note that lee cloud waves have been observed on Mars. But I suspect conspiracists would just argue that both the Mars and Bering Sea images are NASA fakes.
This wind shear process that drives undulatus cloud formation is closely related to how many other common waves are produced by fluids moving parallel to an interface. If someone believes that chemtrails are the only possible explanation for undulatus clouds, then to be consistent they should also not believe that the wind can produce waves the surface of water, or that water can produce underwater beach sand ripples.



