0
$\begingroup$

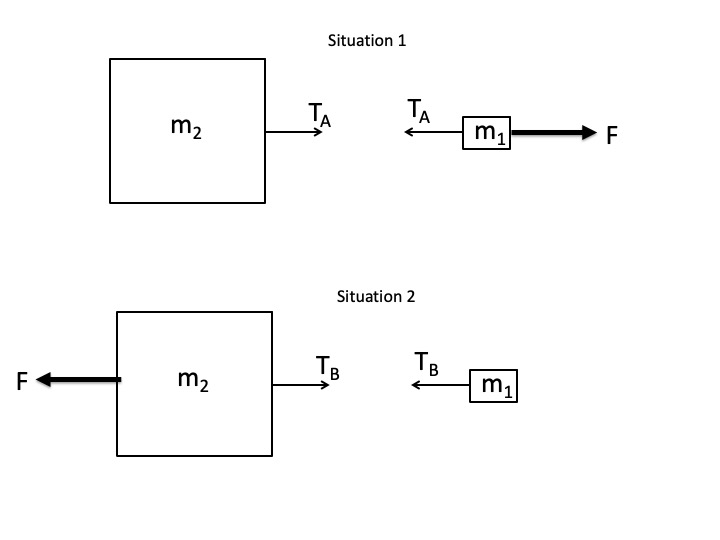
Consider a system of two blocks having masses $m_1$ and $m_2$ lying on smooth floor, where $m_2>m_1.$ They are attached by an ideal inextensible string. There are two different situations $1$ and $2$

  1. Force $F$ is applied To $m_1$ and system accelerates with acceleration $\frac {F}{m_1+m_2}$

  2. Force $F$ is applied To $m_2$ and system accelerates with acceleration $\frac {F}{m_1+m_2}$

Now in case 1, value of tension is $T_{A}=\frac {m_2\cdot F}{m_1+m_2}$, while in case 2,Value of tension $T_{B}=\frac {m_1\cdot F}{m_1+m_2}$.

Clearly $T_{B}<T_{A}$ as $m_2>m_1$.

Why is this happening intuitively?. As in both scenarios, System still accelerates with the same acceleration.

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ Something to help build intuition; consider also a "train" of blocks (say, 3 or more of them), all of the same mass, all connected by an ideal massless string, accelerated as a system by the force $F$ in one direction: what are the tension forces at each connection? $\endgroup$
    – Filip Milovanović
    Commented Nov 6, 2023 at 17:16

2 Answers 2

Reset to default
3
$\begingroup$

Why is this happening intuitively?

Because the tension is only that required to move the trailing mass. If the rope were to break, the first mass would still accelerate since the force is acting on it directly.

Imagine a real car a tiny toy car connected together. If you were to push the real car, the toy car would only need a thread to be pulled behind. Not much tension is required both because the small car is low mass, but also because you're not going to be able to accelerate the big car quickly anyway.

If you were to pull the toy car away, then you would need a strong rope for the real car to accelerate before the string snapped. The rope needs to carry almost the entire force applied to the system.

Improve this answer
$\endgroup$
1
$\begingroup$

Consider the free body diagrams (FBD) below.

As seen in the FBD's, the only force responsible for accelerating the trailing mass in both situations is the tension force in the string.

Given that the trailing mass in situation 1 is greater than the trailing mass in situation 2, in order for both to have the same acceleration the tension force in situation 1 must be greater than the tension force in situation 2, or

$$T_{A}\gt T_B$$

An intuitive example would be a car towing a truck (situation 1) versus a truck towing a car (situation 2). A stronger rope would be needed to tow the truck.

Hope this helps.

enter image description here

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.