Variation of height above the ground and speed

Question

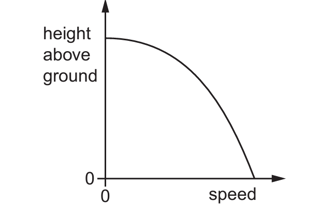
A ball is dropped from rest and falls towards the ground. Air resistance is negligible. What is the graph that shows the variation with speed of the height of the ball above the ground?

Here's my intuition.

$$v^2=u^2+2as$$ $$v^2=2as$$ $$s=\frac{v^2}{2a}$$

Hence $s$ is proportional to $v^2$. The graph must be a quadratic relationship. Hence the graph should be upwards sloping from the origin.

However, this is obviously not the case as there is originally a height above the ground, and therefore there must be a y intercept. The answer scheme provides the following graph.

Why is the curve downwards sloping when I just derived a quadratic relationship as shown above? Have I done something wrong?

Edit: John's explanation is great. However I am still unclear why the parabola slopes downwards rather than upwards.

$$h_0+\frac{v^2}{2a}$$ Seems to be a positive parabola. So why is the graph the other way round? $a$ is inuitively positive too. So I cannot see why this produces a negative parabola shape.

Answer 1

$s$ is not the height above the ground. It is the change in the height. If we define the initial height as $h_0$ and the height some time later as $h$ then:

$$ s = h - h_0 $$

Your equation:

$$ s=\frac{v^2}{2a} $$

is correct, but if you are going to plot height on your graph you need to replace $s$ by $h_0 - h$ to get:

$$ h - h_0 = \frac{v^2}{2a} $$

or you'd probably rearrange it to:

$$ h = h_0 + \frac{v^2}{2a} $$

I am still unclear why the parabola slopes downwards rather than upwards.

Our usual sign convention is that the positive direction for height is upwards. That is, if we take $h = 0$ then heights above the ground are positive and become more positive as we move up, while heights below the ground are negative and become more negative as we move down.

Since velocity is the change in height with time this means upwards velocities are positive and downwards velocities are negative, and therefore that upwards accelerations are positive and downwards accelerations are negative. Specifically the $a$ in your equation is the downwards acceleration due to gravity so the value of $a$ is:

$$ a = -9.81~\textrm{m/s}^2 $$

So your parabola looks like:

$$\begin{align} h &= h_0 + \frac{v^2}{2 \times -9.81} \\ &= h_0 - \frac{v^2}{2 \times 9.81} \end{align}$$