Force not applied on center of mass

Question

I have a question about rigid body motion when a force acts on off-center of mass.

I read the answer to the post force applied not on the center of mass but I'm still confused.

I understood that translational acceleration is same whether force acts on c.o.m or not. Therefore, maybe 2 cases have same linear velocity and trajectory for given same time. And then applied energy will be also same because the trajectory (=distance) is same.

The problem is that the first case has only translational energy and the second case has translational energy which is equal to first case and rotational energy.

Is there something I missing?

Answer 1

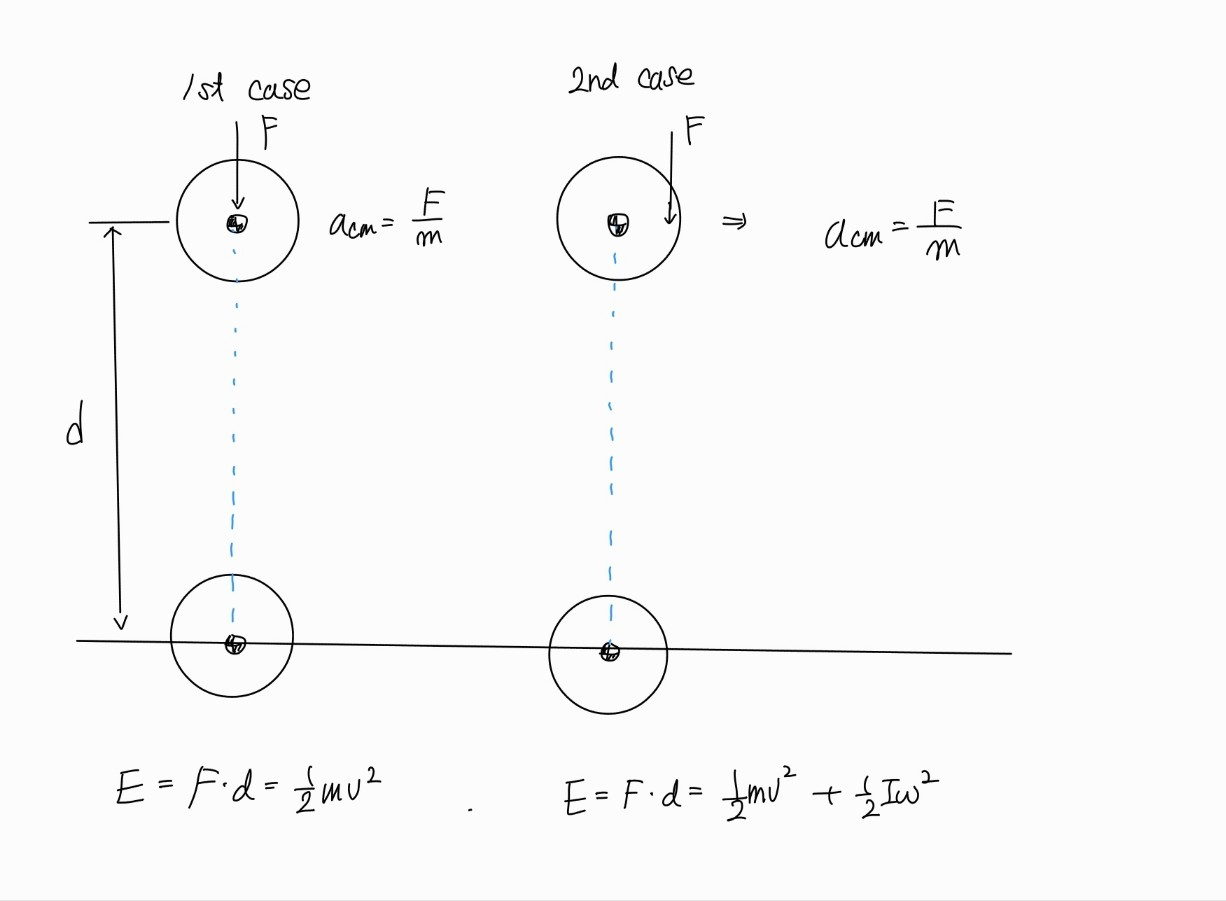
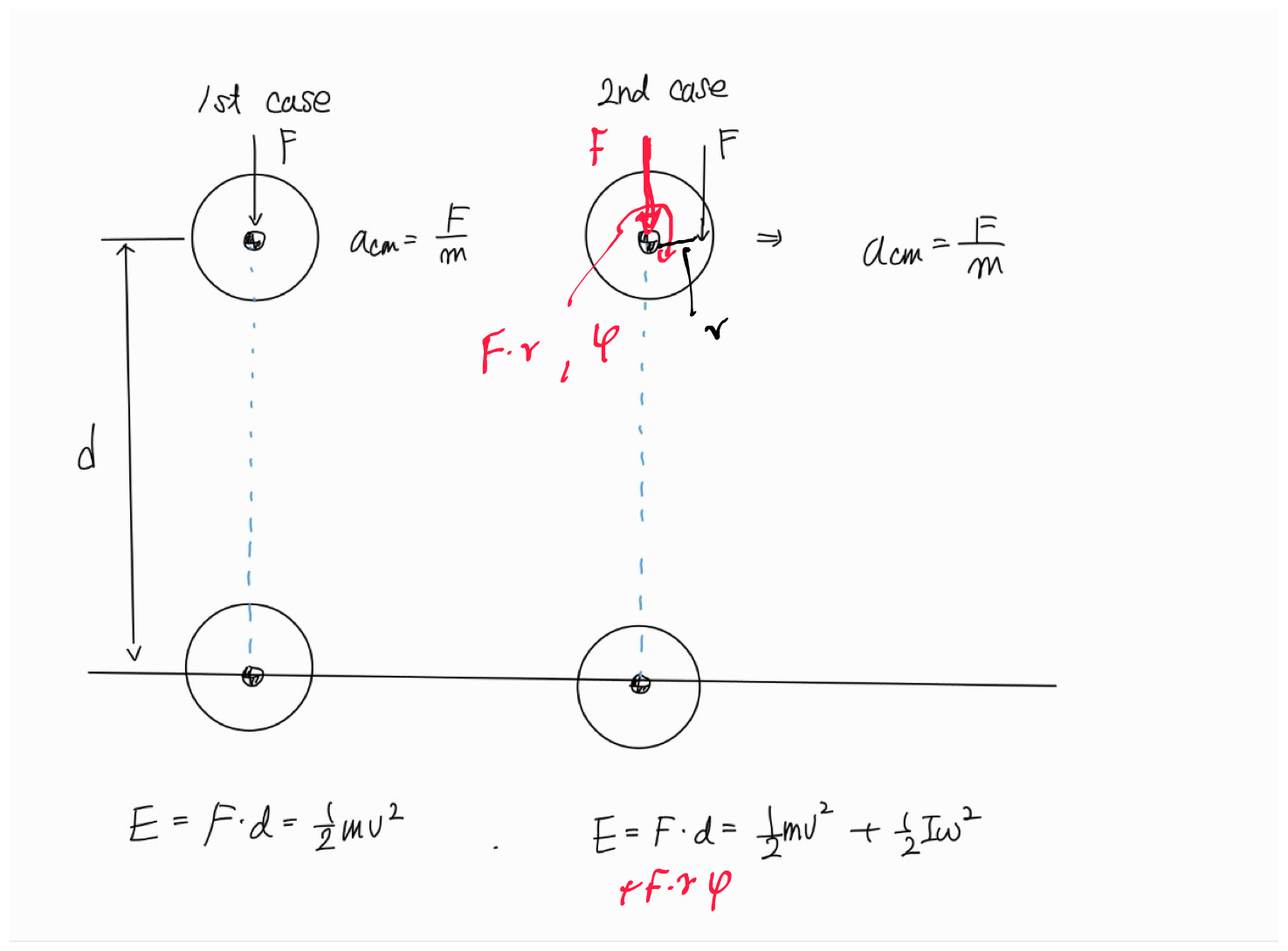
First this may help - Toppling of a cylinder on a block.

Let us consider a different object. Take equal two masses connected together with a long rigid rod of length $l$.

Case 1 - Apply a force $F$ to the center of the rod so that both masses are accelerated equally. After you push for a short distance $d$, you have given the system energy $E = Fd$. You can figure out the velocity from $E = 1/2(2m)v_1^2$

Case 2 - Do it again, but apply the force to one of the masses for a distance $d$. This time that mass accelerates. The other mass is a long way off sideways. The rod pulls a little bit, but that mass accelerates very little. We will approximate its speed as $0$ just to make calculation easier. It will be a little off, but not much. I just want to illustrate the point.

The system is rotating because one mass is moving faster and getting ahead of the other. Again, you have given the system energy $E = Fd$.

One way to understand the energy in this case is to add up the kinetic energies of each mass.

$$E = 1/2mv_2^2 + 1/2m0^2$$

You can calculate that $v_2 = \sqrt 2 v_1$.

Another way to calculate the energy is

$$E = E_{translation} + E_{rotation}$$

where

$$E_{translation} = 1/2 (2m) v_{COM}^2$$

and

$$E_{rotation} = (1/2) I \omega^2$$

$v_{COM}$ is the velocity of the center of the rod. You can verify that

$$v_{COM} = 1/2 v_2$$

You can verify that

$$I = 2 m (l/2)^2$$

and

$$\omega = \frac{v_2/2}{l/2}$$

If you add it all up,

$$E_{translation} + E_{rotation} = 1/4 m v_2^2 \space + \space 1/4 m v_2^2$$

So both ways come out the same.

Also the total energy is the same for case 1 and case 2, even though it is distributed differently among the masses.

You should think about how long it took. In case 1, you pushed $2m$ a distance $d$. In case 2, you pushed $m$ a distance $d$. What does that tell you about momentum?

Answer 2

In the first case, the disk does not turn. That which applies the force does not move along the disk as the disk moves. The second disk rotates as the force pushes. If that which applies the force moves straight down, that object will pass the disk. That which pushes does so along the surface of the disk. That which pushes must move back along the disk to maintain its position. Some of the energy goes into torque against the disk to maintain the position. The relationships $v=r\omega$ and $a=r\alpha$ have to be maintained throughout the motion for that which pushes to keep its location relative to the center of mass. In order to keep pushing forward over the distance shown, the force on the disk must be greater than in the first case.

Answer 3

If the target object is circular or spherical (thin disk or sphere) it won't be rotating in either case, it will just translate as the local vertical at the point of contact will always go through the center (of mass) for a circle/sphere. It will just go in a different direction with a different speed according to the laws of elastic collision. You know this from the case of colliding billiard balls (in this case the ball only rotates because on a surface it can't translate without rotating; in space it would not be rotating).

Answer 4

Work is the product of the force by the distance the force moves, in this case of constant force and one directional displacement.

The question is how exactly the force $F$ in the second case moves. The situation is similar to an yoyo pulled by the string in a horizontal surface without friction. In the first case the force displacement equals the object displacement. But in the second case, the end of the string (the point of application of the force) has to run a bigger distance than the object.

So, while the force is the same, the work is different for the 2 cases, and explains why the kinetic energy is bigger in the second case, when we have rotational kinetic energy, besides the translational one.

Answer 5

Case I

$$E_I=F\,d=\frac m2\,v^2$$ where $~v=\dot d~$

Case II

you obtain a force at the center of mass plus a torque ($~\tau=F\,r~$) that cause the ball to rotate

$$E_{II}=F\,d+F\,r\,\phi=\frac m2\,v^2+\frac I2\,\omega^2$$

where $~\omega=\dot\phi~$