Weird rotational motion of a heavy thick disc

Question

I and my friend noticed a strange behaviour of a rotating disc (or a cylinder) and we don't know how is that happening. This is the video.

Actually what is happening in the video is that the disc wobbles (the rotational axis precesses about the vertical) a bit and then it stops wobbling (the rotational axis coincides with the vertical axis) and again starts to wobble and then stops and this process goes on until the rotation stops.

So can someone here explain me why is that happening?

Edit:-

(1) As per the comment and the answer received I want to add that the disc is a perfectly rigid object (most probably of steel) and one more thing I want to add is that the cylinder as a whole is actually two discs placed one over the other and glued together tightly to avoid any relative motion.

(2) Here's a screenshot showing the bottom of the disc as asked for in the comment:-

Here's the disc from below:-

Edit :-

Here's a video by Vsauce where similar motion can be observed at around 20sec. The smaller top inside does something similar to what I have shown in the video linked earlier.

Answer 1

Not surprisingly, the technical term for this is "wobble". Lets call your disk a top. An you will find that for tops with a point contact you will have precession. In that discussion you will note that they mention when the angular speed of the top decreases to a certain point, you will also get wobble and that wobble is more complicated.

However, I don't think you have a point contact like in the hyperphysics link on precession. Instead I suspect that as you spin you are in contact with the table on the edge of a small ring of metal. The physics of that is more analogous to spinning a coin or a bottle where the point of contact is moving along the edge of the coin or bottle.

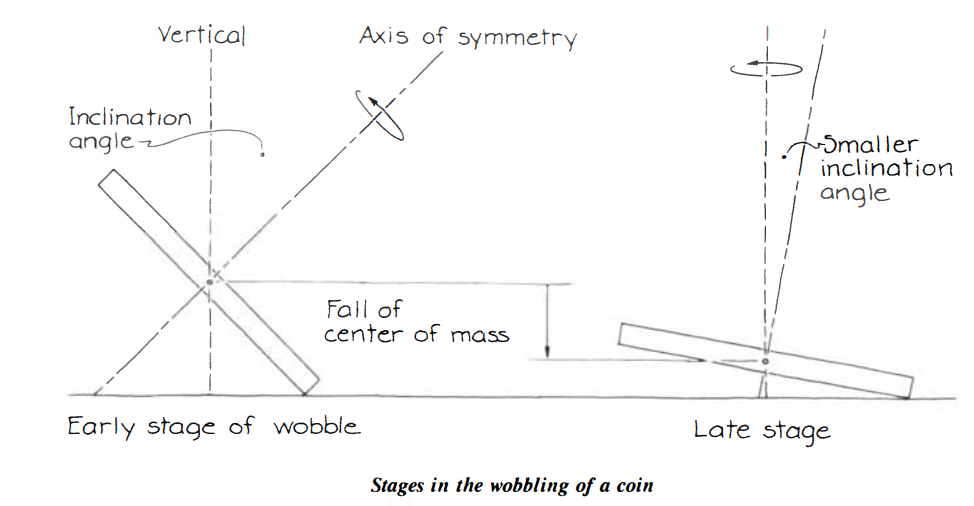
The interesting question you ask is why does it start to wobble (more violently) and then stop wobbling (or at least less violently). With out knowing more about the nature of the contact with with bottom, I think what is happening when it is smoothly spinning it spinning on the edge of the ring and then when it is wobbling more violently that is more like the coin wobbling when it is more flat and it is the contact isn't as nice and predictable. However, unlike the coin you have a lot of angular momentum with the heavy disk, and in that wobbling you transfer some energy such that it starts to spin more on the outer edge of the ring or disk that is in contact with the table. It does that a while and then when it loses enough energy the process repeats.

If the contact is point contact I don't think you would see that periodicity. I am kind of using the coin and its rim as an analogy, You probably have something that is more like a small cylinder, or a small hollow cylinder, and if so how sharp the edge and how uniform that surface is would also make a difference.

If you have a point contact and the spinning mass is unbalanced you will also see both precession and wobble, but that wobble would not be starting and stopping as you seem to see in the video. You would typically see the precession rotate around and the wobble move back an forth.

To work out the mathematics for your special case would take a little work, but there are quite a few papers on the wobble of tops and the wobble of the earth that would be a good starting place.

It is a nice observation and video.

Answer 2

you can see this wobble effect if you obtain the numerical simulation.

the disk has three generalized coordinate (Euler angles) which are

• $~\varphi~$ Rotation about x-axis
• $~\beta~$ Rotation about y-axis
• $~\psi~$ Rotation about the z-axis

Numerical results

ignoring the potential energy ($~g=0~$) and with torque $~\tau_\psi~$ input about the z-axis.

you can see the wobble effect at the results of $~\beta(t)~,$ $~\varphi(t)~$ ans $~\psi(t)$

Remark:

you need the completely nonlinear equations of motion , trying to linearized the equations of motion you don't see the wobble effect.

Answer 3

This appears to be an example of what is called "nutation". This is a wobble in the tilt of a precessing top. I suppose the ideal answer would explain intuitively why this wobble happens, then express it in equations, then solve specifically for the case of a heavy thick disc, in a way that plausibly gave oscillations with the amplitude and frequency seen in the video.

The intuitive explanation here seems reasonable. There are equations here or in this video.