I'll try to address the direct question asked in the title of this question:
"How many cavity round trips typically occur inside laser resonators?"
The cavity finesse roughly captures the answer to this question. No mirror is perfect. If power $P_0$ is incident on a mirror then some percentage $R$ of that power will be reflected, some percentage $T$ will be transmitted, and some percentage $L$ will be scattered (off of surface defects) or absorbed in the mirror material. By conservation of energy we have $1 = R + T + L$.
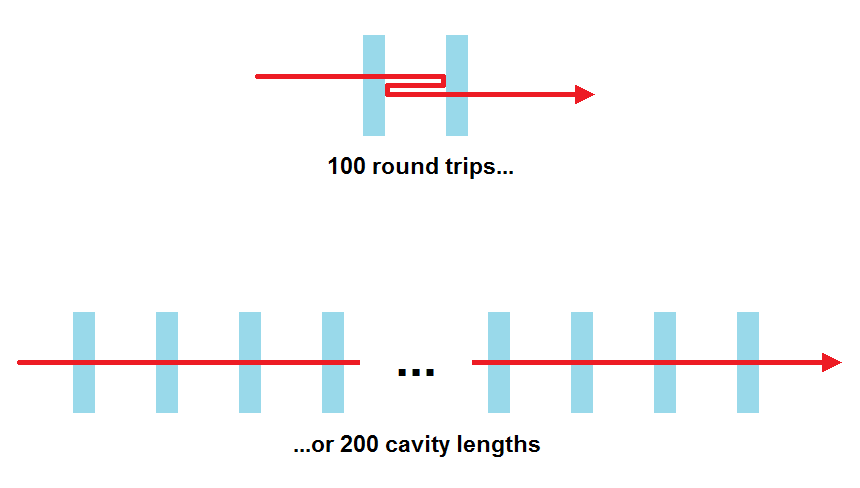
intuitively, if 1% of the light is lost from both mirrors then you'd expect the beam to make approximately 100 round trips before it is pretty much totally out of the cavity. This is basically what the Finesse says. Suppose we have two mirrors with $R_1, L_1, T_1$ and $R_2, L_2, T_2$. The formula for Finesse (which can be approximately though of as "the number of round trips"
$$
\mathcal{F} = \frac{2\pi}{T_1 + T_2 + L_1 + L_2}
$$
Finesse is limited, in practice, by how perfectly we can design mirrors with no loss or transmission. The highest finesse cavities in the world probably reach $\mathcal{F} \approx 10^6$, meaning transmission and losses are at the single part-per-million (ppm) level.
Depending on the specific commercial laser you will probably find finesses anywhere from 100 to 100,000 or more. The finesse will depend on various design criteria, and higher is not always better depending on what you want.
When it comes to lasers, the finesse is related to the longitudinal coherence of the laser. That is, how correlated is output light at one position and time with light at the same position but another time. Or equivalently, how correlated is output light at one position and time with output light at the same time but another position along the propagation direction of the beam?
The free spectral range of a cavity tells us how far apart in frequency space its repeated resonances are. It is given (in cyclic frequency units) by:
$$
f_{FSR} = c/2 L_{cav}
$$
Where $L_{cav}$ is the length of the cavity (not to be confused with mirror losses $L$). The cavity linewidth (in cyclic frequency units) is given by
$$
\nu = f_{FSR}/ \mathcal{F}
$$
$\nu$ is related to the coherence time by $\nu \propto \frac{1}{t_{coh}}$ and the coherence length by $\nu \propto c/l_{coh}$. So we can see increasing the length of the cavity or the finesse of the cavity. So I think there is something to be said along the lines that: the cohereence of light coming out of a cavity is related to the duration of time or length of travel that it spends within the cavity resonator.
This might answer some parts of the question but I'm not sure if it answers more than the Wikipedia page on Fabry-Perot Interferometers.
The question asks a lot of stuff about what I would call "transverse" spatial coherence. And unfortunately it is hard for me to make sense of these parts of the question. The transverse spatial profile of a cavity is related to the curvature and distance between the mirrors. That is, the geometry of the output beam depends on the geometry of the cavity mirrors. Changing the finesse of the cavity (i.e. mirror reflectivity) and thus number of round trips has no effect on the shape of the output beam of light. This is at least true for optical cavities and lasers. In these cases at least one (usually both or all) cavity mirrors are concave to sort of refocus light back into the cavity when it bounces of the mirrors. etalons with two truly planar surfaces are not used as cavity resonators but rather serve other kinds of purposes.
See "Lasers" by Siegman for a much more thorough treatment of all of this.
Response to first comment. Above I've been a little glib about "if $\mathcal{F} = 100$ then the light makes roughly 100 round trips before it's 'gone'". The more precise mathematical statements (more rigorous derivations can be found in the reference) is like this. The field in the cavity decays like $e^{-\pi \nu t}$ and the energy decays like $e^{-2\pi \nu t}$. Focusing on the energy, the $1/e$ time for this decay is
$$
t_{1/e} = \frac{1}{2\pi \nu} = \frac{\mathcal{F}}{2\pi f_{FSR}}
$$
The cavity round trip time is $\tau = 1/ f_{FSR}$ so the $1/e$ number of round trips is
$$
N_{1/e} = t_{1/e}/\tau = \frac{\mathcal{F}}{2\pi} = \frac{1}{T_1 + T_2 + L_1 + L_2}
$$
Which is actually pretty satisfying. The total round trip energy loss probability is $L_{TOT} = T_1 + T_2 + L_1 + L_2$ so the energy in the cavity as a function of round trips is
$$
E = (1-L_{TOT})^N = \left(1-\frac{L_{TOT}N}{N}\right)^N \approx e^{-L_{TOT} N}
$$
I guess if you read this last section in reverse it's a little bit of a hand-wavey proof that the energy decays like $e^{-2\pi \nu t}$.
Transverse Profile:
I'll assume the cavity mirrors have spherical surfaces.
The modes inside of an optical cavity can be expanded as Hermite Gaussian modes. The modes have a waist which is located at some position (within the cavity if both cavity mirrors are concave, or outside the cavity if one of the mirrors is convex) in space and which has some waist size. These two parameters fully describe the mode. For optical cavities, the modes which survive are exactly those modes whose wavefronts at the locations of the cavity mirrors have radii of curvature that match the radii of curvature of the cavity mirrors. It turns out that if you constrain a Gaussian beam (for a certain wavelength) to have fixed radii of curvature at two positions in space this constrains the size and position of that beam's focus. The output beam is just whatever that mode looks like propagating out past the output mirror. One note is that for certain mirror curvatures and positions there is no possible Gaussian mode supported by that cavity geometry. For more details see the Lasers textbook. But note that the geometry of the mode in the cavity and the output mode is fully determined ONLY by the length of the cavity and the radii of curvature of the two cavity mirrors. I emphatically repeat that that changing the mirror reflectivities (and therefore the cavity finesse and number of round trips) has NO IMPACT WHATSOEVER on the transvere profile of the output beam.