Why the third law of thermodynamics requires reversibility?

Question

A possible expression of the third law of thermodynamics is that, it is impossible to reach absolute zero in a finite number of reversible transformations.

I'don't understand why the transformations must be reversible.

I can understand that, if I use reversible steps to reach $T=0k$, it will take infinite steps. Because, a reversible process, requires that every heat transfer happens between infinitesimal $\delta T$. This involve putting my system in contact with an infinite sequence of reservoirs.

However, i think that, even in case the process is irreversible, it is impossible to reach $T=0k$. Indeed, one way to bring the system to absolute zero, is to put it in contact with an hypothetical heat reservoir at $T=0k$. But, an heat reservoir at $T=0k$ doesn't exist, so this method can't be applied. Similarly, other methods, like an ideal gas expansion, would require the expansion to be infinite, and this is not possible.

So, since it seems that even in the case the transformation is irreversible, it's impossible to bring a system at $T=0K$, could't we restate the third law to the simpler form "It is impossible to reach absolute zero".

Answer 1

There is a graph and rough explanation here on wiki. The fact that it may be impossible to reach absolute zero at all is separate from the third law. The usual statement of the third law states that at absolute zero (the system ground state), there is a unique value of entropy. What should then really be said is that the "it is impossible to reach absolute zero in a finite amount of reversible steps" statement is a consequence of that. In this thought experiment, we don't worry about infinitesimal heat transfer issues. Much like the analysis of a Carnot engine, we take isothermal heat transfer as given.

The reason for this consequence is best understood by the figure at the link I provided. As you know, the only way to reversibly remove heat from a system is isothermally. In this case, the entropy of a system is reduced, and the entropy of the surroundings is increased by the same amount. The only way to reversibly reduce the temperature of a system is by adiabatic work extraction (expansion). If this is done reversibly, the entropy of the system remains constant. In the two graphs of the figure, these are the vertical and horizontal lines.

Since the level curves (and all such level curves) for the other property $X$ must approach a single entropy value at absolute zero (at $T = 0$, $s = s_{0}$ for all level curves $X_{i}$), there is no finite sequence of vertical and horizontal lines that reach $T = 0$. If there were different values of entropy that were possible at absolute zero, then in theory there is a reversible process to get there. Of course, such reversible processes, like Carnot engines, are only theoretical, but the point stands that there is no theoretical path to $T = 0$ in a finite number of steps.

Note that the direction of implication I used here is reversible. This is to say, if you take the third law to be the "there no finite number of reversible processes to reach absolute zero" statement, then you can prove that at absolute zero there can be only a single possible value of entropy. The argument would be basically the same. Suppose there were two such values of entropy at absolute zero for different values of another property $X$. Then you can use the two $X_{i}$ level curves to construct a finite series of reversible processes to reach absolute zero, contradicting the assumption.

Thus, the two statements of the third law are equivalent to one another. It may be that it is still impossible to actually construct a machine that can demonstrate absolute zero (i.e. make a subsystem of itself be at absolute zero), even allowing irreversible processes. I suspect that might be true by the second law actually, since removing heat from absolute zero to any higher temperature requires an infinite amount of work from a Carnot refrigerator, so there is a real consideration that there is no path to absolute zero without infinite exergy input. But that is not what the third law material is discussing.

Answer 2

The third law of thermodynamics is that the entropy of a system approach a constant value when the temperature goes to zero, this value is independent from any parameter that characterize the system. A consequence that can be derived from this law is that, quoting H. B. Callen is that:

... no reversible adiabatic process starting at nonzero temperature can possibly bring a system to zero temperature.

To understand why this is a consequence of the third law of thermodynamics, consider the entropy as a function of the temperature, $T$, and of some other parameter, $X$ (for simplicity consider a closed system). So, we have $S=S(T,X)$.

Given a value of the parameter $X$, the entropy is an increasing function of the internal energy, and, so, of the temperature. The $S-T$ diagram for two different values of the parameter $X_1$ and $X_2$, will be something like the one below:

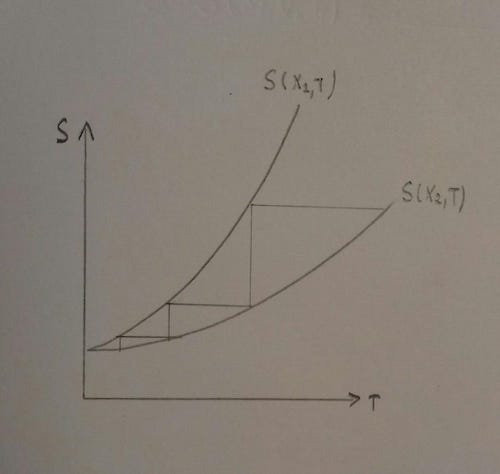
as you can see:

• an adiabatic reversible process is also isentropic, because $dS=\delta Q_{rev}/T=0$, and so, it is represented by an horizontal line. If we change the value of the parameter, in a reversible manner, from $X_2$ to $X_1$, the temperature decrease.

• an isothermal transformation is represented by a vertical line. If we switch back the parameter, from $X_1$ to $X_2$, keeping the temperature constant, the entropy increase.

So, if the horizontal and the vertical transformations are repeated many times, the temperature decreases, but, as you can see, the more $T$ gets close to $0k$, the less it decreases. It would require infinite transformations to go to absolute zero.

Then, can we achieve $T=0k$ if we change the parameter, in an irreversible manner? No. If the parameter changes, from $X_2$ to $X_1$, and the system is adiabatic, $dS \ge Q/T_{surr}=0$. So, in the irreversible transformation, the system state moves from an initial one ($A$) to a final one ($B'$) whose entropy and temperature are larger than those reached through a reversible transformation ($B$). Loosely speaking, an in irreversible transformation adding friction and dissipation is even worst.

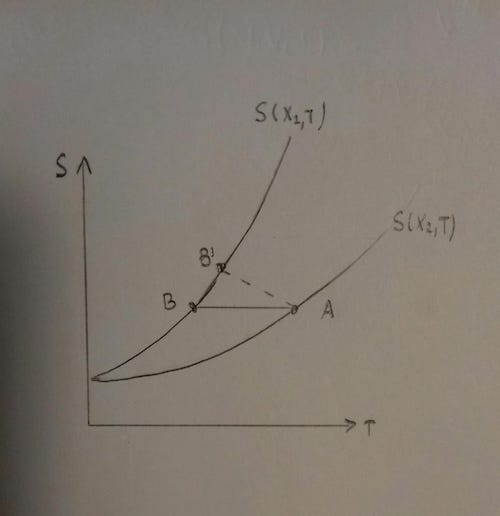
Until now we have considered an adiabatic system, what if the system is not adiabatic? Since we don't have any reservoir at absolute zero, during the cooling process toward zero, at a certain point the environment will become hotter than the system. So heat will enter into the system making things worse.

Is it impossible to reach absolute zero? It may be impossible, but actually, if that is the case, it's not a consequence of the third law of thermodynamics. Indeed the third law involve that it is impossible to reach absolute zero by changing the system parameters, however, there could be other ways to create a very cold system. For example, we could create directly a very cold system, instead of creating one and cool it down (i.e. the laser cooling, in which a flux of gas is slowed down, by a laser, into a very cold gas).

Finally, there may be issues in the concept of reaching absolute zero. For example, it would require an infinite precision. I think this quote from H.B. Callen in on the point:

The question of whether the state of precisely zero temperature can be realized by any process yet undiscovered may well be an unphysical question, raising profound problems of absolute thermal isolation and of infinitely precise temperature measurability.

So, one problem in reaching $0k$ is that it would require an infinite precision. However, when it comes to continuous physical quantities, a measurement is intended with a certain uncertainty.