Free body diagram of mass-spring system #2

Question

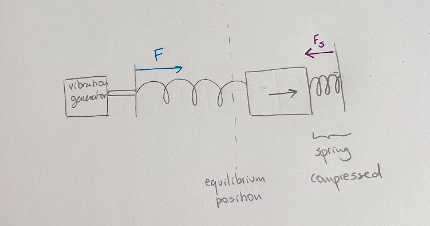
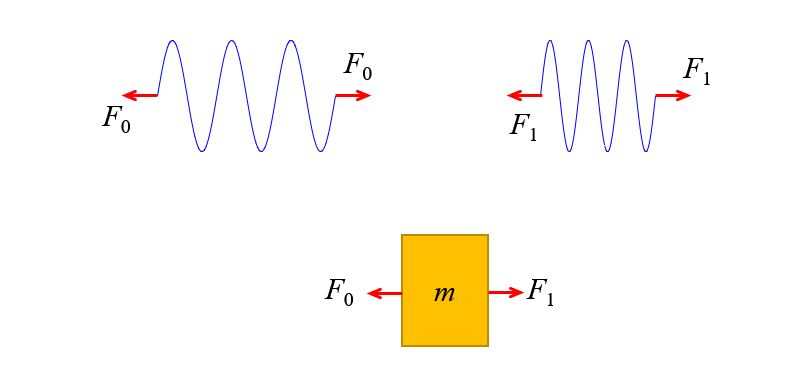
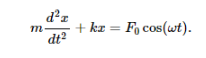I've attempted to draw a free body diagram of the forces acting on the mass again, but I'm confused about the directions. In many of the sources that I've read, they say that the Fres = Fs + F. Fs is the spring force and F is the force from the oscillation generator. But considering when the mass is displaced maximum to the right, the spring of the left will (almost) have no effect since its tension reduce. Only the compressed spring on the right will exert a spring force on the mass, right? Also, when the mass is oscillating in phase with the oscillation generator, that is if the force pushes to the right, the mass will displace to the right. Therefore, why is Fres = Fs + F, and not Fres = F - Fs (if to the right is the positive direction)?

Moreover, the equation I get should be:

So how do I draw the free-body diagram to get that?

Answer 1

If this is the situation

then you need to recognize that there are 2 degrees of freedom here. The end on the left with displacement $x_0$ and the mass with displacement $x_1$. Positive displacement directions shown.

It makes no sense to apply a force on the left on a massless spring as the force just transfers through to the mass, and the deflection on the spring on the left bears no significance at all.

But if you prescribe the motion on the left, like with a shaker table, then you get a better idea of the dynamics of the system.

In any case, each spring has equal and opposite forces on its ends. The means the free-body diagrams looks as follows (all forces shown positive).

Now you need to related the forces $F_0$ and $F_1$ to the displacement $x_0$ and $x_1$, and then apply the equations of motion to the mass $m$.