When we find the solutions of the differential equations leading to the expression for displacement of a body in SHM as a corollary we derive that $\omega=2\pi/T$, Now from what I make out considering it as a projection of circular motion , the body always subtends equal angle at the origin every second or the angle subtended by a body is always integral multiples of $\omega$. Now my question is how is this omega constant because the displacement per second covered by body will keep on decreasing as force is proportional to distance. Am I missing something here?
$\begingroup$
$\endgroup$
12
-
$\begingroup$ You yourself conceded that you are projecting uniform circular motion in phase space to coordinate space. The force and potential energy oscillates sinusoidally, out of phase with the kinetic energy which likewise oscillates. It should be in your book. $\endgroup$– Cosmas ZachosCommented Feb 8, 2023 at 15:39
-
$\begingroup$ I am asking why is the angular frequency constant? $\endgroup$– TheCuriousOneCommented Feb 8, 2023 at 16:09
-
1$\begingroup$ ? Uniform circular motion not good enough for you? $\endgroup$– Cosmas ZachosCommented Feb 8, 2023 at 16:11
-
$\begingroup$ But why are we associating it with uniform circular motion? $\endgroup$– TheCuriousOneCommented Feb 8, 2023 at 16:14
-
2$\begingroup$ Geometry is intuition. $\endgroup$– Cosmas ZachosCommented Feb 8, 2023 at 16:42
|
Show 7 more comments
4 Answers
$\begingroup$
$\endgroup$
16
" how is this omega constant because the displacement per second covered by body will keep on decreasing as force is proportional to distance." Imagine a point, P, going round a circular path with constant angular velocity, $\omega$, about the circle centre. Now visualise the projection, Q, on to, say, the vertical diameter, of this moving point. Q will move fast when it is near the circle centre, and more and more slowly as it approaches the end of the diameter – and so on. In other words the motion of Q will be the same as that of a mass on a spring performing simple harmonic motion, even though $\omega$ is constant.
That $\omega$ really is constant follows inescapably from the mathematics, specifically that the solution to the Newton's law equation, $$m\frac{d^2 x}{dt^2}=-kx,$$ is $$x=A\sin(\omega t +\phi)$$ in which $\omega=\sqrt{k/m}$ and is a constant because $k$ and $m$ are constants. $A$ and $\phi$ are arbitrary constants.
By definition $A\sin \theta$ is the $y$ coordinate of the point at angle $\theta$ round a circle of radius $A$ (centred on the origin) from the +$x$ axis. Therefore $A\sin(\omega t +\phi)$ is the $y$ coordinate of a point on the circle at angle $(\omega t + \phi)$ to the +$x$ axis). But this angle increases by $\omega$ radians per unit time, that is $\omega$ is the point's angular velocity. Hence the projection approach discussed above.
answered Feb 8, 2023 at 21:00
-
$\begingroup$ Could you explain what happens(excluding math) if we assume the SHM to be a non uniform motion? And what would be its complications $\endgroup$ Commented Feb 12, 2023 at 6:30
-
$\begingroup$ What, I wonder, do you mean by "a non-uniform motion"? I would call SHM, as given by $x=A\sin(\omega t+\phi)$, non-uniform as the velocity keeps changing over each cycle. We don't, in any case, have any choice over what we can assume about SHM, as the motion has to obey $x=A\sin(\omega t+\phi)$, or it won't be SHM ! $\endgroup$ Commented Feb 12, 2023 at 8:45
-
$\begingroup$ Have you studied the displacement-time graph and velocity-time graph for a body doing SHM? And – a question that must surely be asked – do you have a textbook that deals with SHM? $\endgroup$ Commented Feb 12, 2023 at 15:14
-
$\begingroup$ Yes I have. Ok a better way to put the question is why omega=sqrtk/m $\endgroup$ Commented Feb 12, 2023 at 15:27
-
$\begingroup$ We are defining $\omega$ to be $\sqrt{(k/m)}$. What, I suspect, is giving you difficulty is the interpretation of $\omega$ as an angular velocity. To understand this you need to understand the mathematics that shows why $x=A\sin(\omega t+\phi)$ is a solution of the Newton's second law equation $m \frac{d_2x}{dt^2}=-kx$. My answer deals with the meaning or interpretation of $A\sin(\omega t+\phi)$. $\endgroup$ Commented Feb 12, 2023 at 15:38
$\begingroup$
$\endgroup$
The projection of the (two-dimensional) uniform circular motion of an object onto a (one-dimensional) axis is an exact mathematical analog to simple harmonic motion. Uniform circular motion is identical to rotation about an axis with constant angular velocity, $\omega$. You can see the details of this connection, e.g., in pp 2-5 of these notes.
$\begingroup$
$\endgroup$
2
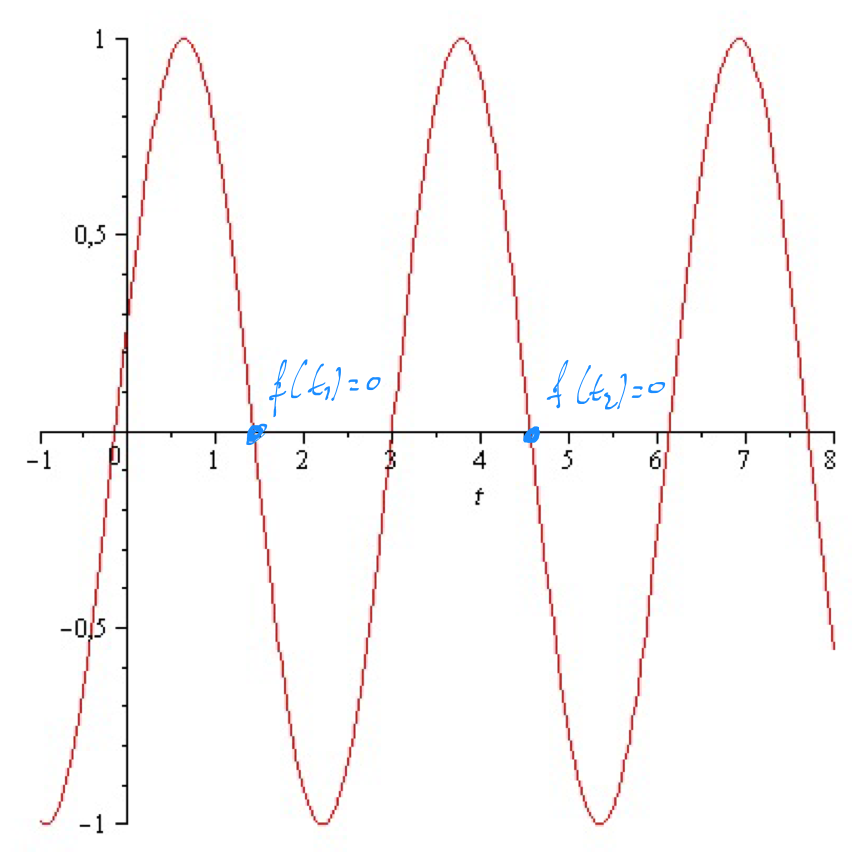
Assume you have this time plot .
first we identify this plot as a $~f(t)=A\,\sin(a\,t+\phi)~$ . To "measure" the period $~T~$ of the function, we obtain the zero-crossing of $~f(t_i)=0~$
$$ T=4.562388980-1.420796327=3.141592653$$
thus the function is now : $$f(t)=A\,\sin(\underbrace{\frac{2\pi}{T}}_{a}\,t+\phi)\tag 1$$
we defined a new parameter , call it angular velocity which is:
$$\frac{2\,\pi}{T}:=\omega~,\text{unit}~[\frac 1s]$$
Eq. (1) is also the solution of SHM
$$\ddot x+\omega^2\,x=0$$
-
$\begingroup$ Why are you assuming a becomes 2pi/T? $\endgroup$ Commented Feb 13, 2023 at 1:34
-
$\begingroup$ Perhaps you are looking for this answer . Put the data $~\frac{2\pi}{T}~$ you obtain a=2 , thus $f(t)=\sin(a\,t+\phi)$ my Ansatz $\endgroup$– EliCommented Feb 13, 2023 at 8:50
$\begingroup$
$\endgroup$
In linear motion angular frequency makes no immediate sense, it is just $2\cdot\pi\cdot f$ but since it is in the formula for harmonic motion it is easier to write $sin(\omega t)$ instead of $sin(2\pi f t)$ You can proof, that the linear motion is the same as the projection of uniform circular motion.
