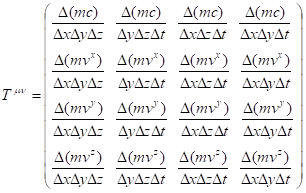First, to correct a misconception that is widespread even in the Physics literature: the object that the various densities and fluxes belong to as components is not a tensor, but is (as the term "density" already indicates) a tensor density. In particular, it is the tensor density that appears in the continuity equation for the transport law $∂_ρ 𝔗^ρ_ν = -𝔎_ν$. Here, I'm using the notation $∂_ρ = ∂/∂x^ρ$, and the summation convention throughout.
Second: nor is it rank (0,2) or (2,0), but rank (1,1), when expressed in its natural, pre-metric, form. It is related to the corresponding tensor $T_{μν}$ by: $𝔗^ρ_ν = \sqrt{|g|} g^{ρμ} T_{μν}$, where $g_{μν}$ comprises the components of the metric, $g^{ρμ}$ the components of the inverse metric and $g$ is the determinant of the matrix formed of $g_{μν}$.
The tensor and tensor density are, by construction symmetric; the symmetry condition being expressible as $T_{μν} = T_{νμ}$, or equivalently, as $g_{νρ} 𝔗^ρ_μ = g_{μρ} 𝔗^ρ_ν$.
The tensor density, itself, is obtained from the canonical stress tensor, which I'll denote $𝔓$, by a correction of the form $𝔗^ρ_ν = 𝔓^ρ_ν + ∂_μ 𝔭^{μρ}_ν$, involving an additional tensor density that is anti-symmetric in $(μ,ρ)$: $𝔭^{μρ}_ν = -𝔭^{ρμ}_ν$. The anti-symmetry ensures that the transport law applies equivalently to $𝔓$ and to $𝔗$. The right choice for $𝔭$ yields a tensor density $𝔗$ and tensor $T$ from $𝔓$ that is symmetric.
The canonical stress tensor for a field theory given by an action principle with an action integral of the form $S = ∫ 𝔏 d^4x$, is given by
$$𝔓^ρ_ν = \frac{∂𝔏}{∂v^A_ρ} v^A_ν - δ^ρ_ν 𝔏,$$
where $𝔏$ is the corresponding Lagrangian density (again: density) and - for 4D space-time with coordinates $\left(x^0, x^1, x^2, x^3\right) - d^4x = dx^0 ∧ dx^1 ∧ dx^2 ∧ dx^3$; for a field whose components are given by $q^A$ and gradients by $v^A_μ = ∂_μ q^A$, where $δ^ρ_ν$ is the Kronecker delta, as usual, given by $δ^ρ_ν = 1$ if $ν = ρ$ and $δ^ρ_ν = 0$ if $ν ≠ ρ$.
Use the following to denote the respective dimensions: $q^A = [A]$, $x^μ = [μ]$, and denoting the dimension of $S$ by $[S] = H = ML^2/T$, where $M$, $L$ and $T$ respectively denote the dimensions of mass, length and duration. Also, write $Ω = \left[d^4x\right] = [0][1][2][3]$. Then:
$$
\left[v^A_μ\right] = \frac{[A]}{[μ]}, \hspace 1em [𝔏] = \frac{H}{Ω}, \hspace 1em [𝔗^ρ_ν] = [𝔓^ρ_ν] = \frac{[𝔏]}{[A]/[ρ]} \frac{[A]}{[ν]} = \frac{H}{Ω}\frac{[ν]}{[ρ]}.$$
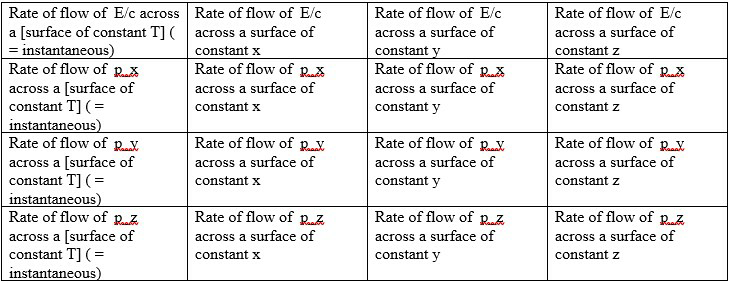
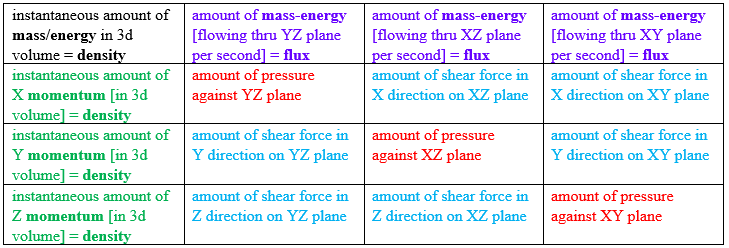
The usual interpretation for $𝔓$ is that the 3-form $𝔓^ρ_ν ∂_ρ ˩ d⁴x$ is the 3-current for the momentum component $p_ν$, meaning that $𝔓^0_0$ is an energy density, $\left(𝔓^1_0,𝔓^2_0,𝔓^3_0\right)$ its corresponding flux, and for $j = 1, 2, 3$, $𝔓^0_j$ is the density for the momentum component $p_j$, and $\left(𝔓^1_j,𝔓^2_j,𝔓^3_j\right)$ its corresponding flux. In coordinates, where $x^0$ denotes time, and $\left(x^1,x^2,x^3\right)$ are Cartesian (or at the least: coordinates with length as their dimension), the key dimensions in question are $Ω = TL^3$ and
$$\left[𝔓^0_0\right] = \frac{H}{Ω} = \frac{ML^2/T}{TL^3} = \frac{M}{LT^2} = \left[𝔓^1_1\right] = \left[𝔓^2_2\right] = \left[𝔓^3_3\right],$$
which are respectively the dimensions for both energy density and pressure, since
$$\frac{ML^2/T^2}{L^3} = \frac{M}{LT^2} = \frac{ML/T^2}{L^2}.$$
For the momentum density, we obtain
$$\left[𝔓^0_1\right] = \left[𝔓^0_2\right] = \left[𝔓^0_3\right] = \frac{ML^2/T}{TL^3}\frac{T}{L} = \frac{M}{L^2T} = \frac{ML/T}{L^3}.$$
In all cases, the fluxes and densities have the expected relations to one another (flux = density multiplied by speed):
$$\left[𝔓^1_ν\right] = \left[𝔓^2_ν\right] = \left[𝔓^3_ν\right] = \left[𝔓^0_ν\right] \frac{L}{T}.$$
The Dimensions of $T_{μν}$
This depends on what convention is used for the metric. If the metric denotes a line element for proper time, then dimensional analysis would yield
$$T^2 = \left[g_{μν} dx^μ dx^ν\right] = \left[g_{μν}\right][μ][ν] ⇒ \left[g_{μν}\right] = \frac{T^2}{[μ][ν]}.$$
If the line elements denotes proper distance, which is the prevailing convention, then the analysis would be modified to the following:
$$L^2 = \left[g_{μν} dx^μ dx^ν\right] = \left[g_{μν}\right][μ][ν] ⇒ \left[g_{μν}\right] = \frac{L^2}{[μ][ν]}.$$
That's the convention we'll adopt here.
Under that convention $g = L^8/Ω^2$ and $\sqrt{|g|} = L^4/Ω$ ... hence $\sqrt{|g|} d^4x = L^4$, independently of what dimensions the coordinates individually have. For the inverse metric, we have
$$[g^{μν}] = \frac{[μ][ν]}{L^2}.$$
As a result, we obtain:
$$\frac{H}{Ω}\frac{[ρ]}{[ν]} = \left[𝔗^ρ_ν\right] = \frac{L^4}{Ω} \frac{[ρ][μ]}{L^2} \left[T_{μν}\right] ⇒ \left[T_{μν}\right] = \frac{H}{L^2[μ][ν]} = \frac{M}{T[μ][ν]}.$$
In particular, for $i, j = 1, 2, 3$, this yields:
$$\left[T_{00}\right] = \frac{M}{T^3}, \hspace 1em \left[T_{i0}\right] = \left[T_{0j}\right] = \frac{M}{LT^2}, \hspace 1em \left[T_{ij}\right] = \frac{M}{L^2T}.$$
The Correct Coupling Coefficient For Einstein's Equations
This confusion between tensor and tensor density has led to the widespread use of the wrong expression for the coupling coefficient for Einstein's Field Equations - even on a wall over at Leiden University, itself. In fact, in the literature there are several different expressions used for the coefficient.
The Hilbert tensor density is given in terms of the corresponding Lagrangian density by
$$𝔗_{μν} = -2\frac{∂𝔏}{∂g^{μν}}.$$
The fact that the expression is substantially simplified when expressed in terms of densities rather than tensors is a tell that you're actually dealing with densities, not tensors. The corresponding dimension
$$\left[𝔗_{μν}\right] = \frac{H/Ω}{[μ][ν]/L^2} = \frac{H}{Ω}\frac{L^2}{[μ][ν]}$$
is consistent with what we already have, noting that $𝔗_{μν} = g_{μρ}𝔗^ρ_ν$, so this checks out.
The gravitational part of the Lagrangian is given, by $k √|g| R$, for a suitable scale factor $k$, where $R$ is the curvature scalar. The dimensions for the various geometric quantities are
$$Γ^ρ_{μν} = \frac{[ρ]}{[μ][ν]}, \hspace 1em R^ρ_{σμν} = \frac{[ρ]}{[σ][μ][ν]}, \hspace 1em R_{μν} = \frac{1}{[μ][ν]},$$
respectively for the connection, curvature tensor and Ricci tensor.
This is independent of what convention we adopt for the line element $g_{μν} dx^μ dx^ν$. The dimension for the curvature scalar, however, is not:
$$[R] = \left[g^{μν}R_{μν}\right] = \frac{[μ][ν]}{L^2}\frac{1}{[μ][ν]} = \frac{1}{L^2}.$$
Using this, we find that the scalar factor must have the following dimension:
$$\frac{H}{Ω} = \left[k \sqrt{|g|} R\right] = [k] \frac{L^4}{Ω} \frac{1}{L^2} = [k] \frac{L^2}{Ω} ⇒ [k] = \frac{H}{L^2} = \frac{M}{T}.$$
Noting that
$$[G] = \frac{L^3}{MT^2}, \hspace 1em [c] = \frac{L}{T},$$
where $G$ denotes Newton's gravitational coefficient and $c$ the vacuum speed of light, it follows that
$$[k] = \frac{L^3}{T^3} \frac{MT^2}{L^3} = \left[\frac{c^3}{G}\right].$$
The scale factor used in the Einstein-Hilbert action
$$S = \frac{1}{2κ} \int \sqrt{|g|} R d^4 x$$
is $k = 1/(2κ)$ and, up to powers of $c$ in 4 dimensions, $κ = 8πG$. (Note also the qualification: the factor $8π$ is specific to 4 dimensions and changes for geometries of dimensions other than 4). The dimensional analysis, here, indicates this power is $c^3$, not the more commonly-seen $c^4$ and that
$$κ = \frac{8πG}{c^3}.$$
Some literature references get this right. Some use $c^4$, instead of $c^3$, and Einstein used $c^2$. References seen on-line almost all use $c^4$, which is wrong.
The situation is analogous to what used to happen with everyone using "Lorentz gauge" instead of (the correct) "Lorenz gauge", before being corrected by (the late) J. D. Jackson.