0
$\begingroup$

The following is an example from David Morin's Introduction to Classical Mechanics.

A rope wraps an angle $\theta$ around a pole. You grab one end and pull with a tension $T_0$. The other end is attached to a large object, say, a boat. If the coefficient of static friction between the rope and the pole is $\mu$, what is the largest force the rope can exert on the boat, if the rope is not to slip around the pole?

Solution
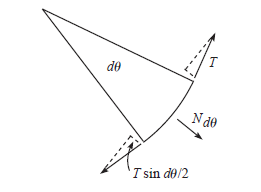
Consider a small piece of the rope that subtends an angle $d\theta$. Let the tension in this piece be $T$ (which varies slightly over the small length). As shown in the figure, the pole exerts a small outward normal force, $Nd\theta$ , on the piece. This normal force exists to balance the “inward” components of the tensions at the ends. These inward components have magnitude $T \sin(d\theta/2)$. Therefore, $Nd\theta = 2T \sin(d\theta/2)$.
The solution then continues to calculate the inequality $T\leq T_0e^{\mu\theta}$.

enter image description here
I do not know how to get the magnitude for the inward components as $T sin(d\theta/2)$.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

Since the axial action $T$ is aligned with the axis of the rope at every point, and since the infinitesimal element of the rope describe a arc of circumference of angle $d \theta$, the angle between the directions of the axial action $T$ at the free ends of the elementary rope is $d \theta$.

Now, draw the bisector of angle $d \theta$. This is your radial direction. The projection of each $T$ along this direction is $T \sin(d\theta/2)$.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Is $T$ not in the tangential direction to the rope or along the direction of the rope around the pole? By axial action, do you mean $T$ is along the direction of the pole? $\endgroup$
    – 1500kook12
    Commented Sep 6, 2022 at 19:05
  • $\begingroup$ $T$ is along the direction of the rope, but this direction changes, since the rope is rolled up around the pole. $\endgroup$
    – basics
    Commented Sep 6, 2022 at 19:24
  • $\begingroup$ as an example, in your picture the rope describes a small arc of a circle of angle $d \theta$ $\endgroup$
    – basics
    Commented Sep 6, 2022 at 19:26
  • $\begingroup$ To confirm I'm getting it right, should I use the theorem that the angle between the tangents and the angles subtended by the radii of the arc are supplementary? where the tangents would be $T$ and angle subtended by the radii would be $d\theta$ $\endgroup$
    – 1500kook12
    Commented Sep 8, 2022 at 13:06
  • $\begingroup$ Or that the angle between the two directions indicated by the 2 arrows T, is the same as $d \theta$ $\endgroup$
    – basics
    Commented Sep 8, 2022 at 14:02

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.