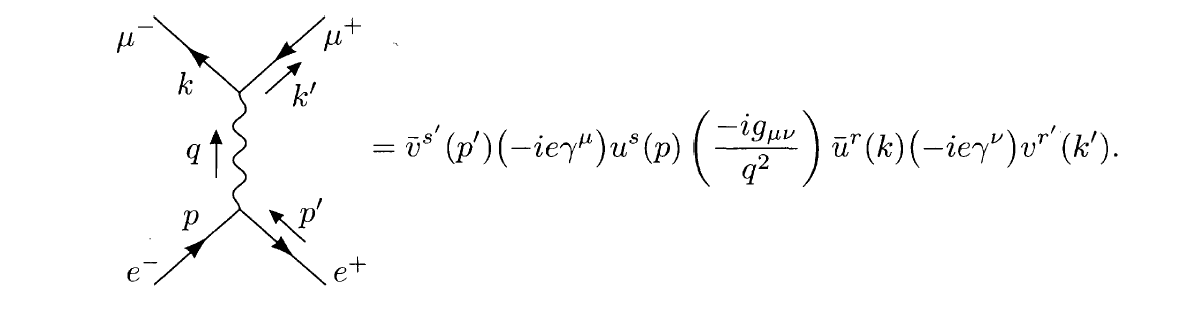Feynman diagram with different particles

Question

I'm studying Peskin and Schroeder chapter 5. At the beginning of section 5.1, the book tries to compute S matrix of $e^+e^-\rightarrow \mu^+\mu^-$. Using the Feynman from section 4.8, we can draw a Feynman diagram (bottom of page 131) and write down its amplitudes

However I think this amplitude comes from the following term in the perturbation expansion (maybe up to a sign):

$$\bigg\langle 0\bigg|\,a_{k,r} b_{k',r'} (-i)\int_{\mathbb{R}^4}e\overline{\psi}\gamma^\mu\psi A_\mu(-i)\int_{\mathbb{R}^4}e\overline{\psi}\gamma^\mu\psi A_\mu \,a_{p,s}^\dagger b_{p',s'}^\dagger\bigg|0\bigg\rangle.$$

My question is: since we are dealing with two different species of particles here, do the annilation operators and creation operators $a_{k,r},b_{k',r'},a_{p,s},b_{p',s'}$ act on the same Hilbert space? If not, then the contraction procedure no longer works and in effect the expression does not make sense.

Also in the expansion of $\psi$, what do the creation operators $a^\dagger, b^\dagger$ create? Electrons or muons?

Answer 1

QFT hides a lot of tensor products inside its notations.

All the operators are acting on the same Hilbert space, but this Hilbert space is a tensor product between Hilbert spaces corresponding to each of the particles. In particular, muons and electrons are in different "sectors" of this Hilbert space.

The contraction procedure works as long as you remember that you must create and annihilate all of the particles in the diagram. Each propagator corresponds to this: create a particle somewhere, annihilate it somewhere else.

The trick in your expression is then that there is more than one set of fermionic creation and annihilation operators and more than one fermion field. The electron and positron corresponds to a field (call it $\psi_e$, for example), while muon and antimuon have a different one (say $\psi_\mu$). Both of them interact with the photon in the same way and the only difference between them is their mass, so it is common to not write explicitly on the QED Lagrangian that we actually are dealing with different fields. One could also consider the tau and antitau, which would then lead to a third field.

Edit

It might be better to write the QED Lagrangian explicitly considering these remarks. It reads

$$\mathcal{L} = - \frac{1}{4}F^{\mu\nu}F_{\mu\nu} + \sum_{f} \Big(\bar{\psi}_f \left(i \gamma^\mu D_\mu - m_f \right)\psi_f - e_f \bar{\psi}_f \gamma^\mu \psi_f A_\mu\Big),$$ where $f$ indicates each existing charged fermion (i.e., it runs over the charged leptons and over all quarks), $\psi_f$ is the field associated to the fermion $f$, $m_f$ is the mass of the fermion $f$, and $e_f$ is the electric charge of the fermion $f$.