Fermi statistics in the Feynman rules for the Gross-Neveu model

Question

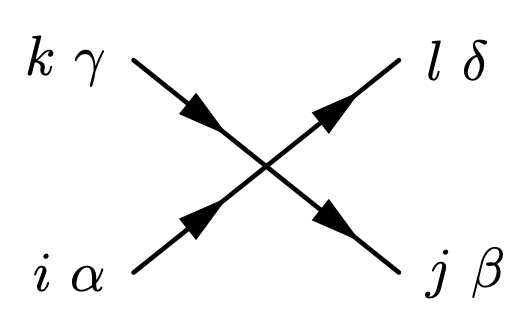
I am trying to understand the Feynman rule for the 4-fermi interaction in the Gross-Neveu model. Based on this Peskin & Schroeder solution 12.2 and this and this Stack Exchange clarification, I have the 4-fermi term as

\begin{equation} \frac{1}{2}g^2 (\bar{\psi_i}\psi_i)^2 \end{equation}

where $i$ labels the species of fermions, 1 through $N$. The corresponding Feynman rule is

\begin{equation} ig^2(\delta_{ij}\delta_{kl}\delta_{\alpha\beta}\delta_{\gamma\delta}+\delta_{il}\delta_{jk}\delta_{\alpha\delta}\delta_{\gamma\beta}) \end{equation}

for the vertex labeled as follows:

I have three questions about this.

(1) I see that the two groups of delta functions comes from pairing each incoming arrow with each outgoing arrow. Why isn't there a relative sign that comes from permuting the $\psi$'s to make these different pairings?

(2) Why doesn't the factor of $1/2$ from the Lagrangian end up in the Feynman rule?

(3) It seems like these combinations of delta functions overcount some terms because they don't take into account fermi statistics. For example, say I chose the representation

\begin{equation} \gamma^0=\sigma_1, \gamma^1=i\sigma_2 \end{equation}

and then, for $N=1$ (dropping species subscripts), calculate

\begin{equation} (\bar{\psi}\psi)^2=\psi^{\dagger}\gamma^0\psi \psi^{\dagger}\gamma^0\psi = (\psi^2)^{*}\psi^1(\psi^2)^{*}\psi^1+(\psi^2)^{*}\psi^1(\psi^1)^{*}\psi^2+(\psi^1)^{*}\psi^2(\psi^2)^{*}\psi^1+(\psi^1)^{*}\psi^2(\psi^1)^{*}\psi^2 =2(\psi^1)^{*}\psi^2(\psi^2)^{*}\psi^1 \end{equation}

where the superscripts are spinor indices, and some terms are zero by fermi statistics. A term like this can certainly be produced by the Feynman rule above. But it appears as though the Feynman rule is making extra terms like $(\psi^1)^{*}\psi^1(\psi^1)^{*}\psi^1$. Shouldn't those be zero by fermi statistics? Is there something wrong with this Feynman rule?

Answer 1

I think this resolves the issues:

(1) There should in fact be a relative sign in the rule for the four-fermi vertex:

\begin{equation} i g^2 (\delta_{ij}\delta_{kl}\delta_{\alpha\beta}\delta_{\gamma\delta}-\delta_{il}\delta_{kj}\delta_{\alpha\delta}\delta_{\gamma\beta}) \end{equation}

You can see that the expression should be antisymmetric in the pairs of $\psi$ or $\bar{\psi}$ indices from evaluating:

\begin{equation} \bar{\psi}_i^{\alpha}\psi_j^{\beta}\bar{\psi}_k^{\gamma}\psi_l^{\delta}(\delta_{ij}\delta_{kl}\delta_{\alpha\beta}\delta_{\gamma\delta}-\delta_{il}\delta_{kj}\delta_{\alpha\delta}\delta_{\gamma\beta})=2\bar{\psi}_i^{\alpha}\psi_i^{\alpha}\bar{\psi}_k^{\gamma}\psi_k^{\gamma} \end{equation}

(2) With the rule as written (with no $1/2$), that just sets up what you mean by symmetry factors. If you include the $1/2$ from the Lagrangian, properly do the Taylor expansion, and account for the factor of 2 from the antisymmetrization of the above expression, you find e.g. for the following diagrams

the familiar-looking symmetry factors of (a) 1, (b) 2, (c) 1. Explicitly, consider diagram (a). From the Taylor expansion, Lagrangian, and antisymmetrization of indices, we have in the denominator $2!*(2*2)^2=32$. The $k$ leg can be contacted into the upper vertex 2 ways. The $l$ leg, another 2 ways. Similarly 2 for $i$ and 2 for $j$. There is only one remaining way to contract the legs together in the loop. Finally, we get a factor of $2!$ from the interchange of contractions into the upper and lower vertices. Overall that puts a factor of 32 in the numerator. Thus with the vertex normalized to $ig^2*$(delta functions) we see the symmetry factor for (a) is 1. Diagram (b) is a little different. We get $2!$ from vertex interchange. Leg $k$ can contract in the left vertex 2 ways. Leg i then only has one option. Similarly, 2 for $l$ and 1 for $j$. Then there are 2 ways to contract the internal legs in the loop. We are left with 16 in the numerator, giving the diagram overall a factor of $16/32$=$1/2$. Thus we interpret the remaining 2 in the denominator as the symmetry factor.

(3) This is resolved by the minus sign in (1).