First, let's address some possible misconceptions: (1) that the ice can't melt because it's at the same temperature as the surroundings or because we're not actively heating it, or (2) that heat transfer doesn't occur toward the melting ice (regarding your statements "how is it even possible ?", "there could be no energy flow since there is no temperature difference", and "How will it absorb energy from the surrounding ice which is also at the same temperature ?").
In regelation, we force melting by making the ice unstable in a way that's distinct from heating it. (More on that below.) The latent heat associated with the phase change (specifically, the enthalpy of fusion) must be paid for by sensible heating from elsewhere. In this way, the melting absorbs energy from the immediate surroundings, cooling them, creating a temperature gradient, and thus driving heat transfer from the broader surroundings through Newton's law of cooling.
Now, let's look at the thermodynamic reason for melting.
A phase transition temperatures is simply the intersection in the Gibbs free energy (or the chemical potential, which is just the molar Gibbs free energy) of two phases:
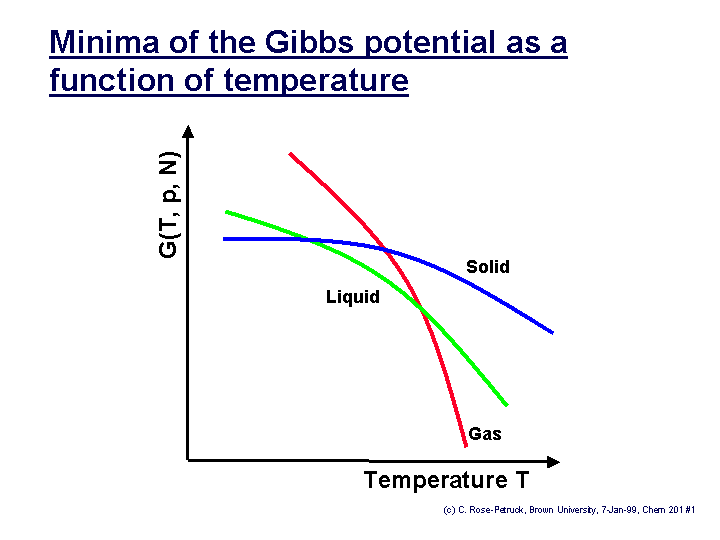
The Gibbs free energy $G$ incorporates the enthalpy $H$, temperature $T$, and entropy $S$ as $G\equiv H-TS$, so we find that the temperature-dependent slope of every curve is that phase's entropy. (Observe that high-entropy gas always has a steeper curve than low-entropy condensed matter.) The lower $G$ curve is the more stable one. Accordingly, we see solids melt into liquids and then boil into gases as the temperature increases.
Regelation occurs because applying a pressure to a region of solid ice increases its Gibbs free energy as $G=F+PV$, where $F$ is the Helmholtz free energy, $P$ is pressure, and $V$ is volume. Pushing the blue curve up in the diagram clearly lowers the equilibrium temperature with liquid water. (The green curve doesn't move because the liquid, being a fluid, can simply move away from a region of local compression; thus, the Gibbs free energy of the liquid remains unchanged.)
Then, let's look at the kinetics and heat transfer during the process.
Assume that compression (from a weighted wire stretched over the top of the ice, as you reference) lowers the melting temperature from $T_\text{m}=0^\circ \text{C}$ to $T^\prime_\text{m}<0^\circ \text C$. We have compressed solid ice at 0°C (or somewhat below, but assumed to be above $T^\prime_\text{m}$). The compressed ice accordingly melts and cools below its original temperature, which draws thermal energy from the surrounding region (uncompressed ice that started at the original temperature and is now cooling) to supply that latent heat of fusion. The temperature of the adjacent surroundings is lowered correspondingly by that sensible heat transfer.
Depending on the geometry, the melted water may flow away (probably to refreeze elsewhere). This exposes fresh ice that consequently melts under the applied pressure. Now, if the surrounding ice has cooled to the new compression melting temperature $T^\prime_\text{m}$ because of all this latent heat being absorbed, melting—even under compression—is no longer thermodynamically favorable. This is a temporary situation, though: heat transfer from the surrounding ice ($>T^\prime_\text{m}$) warms the compressed ice and allows continued progression. In this thought experiment, therefore, the wire passes downward entirely through the solid ice, melting the ice below it and allowing refreezing above it.

