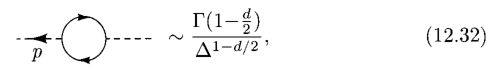Peskin and Schroeder p. 409 “quadratically divergent mass renormalization”

Question

I have three questions about page 409 of Peskin and Schroeder.

First, they state that the diagram

where $\Delta = m_f^2 - x(1-x)p^2|_{m_f=0}$, has a pole at $d=2$ “corresponding to the quadratically divergent mass renormalization.” If I understand correctly, the expression (12.32) evaluated at the renormalization scale $p^2 = -M^2$ should cancel the “propagator counterterm” $(p^2\delta_Z - \delta_m)$. Is the “quadratically divergent mass renormalization” $\delta_m = m_0^2Z - m^2$? Why is it quadratically divergent at $d=2$? In general, if the one-loop correction to the propagator as a function of the parameter $d$ has poles in the complex planes, which poles should we cancel with a counterterm and why? To define the counterterms, shouldn’t we be evaluating (12.32) and subsequent higher-order loop corrections all at $d=4$?

Second, why is the expression (12.32) at $d=4$ equal to

$$-p^2\left(\frac{1}{2-d/2} + \log\frac{1}{-p^2} + C\right)?\tag{12.33}$$

Third, why can the last expression be simplified to

$$-p^2\left(\log\frac{\Lambda^2}{-p^2}+C\right)?\tag{12.34}$$

Answer 1

I will try to answer all your questions, but I am not sure I can... I will give it a try though and then you can comment if you like for further clarification.

1. The book says that expression (12.32) has a pole at $d=2$. Namely (and according to the book's Appendix) $$\frac{\Gamma(1-\frac{d}{2})}{\Delta^{1-\frac{d}{2}}}=\Gamma(\frac{\epsilon}{2})\Delta^{-\frac{\epsilon}{2}}=\frac{2}{\epsilon}+\text{finite terms}$$ where I have simply substituted in the formula $d=2-\epsilon$ and used the Taylor expansions for the two functions. This is divergent, but beware! It's divergence has nothing to do with the fact that the pole at $d=2$ corresponds to the quadratically divergent mass renormalization. The authors do not refer to the way the term diverges. Instead, they associate the divergence to mass renormalization because it is a correction to the two propagator. In general, the poles of the propagator corrections that are multiplied with $p^2$ are cancelled with the help of the $\delta_Z$ counter term, whereas all the independent (of the momentum) terms are cancelled with the help of the $\delta_m$ counter term. This is the standard practice in renormalization techniques (If I understand things correctly). The counter terms are defined order by order. Here, we evaluate the divergent diagrams to loop order and absorb the infinities into the counter terms. If we want to extend this procedure to the next-to-loop order, then we have to calculate the terms corresponding to the diagrams containing two loops and repeat the procedure there.

2. The expression you write is divergent at $d=4$ and this can be seen from $$\frac{\Gamma(1-\frac{d}{2})}{\Delta^{1-d/2}}= \Delta\frac{\Gamma(2-\frac{d}{2})}{(1-\frac{d}{2})}\Big(\frac{1}{\Delta}\Big)^{2-\frac{d}{2}}= \Delta\Gamma(2-\frac{d}{2}) \frac{1}{(1-\frac{d}{2})}\Big[1-(2-\frac{d}{2})\log\Delta+C\Big]$$ where I used the formulas from the Appendix. We can see that the product $\Delta/(1-\frac{d}{2})$ is finite and in addition, since in the massless limit $\Delta\sim -p^2$, it is easy to identify that expression (12.32) is proportional to $$-p^2\frac{1}{(2-\frac{d}{2})}\Big[1-(2-\frac{d}{2})\log\Delta+C\Big]$$ where an additional $1/(2-\frac{d}{2})$ factor comes from expanding the $\Gamma$ function in the $\epsilon\rightarrow0$ limit. The equation above is actually Eq. (12.33).

3. Last, the book does not say that the last expression can be reduced to $$-p^2\Big(\log\frac{\Lambda^2}{-p^2}+C\Big)$$ Instead, the authors claim that expressions of the form (12.33), i.e. propagators at the loop level, can be written in the previous form. This is actually true if instead of dimensional regularization, one follows the Pauli-Villars procedure described in Chater 7.1. So, I refer you to the latter Chapter to see how this is done for the case of QED and if you like, you can apply the procedure to Yukawa theory to deduce an expression for a loop order propagator. You will see that the form will be the one given by the book.

I hope all of these helps...