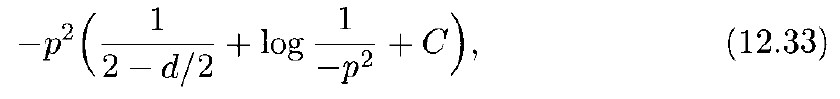Peskin & Schroeder, section 12.2, page 409, analytic continuing from $d=2$ to $d=4$?

Question

In Peskin & Schroeder section 12.2，page 409, consider the one-loop correction to the propagator in Yukawa theory, it has the form

The authors first spot the pole at $d=2$, and find out that it could be completely absorbed by counterterm $\delta_m$, then they analytically continuing this equation from $d=2$ to $d=4$, and claim that we don't need to worry about the $\delta_m$ anymore, since in the limit $d\to4$, this equation has the form Eq(12.33)

My question is, why couldn't we just stick with the situation $d=4$ and claims that in the massless limit of scalar field, there will be no contribution to the mass shift, why should we bother to consider the pole at $d=2$?

Additionally, even if we absorb the mass shift at $d=2$ with $\delta_m$, can we justify the analytic continuation? I think $d=2$ and $d=4$ are two completely different and independent situation, how could we just analytically continue one to the other?

Answer 1

Probably there are some important details that I do not fully understand. But on a high-level, we want to do this because we don't want $\delta_m$ to depend on $M^2$.

If we did it directly in $d=4$, (12.32) would have a pole depending on $p^2$, and therefore we would have to make $\delta_m$ depend on $M^2$. This would mess up all the following derivations.