0
$\begingroup$

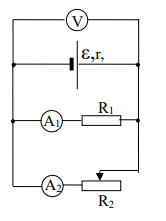
Question

Diagram

The diagram in question has no numerical values.

  1. $R_1$ is a resistor of constant resistance. $R_2$ is a variable resistor set to minimal resistance.
  2. $V$ is an ideal voltmeter. $A_1$ & $A_2$ are ideal ammeters following each equivalent resistor.
  3. emf $\varepsilon$ has internal resistance $r$.

The variable resistor is being set to maximum resistance. Which of the following is true?

  1. Ammeter $A_1$ would read lower current than before, Ammeter $A_2$ would read higher current than before.
  2. Ammeter $A_2$ would read lower current than before, Ammeter $A_1$ would read higher current than before.
  3. The voltmeter would read lower voltage.
  4. Both Ammeters $A_1$ and $A_2$ would read lower current than before.

I was given this question and I suspect, after using made-up numerical values for each component, that the answers are incorrect, question is not phrased well, or rather the question is missing details.
Generally speaking, before delving into each answer, as long as the resistance of $R_2$ increases, the current in the circuit decreases, whilst the voltage of the circuit increases.
What I think of each answer is:

  1. The current in the circuit decreases, while the resistance of $R_2$ increases, so less current would go through $A_2$ than originally. Can't be.
  2. Not sure if its always true, but I found that it's true when the minimal and maximal resistance of $R_2$ is greater than that of $R_1$. But not if the min/max of $R_2$ is lower than that of $R_1$. So it can't be.
  3. The voltage of the circuit increases when the current decreases. Can't be.
  4. Since answer 2 is true in some cases, this can't be true.

Am I missing something?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

Am I missing something?

Hint: It appears you are missing the effect of the voltage drop across the battery internal resistance $r$ when resistor $R_2$ is varied.

Hope this helps.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Wouldn't the only effect be the one I wrote - Which is that when R2 is minimal the voltage drop on the internal resistor is greater (higher current), and the other way around when R2 is maximal? $\endgroup$
    – Nimrod M
    Commented May 20, 2022 at 14:12
  • $\begingroup$ Coming to think about it again, it may be that because the voltage is greater when R2 is maximal, R1 has to drop greater voltage, and because it is a constant resistor, I=V/R1 is greater. Hence answer 2 is correct? (But only when compared to the R2, and not the situation before setting R2 to maximum resistance). $\endgroup$
    – Nimrod M
    Commented May 20, 2022 at 14:22
  • $\begingroup$ I agree wit Everything you said up to the last statement in parentheses, which I don’t understand. You shouldn’t try to “feel” your way through this. Apply KVL to loop containing battery and R2 and loop containing battery and R1 $\endgroup$
    – Bob D
    Commented May 20, 2022 at 15:01
  • $\begingroup$ Indeed KVL further proves the point: I=emf/(R2 + r), when R2 is greater, so the current is lower. Looks like I had some trouble with my calculations and the understanding of KVL. Thank you. $\endgroup$
    – Nimrod M
    Commented May 20, 2022 at 15:57
  • $\begingroup$ It’s always better when you figure it out yourself $\endgroup$
    – Bob D
    Commented May 20, 2022 at 16:03

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.