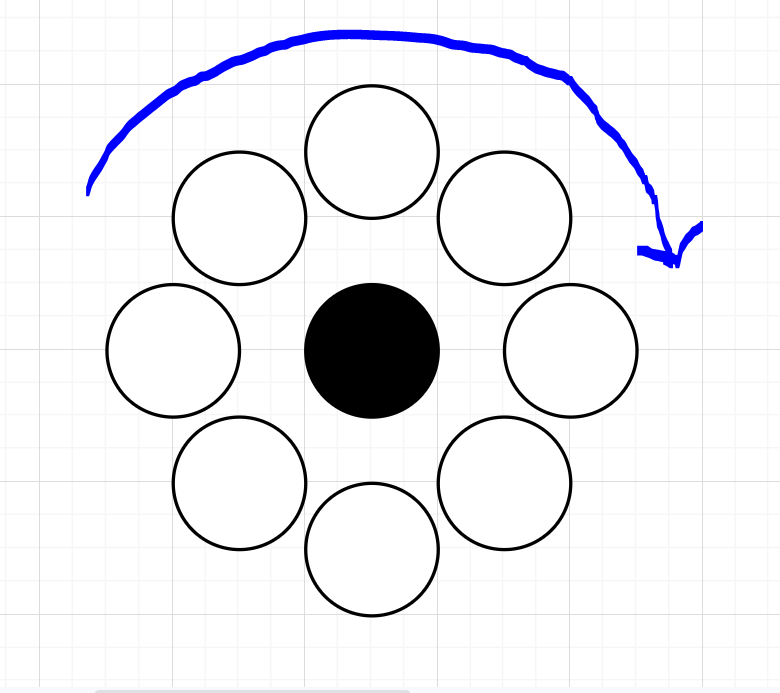
Consider this setup:
The black circle is the observer, who has a $360^{\circ}$ range of observation around it. In its orbit are other circles, orbiting at relativistic speeds. They are all orbiting at the same radius away from the center (the observer). From the stationary observer's perspective, the distance between any two orbiting circles should be contracted. Since the observer sees all the circles, all the distances decrease. That means that the radius of the entire orbit should decrease as well from the observer's frame of reference, no? If not, how can all of the circles be closer together from that frame?
Consider a similar (perhaps fundamentally identical?) paradox that is a little less abstract. You have a circuit of moving electrons that go in a circle around the observer. This observer again is able to observe all electrons. Within any one slice of the wire, the electron density increases due to length contraction. If it increases all throughout the wire, from the observer's frame, then surely the observer's frame is observing more electrons than are present in the electrons' frame of reference? That too seems utterly paradoxical.