Consider an upwardly accelerated elevator as in the figure. From outside the elevator, an inertial observer (unseen in the figure) shoots light perpendicular to the moving direction of the elevator. The width of the elevator is $\Delta x$ for the inertial observer. The inertial observer measures the light traversing time $\Delta t$. Thus, $c = {\Delta x / \Delta t}$. For the observer inside the elevator the path of the light is bent and its arclength is $\Delta s' > \Delta x$. He/she measures the traversing time $\Delta t'$. Thus $c'= \Delta s'/ \Delta t'$. Is it true that $c = c'$? If so (probably an axiom), $\Delta t' > \Delta t$. As far as I have learned, an accelerated frame is equivalent to a frame in a gravitational field and there is a time dilation in a gravitational field. Then, it should be that $\Delta t' < \Delta t$. Thus, the two inequalities contradict to each other. Should we discard the axiom $c=c'$ or is there something I misunderstand? Your illumination will be appreciated. 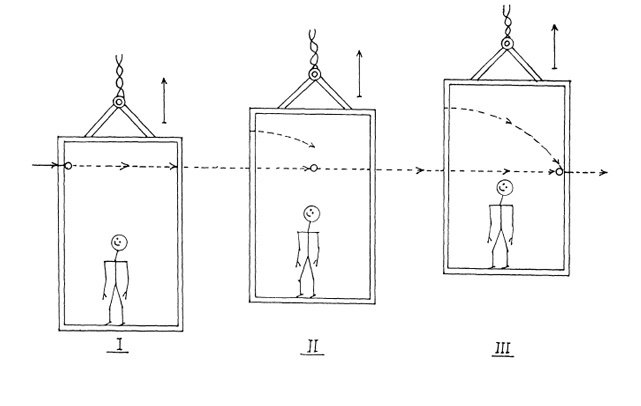
$\begingroup$
$\endgroup$
2
-
$\begingroup$ See physics.stackexchange.com/q/399235/123208 physics.stackexchange.com/q/399738/123208 & en.wikipedia.org/wiki/Rindler_coordinates $\endgroup$– PM 2RingCommented Mar 20, 2022 at 9:22
-
$\begingroup$ You've discovered that $c=c'$ doesn't work in a non-inertial frame. $\endgroup$– PM 2RingCommented Mar 20, 2022 at 9:26
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
The speed of light is always measured to be $c$ locally in an inertial frame.
The inside of the lift isn't an inertial frame due to its acceleration, which is large enough to see/measure the non-inertial effects.
