Negative-frequency waves

Question

Let's consider a plane wave described by $E = E_0\exp(i(kx-\omega t))$. We can either consider this wave to move forward (i.e. along increasing distance $x$) with a positive angular frequency $\omega > 0$, or consider it to move backward with a negative angular frequency $\omega < 0$. We usually say that the second choice is unreal and stick with the first one. However, what if we can no longer choose ?

Consider one beam of light in an optical fiber back-reflected by an imprinted grating (i.e. small oscillation of the refractive index in the fiber), that we consider here as a standing wave.

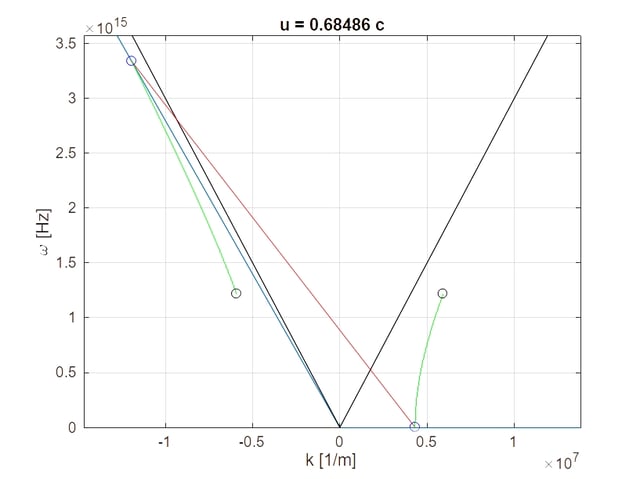
The forward- and backward- propagating beams of light move at a velocity $\pm c/n$, where $n$ is the glass's refractive index. These three waves are drawn in the following $\omega-k$ diagram:

The black lines indicate the dispersion relations of waves propagating in vacuum at a velocity $c$ for reference only, while the blue lines represent the dispersion of the waves propagating at a velocity $c/n$ in our fiber. The two blue circles indicate the $\omega$ and $k$ of the two optical waves and are connected with the red line, representing the grating. The three waves being connected in this diagram indicates energy and momentum conservation.

Now, let's start observing these waves while moving at a velocity $u$ along the direction of the fiber, where $0 < u < c/n$. Applying Lorentz transformations to the three waves, we obtain the following modified diagram:

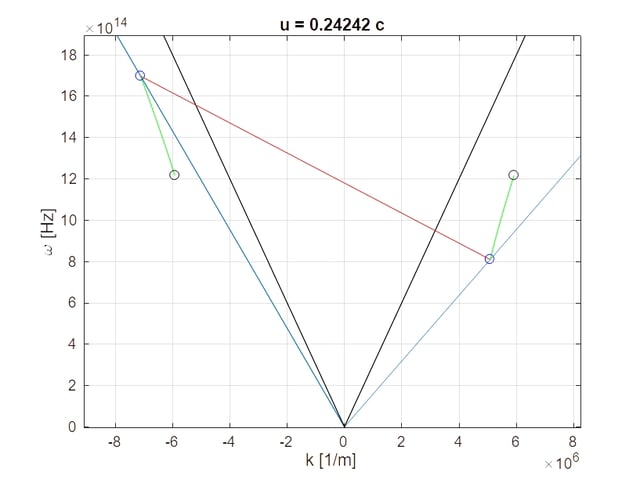
The green curves in this diagram only indicate the path that the two blue circle follow when gradually increasing the velocity $u$ from $0$. We see that now our grating has a velocity with respect to us, and remains connected to the two waves, so momentum and energy are still conserved. Let's increase our velocity to $u = c/n$:

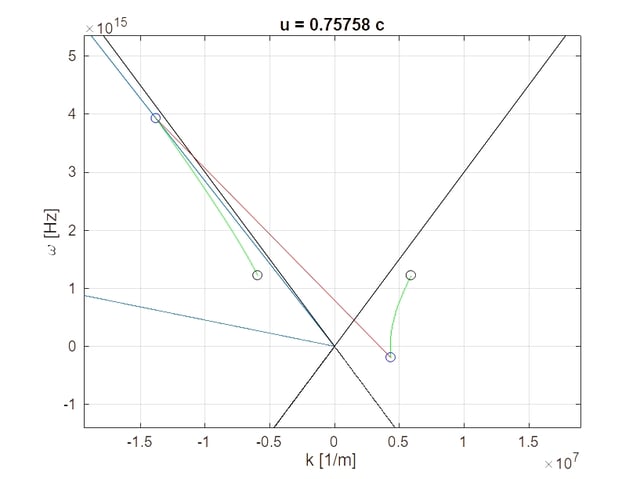
Here, we travel at the same velocity as the forward-propagating wave, so it now looks like a standing wave to us ($\omega = 0$). The waves remain connected, indicating momentum and energy conservation. Let's increase our velocity to $u > c/n$:

Now comes the interesting part. Normally we would see the initially forward-propagating wave propagating backwards since we move at a larger velocity than it (i.e. along the small-slope blue line), but momentum and energy conservation forces us to see this wave as a forward-propagating, negative $\omega$ wave, because this solution connects the three waves, indicating energy and momentum conservation, i.e. the red line, which has its length and direction given by Lorentz transformation, can only connect the backward-propagating wave (blue circle on the $k < 0$ side, also given by Lorentz transformation) to the blue circle on the $k> 0$ side, but cannot connect any dot on the small-slope blue line, corresponding to the $k < 0$ solution. So, by choosing $\omega > 0$ and $k < 0$, we would break energy and momentum conservation, and the only solution to conserve energy and momentum is to see this wave as having a negative angular frequency $\omega < 0$ and a positive $k > 0$. Would that be a way to observe negative-energy photons ? If not, how is this situation solved ?

Answer 1

I am not sure if this would answer your question. Because I didn't try to solve your example, including Lorentz transformation, energy and momentum conservation, photon energy etc.

But to me, there are two issues to be addressed. First one, to me, is (-k,+w) and (k,-w) are identical, if we think about how to express a plane wave.

For either mechanical wave or EM wave, we are talking about the vibrations with space x and time t. In principle vibrations are real numbers, so it is $E=E_0cos(kx-wt)$, and you can choose to keep $w$ positive and use $k$ to control the direction. But since complex numbers are more convenient (and since wave equations are linear), so we can use complex number $E=E_0e^{i(kx-wt)}$ for calculations and use the real component for physical interpretations.

So if the real component is concerned, $E=E_0e^{i(-kx+wt)}$ is identical to $E=E_0e^{i(kx-wt)}$

Or another way to see this is $E_0cos(kx-wt) = \frac{1}{2}(E_0e^{(kx-wt)} + E_0e^{(-kx+wt)})$. In complex expression, whether you have obtained positive omega part or negative omega part, you always have to add its complex conjugate to obtain the real component.

The second issue, which I am not sure in your case, is that do you need a negative energy photon to have your energy conserved? If so, then I guess somewhere breaks down when you have your observer's speed faster than the light speed in fiber?