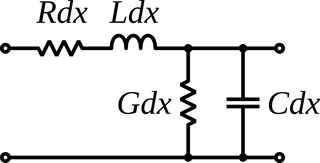0
$\begingroup$

In a transmission line consisting of 2 parallel wires, one can show that the voltage and current exist as a wave propagating along the wire.

When calculating, for example, the reflection coefficient due to an unmatched load, one uses the waveforms $$V_0e^{i(\omega t - kz)}$$ $$I_0e^{i(\omega t - kz)}$$ to derive the result. However, why do we not use $$E_0e^{i(\omega t - kz)}$$ $$H_0e^{i(\omega t - kz)}$$ (representing the propagating E and H fields) for this derivation?

Essentially what I am unsure of is the difference between the propagating voltage and current waves with the propagating EM waves in a transmission line. Are they one of the same thing?

Any help will be much appreciated.

Improve this question
$\endgroup$
1

2 Answers 2

Reset to default
1
$\begingroup$

It is a matter of convenience: voltage and current in the wires are related to the electric and magnetic fields via the Maxwell equations - we can, find ones once we know the others. When we deal with a rectangular or cylindrical wave guide, dealing with electric and magnetic field is preferable, since the there is no clear way to define voltage and current in this case - e.g., voltage is measured between two points, and the choice of these two points in a cross-section of the wave-guide is somewhat arbitrary. For two parallel wires, voltage is straightforwardly defined as the potential difference between the two wires at the specified cross-section, whereas the electric and magnetic fields have a rather complex structure in the space between the wires.

Update
Current and voltage in a transmission line can be viewed in terms of Telegrapher's equations, where voltage stands as a proxy for the electric field (in the effective per-length capacitance), whereas the current is a proxy for the magnetic flux (in the effective per-length inductance).
enter image description here
(image source)

In either case, describing oscillations/waves requires two conjugate variables, because oscillator/wave equations are second order equations. For a harmonic oscillator these are position and momentum, for EM wave these are the electric and the magnetic field, whereas for a transmission line these are voltage and current. As previous paragraph shows, voltage and current can be related to the electric and magnetic fields, but used here as more practical variables for circuit engineering (see also Lumped-element model.)

Improve this answer
$\endgroup$
0
$\begingroup$

The E field and the voltage are the same thing. The current is its own thing, it depends on the impedance of the line and the voltage or E. The magnetic field is its own thing but depends on the current.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Thank you for the response, but then why is it that when we calculate the reflection coefficient that we use the voltage and current wave instead? $\endgroup$
    – Student 1
    Commented Nov 18, 2018 at 12:39
  • $\begingroup$ Usually when calculating reflection coefficient we are concerned with efficiency, or how much energy or power is transferred or reflected, so V and I are convenient because impedance is considered to get I and P=VI. $\endgroup$
    – PhysicsDave
    Commented Nov 18, 2018 at 12:45
  • $\begingroup$ I see, and I guess even if we were to use H instead of I then we just multiply everything by a constant so they would cancel anyway? $\endgroup$
    – Student 1
    Commented Nov 18, 2018 at 12:49
  • $\begingroup$ Impedance can be complex, so getting I from V is the key. I don't remember all the details, but H is proportional to rate of change of current. $\endgroup$
    – PhysicsDave
    Commented Nov 18, 2018 at 12:52

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.