What is the interpretation of the symmetric stress tensor in laminar flow?

Question

The strain rate tensor $D_{ij}$ is defined as

$$ D_{ij} = \frac{1}{2}\left(\frac{\partial v_i}{\partial x_j} + \frac{\partial v_j}{\partial x_i} \right) $$

For a Newtonian fluid the stress tensor $\sigma_{ij}$ is related to to strain rate tensor by

$$ \sigma_{ij} = 2\mu D_{ij} $$

where $\mu$ is the viscosity.

Say we have a flow $\mathbf{v} = [v_x(y), 0, 0]$, i.e. only flow in the $x$-direction with only variation in the $y$-direction. The only non-zero components of the stress tensor are then

$$ \sigma_{xy} = \sigma_{yx} = \mu\frac{\partial v_x}{\partial y} $$

$\sigma_{yx}$ is easily interpreted as the friction force due to viscosity in the $x$-direction acting on surfaces with unit normal in the $y$-direction.

But what is the physical origin/interpretation of $\sigma_{xy}$, that is, a force acting in the $y$-direction on a surface with unit normal in the $x$-direction? Is it a reaction force to the tendency of the friction forces to rotate a fluid element?

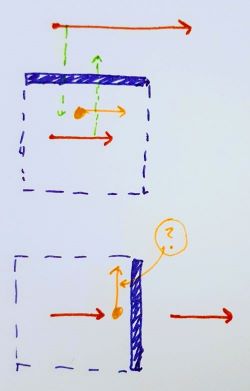
An attempt to make it visual:

In the upper image, it seems reasonable that there is a momentum transfer (green arrow) across the thick blue surface, which results in a non-zero net rate of change of momentum/force (orange arrow) since the fluid velocities (red) are different on each side of the surface.

However, in the lower image I can't see the physical/microscopic origin of the (orange arrow) shear force (which must be there since the stress tensor is symmetric), since the velocities are the same and hence there is no net transfer of momentum across the (thick blue) surface.

Answer 1

The strain rate tensor actually comes from decomposing the velocity gradient tensor i.e. $$\frac{\partial u_i}{\partial x_j}=\frac{1}{2}(\frac{\partial u_i}{\partial x_j}+\frac{\partial u_j}{\partial x_i})+\frac{1}{2}(\frac{\partial u_i}{\partial x_j}-\frac{\partial u_j}{\partial x_i})$$

where on the Left Hand Side, former is the symmetric part and the latter is the antisymmetric part. It is similar to writing a matrix as the sum of a symmetric matrix and skew symmetric matrix.

If you don't understand decomposition of tensors then simply think of the above equation as writing A= 1/2(A+B) + 1/2(A- B).

Now if would have gone through the derivation of the rate of angular deformation of a 2-D fluid element then you will notice that,$$\frac{\partial u_i}{\partial x_j}+\frac{\partial u_j}{\partial x_i}$$is the rate of angular deformation of the fluid element and,$$\frac{1}{2}(\frac{\partial u_i}{\partial x_j}-\frac{\partial u_j}{\partial x_i})$$is the angular velocity of the fluid element. In solid objects, stress is the function of Modulus of elasticity and the strain in the element.

Now, stress is the function of velocity gradient tensor as a whole and not only the symmetric part as you've written above. What you've written is correct only if we impose a constraint on the fluid element that the Moment of the shearing forces about the centre of the fluid element is zero. This condition causes the rotational velocity of the fluid element to become zero and therefore we can write the shear stress as the function of symmetric part only.

Assuming your case in which there is x-direction translation of the fluid element, let's say there is a no slip condition at the lower surface such that x dir. velocity is zero. Moment about the centre(M) $$M= \sigma_{yx}.(lb)h-\sigma_{xy}.(bh)l$$ where lb is area of the face on which sigma yx is acting and h is distance between the the opp. faces and respectively for sigma xy. So if M is zero we get $$ \sigma_{yx}.(lb)h=\sigma_{xy}.(bh)l$$

And therefore the $$\sigma_{xy}$$ is a result of maintaining rotational equilibrium.

For more details watch this lecture.

Note:- In continuum mechanics, the stress in the fluid element is the function of viscosity coefficient and the rate of deformation (angular + linear).

Answer 2

In terms of tensors: $$ dF_i = \sigma_{ij}[\mathbf{\hat{u}}]_j dS \Longrightarrow F_i = \iint_{S} \sigma_{ij}[\mathbf{\hat{u}}]_j dS = \iint_{S} \sigma_{ij} dS_j $$ that is, a surface force can be described by its orthogonal components, each given by the contraction of stress. In this contraction one component of stress will be a free index $i$ and another, the contracted one, will give contributions to every direction $j$ (including, but not limited to, your given direction $i$).

If $v_i$ and $\sigma_{ij}$ are such as you have given, then the above definition reduces to: $$ F_x = \iint_{S} \sigma_{xy} dS $$ that is, shearing force ($x$ direction) integrated over the surface area (which points to the $y$ direction in flat plates where such equations are usually derived, that is, $dS_y = [\mathbf{\hat{u}}]_y dS = \mathbf{\hat{u}} \cdot \mathbf{\hat{e}_y} dS = dS$ since then $\mathbf{\hat{u}} = \mathbf{\hat{e}_y}$).

In the end, it's just $\text{shear stress} \times \text{area}$. You might argue that the stress tensor is just encoding how each force component behaves, per infinitesimal and directed area (think of d$\mathbf{S} = \mathbf{\hat{n}} dS$).