It is widely known that placing a converging lens in front of a collimated light source focuses all light into a point @ $d=F$, and that the inverse occurs: a point source at the focal length of a converging lens collimates light into parallel rays.
Shifting the light source from the focal point (defocusing the optical system) causes those rays to diverge.
What equation would give me the resulting angle $\theta$ given $F$ (focal length) and $d$ (distance between source and lens)?
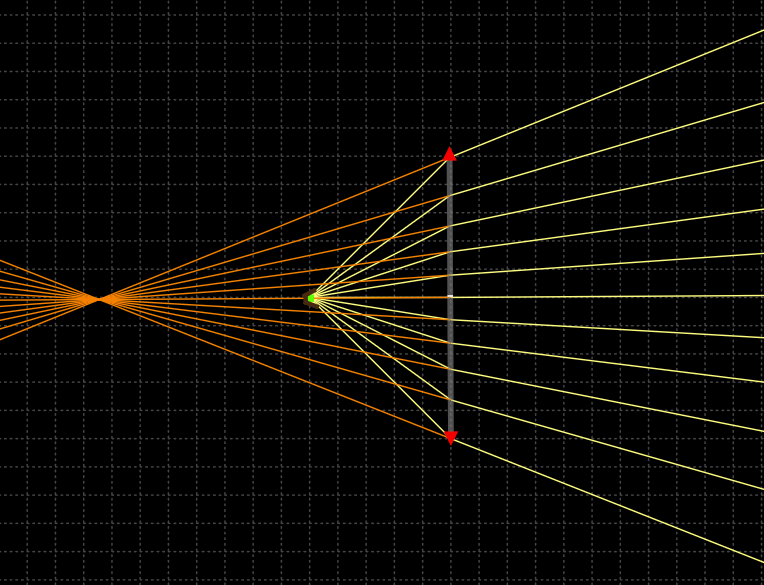
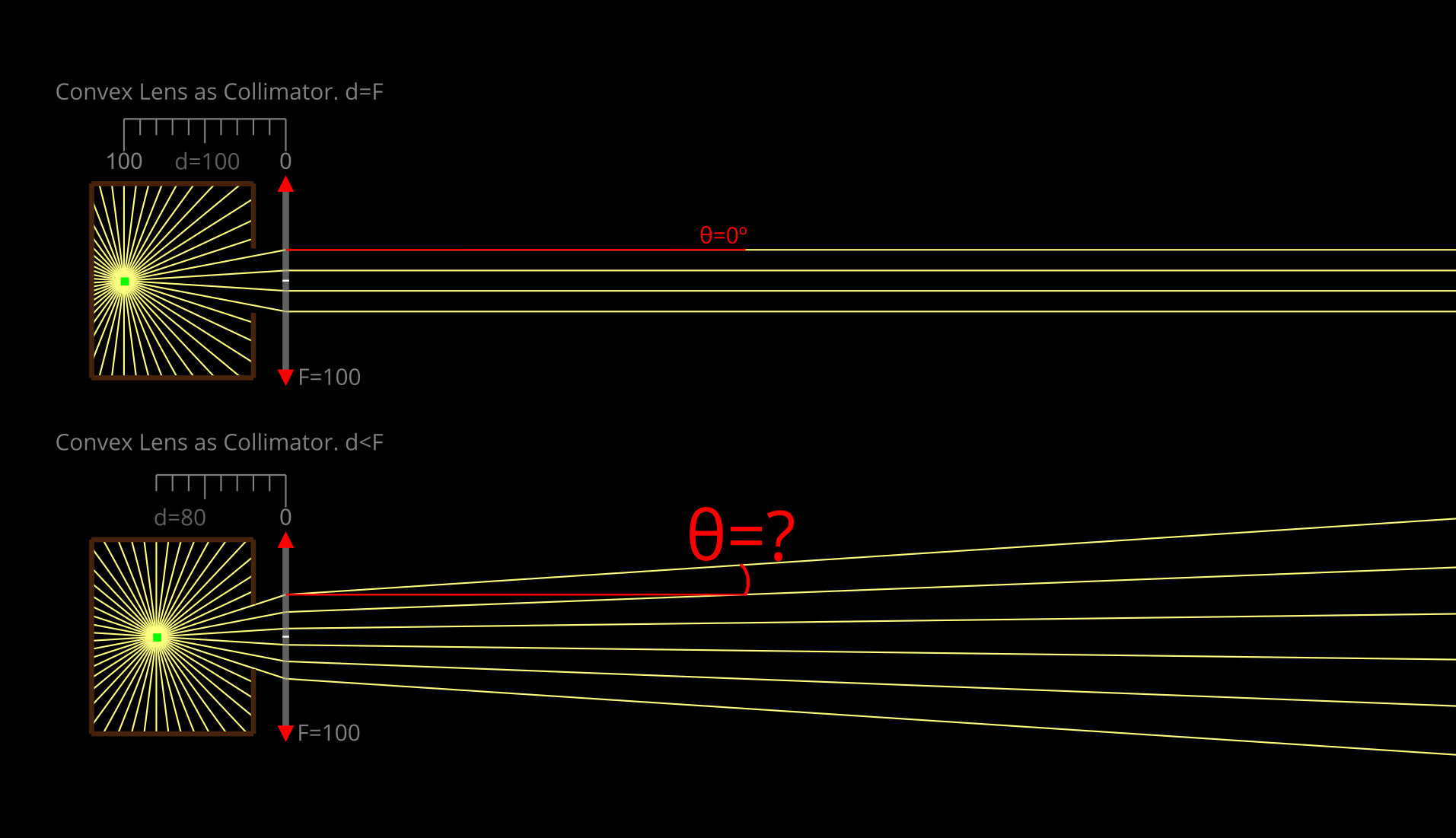
You can calculate first the formed image by using the lens equation:
$${1/p + 1/q = 1/f}$$
In your case $q = (1/f-1/p)^{-1}$ with $f=100$ and $p=80$ we solve the equation and get $q=-400$. The negative sign reflects that the image is virtual and on the left side.
From here we will assume $p$ is smaller than $f$ so the image is virtual, but similar concepts will apply with a positive $q$ and a real image.
Once you know where is the virtual image you can calculate the angle using trigonometry. At a given height of the lens $y$ with respect to the optical axis andthe absolute value of the distance to the virtual image $x$ . We can calculate the angle with the following equation: $${\theta} = arctg(y/x)$$
For instance, in your case we have $x= 400$ so in the optical axis $y=0$ and then ${\theta}=0$, which is the expected solution and for a height equal to the distance to the virtual image $y = 400$ we get the $arctg(400/400)= π/4$ or $45°$ which also makes sense.
Finally a more general equation for this system and the given information would look like:
$${\theta} = arctg(y/p-y/f)$$
Note that we inverted the sign inside the arctg as $ x = abs(q)$, as we implied that $p$ is smaller than $f$
