It is the difference between bound states and free particles. A bound state is a solution of a quantum mechanical equation, the wave function $Ψ$ a complex function and $Ψ^*Ψ$ is the probability of finding the electron about the nucleus at a specific (x,y,z,t). The electrons are in orbitals, not orbits, which is the naive "cloud" description. See Quantum microscope’ peers into the hydrogen atom.
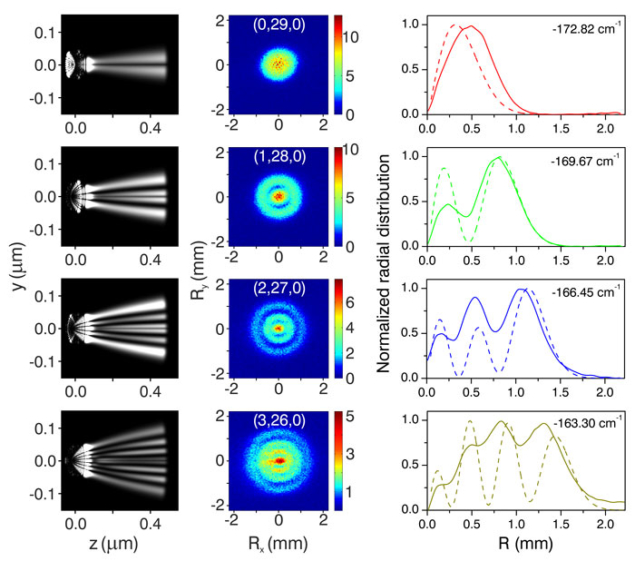
What lies within the H atom? Experimental observation of the transverse nodal structure of four atomic hydrogen Stark states. The images in the middle show experimental measurements.
And here is an electron, free, leaving an interaction vertex:
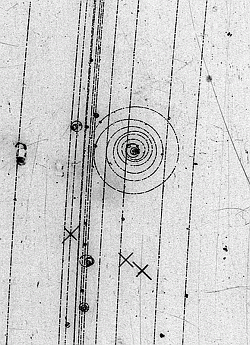
The curly line was produced by an electron that was struck by one of twelve $K^-$ passing beam particles in a liquid hydrogen bubble chamber. It curves in an applied magnetic field and loses energy rapidly, spiralling inwards.
Its energy and momentum can be solved with the classical equation of a charged particle in a magnetic field losing energy due to the medium it interacts with. So it is a trajectory in the classical sense.The tracks of the beam particles are also a trajectory in the classical sense.



