Gravitational field strength and Horizon in Rindler coordinates

Question

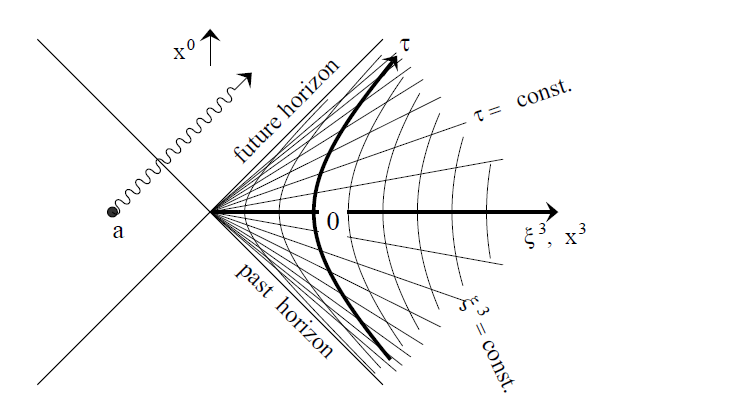
I came across the following statements in 't Hooft's black holes notes, but not being able to justify them.

The metric in the Rindler coordinates $x=\tilde{x}, y=\tilde{y}, z= \rho \cosh{\tau}, t= \rho \sinh{\tau}$ is $$ds^2 = -\rho^2 dt^2 + d\rho^2 + d\tilde{x}^2+d\tilde{y}^2$$

1. Gravitational Field Strength

The actual gravitational Field strength felt by the (Rindler)observer is inversely proportional to the distance from the origin.

How can I see this from the metric? How do I mathematically and physically justify this claim?

2. Horizon

Why does the surface $x=t$, act like a Horizon i.e. anything from outside the shaded area shown in the picture can't enter into the shaded region? I think, this can be seen by showing that all time-like curves starting from a point outside the shaded area don't enter the shaded region. But what are all the time-like curves in the Minkowski space-time? and would this assumption still hold for any arbitrary geometry which is locally like the Rindler metric(e.g. the schwarzchild metric near the horizon?)

Answer 1

1)Gravitational Field Strength

You have to consider the analogy with a uniform accelerated move, in special relativity, that is :

$z' = \frac{1}{a} ch (a \tau'), t' = \frac{1}{a} sh (a \tau')$

Here $a$ is the acceleration and $\tau'$ is the proper time.

You get : $dz'^2 - dt'^2 = -d\tau'^2$,as wished.

Now, make the coordinate change $\tau = a \tau'$, we get :

$z' = \frac{1}{a} ch (\tau), t' = \frac{1}{a} sh (\tau)$

$dz'^2 - dt'^2 = - \frac{1}{a^2} d\tau^2$.

But this is the same result that the Rindler metric with $d\rho=d \tilde x =d \tilde y = 0$, so we make the identification between $\rho$: and $\frac{1}{a}$, so the gravity strengh (acceleration) is inversely proprotionnal to the space-like quantity $\rho$

2) Horizon

I am not sure to understand all your questions, but you have to be careful about several points :

The Minkowski metric of the form : $-dt^2 + dz^2 + d \tilde x^2 + d \tilde y^2$, linked to the Rindler metric, by the transformations $z= \rho \cosh{\tau}, t= \rho \sinh{\tau}$, is only correct :

• near the horizon
• for a small angular region, that could be considered centered at $\theta = 0$

The horizon itself is not a 3 dimensional-surface in space-time, because the horizon corresponds to $\rho=0$, that is $t=z=0$.

This is because the metrics $g_{00} = 0$ at the horizon, so the horizon has no extension in the time coordinates.

So, considering that the horizon is $z^2 - t^2 = 0$ is not correct, the horizon is $t=z=0$.

A correct global representation is then using Kruskal–Szekeres coordinates, which gives a correct global point of view.