Why isn't a pseudo force considered for a block on an accelerating block?

Question

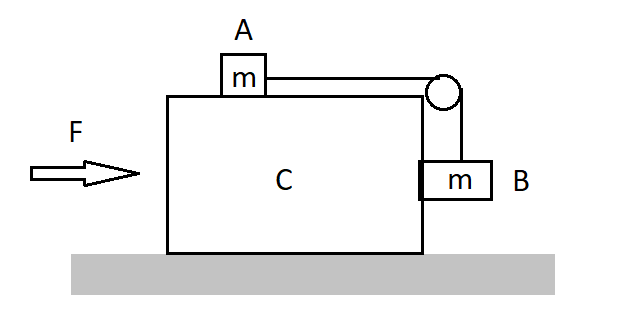
I was going through an example where there is a system of blocks where two blocks A and B of mass $m$ are attached through a pully on another block C of mass $M$. The friction between the blocks is $\mu$, and the objective is to calculate the minimum or maximum force to keep the smaller blocks at rest with respect to C.

In the example, when A, B, and C are taken as a system, the total acceleration is said to be $\frac{F}{M + 2m}$, which I understand.

However, when A alone is taken as the system, the only forces considered are the tension $T$ towards the right, the frictional force $f$ towards the left, the weight $mg$ and the normal force $N$.

Why isn't a psuedo force opposite to F considered since we are in a non-inertial frame? When the forces on B are considered in the example, it accounts for a normal force $N'$ that acts towards the right because of the acceleration of the system caused by F, but I don't see it being a factor in the forces considered for block A as a system.

Also, the frictional force $f$ is taken to be $\mu mg$ and it states the horizontal net force is $T - \mu mg = ma$ since "the block moves towards the right with an acceleration a". Is this the same acceleration of the entire system caused by F? In that case, how is it being considered instead of the acceleration caused by block B attached to the rope?

I have not completely wrapped my head around the procedure for isolating and analyzing individual bodies in a system so I apologize if the question comes across as vague or convoluted.

Answer 1

$a$ is the acceleration of block A with respect to the reference frame $S$ in which the ground is at rest. We do not need to introduce a pseudo force because $S$ is an inertial frame. However, $a$ is not necessarily the same as the acceleration $\frac F {M+2m}$, which is the acceleration of the centre of mass of the whole system. We can only say that $a = \frac F {M+2m}$ if block A does not slip on block C.

If we work in a reference frame $S'$ in which block A is at rest, then $S'$ is accelerating to the right with acceleration $a$, so we need to include a horizontal pseudo force $-ma$. If we assume $\mu$ is the coefficient of static friction and block A is on the point of slipping then the horizontal equation of motion for block A becomes

$T - \mu m g - ma = 0$

The right hand side is zero because block A is at rest in frame $S'$. But if we re-arrange this equation we get

$T - \mu m g = ma$

which is exactly the same as the horizontal equation of motion for block A in frame $S$ - which is what we expect.

Note that block A is not moving vertically, so its vertical equation of motion (in both frame $S$ and in frame $S'$) is

$N - mg = 0$

Answer 2

When you analyze a system using $F=ma$ and $a$ is non-zero, that doesn't mean it is not an inertial frame. You are analyzing the motion from the "laboratory frame" attached to the floor, which is inertial. 95% of Newtonian mechanics problems are analyzed in this way.

You are looking for a pseudo-force to "account for" the acceleration of the body. There is none. The equation already has an $ma$ term that accounts for it. The forces do not sum to zero, and there is a net acceleration caused by the unbalanced force. E.g. the normal force $N'$ on $B$ is simply equal to $F$ in your example, and the x-acceleration is given by $F=ma_{B,x}$.

If we were analyzing the system in a non-inertial frame that accelerated with the blocks, in that frame $a$ would in fact equal zero:

$$\sum F =m*(0)=0$$

and to make the equation balance, in this case we would need a pseudo-force $F'$ to counteract the applied force $F$:

$$\sum F = F+F' = m*(0)=0$$