Minimum force necessary to keep block from slipping in a two-block system

Question

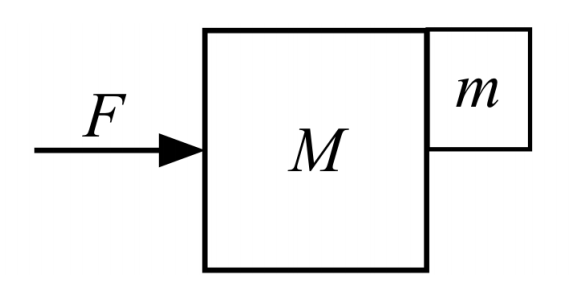
Consider the following system of two blocks, with the larger block having mass, $M$ and the smaller block $m$.

I want to find the minimum force such that the smaller box does not slip as the two block system accelerates. My approach is as follows.

We know that the force of gravity acting on the smaller mass is $mg$. Therefore, the force of friction must counteract this force, such that $mg=\mu F_{Mm}$, where $F_{Mm}$ is the normal force parallel to the surface along which the blocks are moving. However, $F_{Mm}=F=(M+m)a$ for the two block system. This leaves us with, $\mu(M+m)a=mg\rightarrow a=\frac{mg}{\mu(M+m)}$. Consequently, the minimum force required is given by $F=ma=(m+M)\frac{mg}{\mu(m+M)}=\frac{mg}{\mu}$.

To be entirely honest, I'm not sure if my formulation/understanding is correct. If it is, then my second question would be, is the coefficient of friction the coefficient of static friction or the coefficient of kinetic friction? I think it is static friction because the block would be motionless. Is the coefficient of kinetic friction only necessary when the acceleration is below the required minimum acceleration for no slipping to occur?

Answer 1

$mg=\mu N$ is correct, where $N$ is the normal force between the 2 blocks - ie horizontal. $\mu N$ is the friction force parallel to the contact surface - ie vertical.

The force $F=(m+M)a$ is accelerating the 2 blocks. $N=ma$ is the force accelerating the smaller block.

Although the 2 blocks are moving over the ground, there is no relative motion between them, so the friction between them is static.