The Wikipedia article on matter waves describes the dependency between phase velocity $v_p$ and group velocity $v$ as $$ v_p = \frac{c^2}{v} $$
So for group velocity $v=0$ the phase velocity is infinite? Is this a side effect of some simplification in the derivation? Or does this have some half-intuitive physical interpretation, like "electrons never rest", i.e. they never have $v=0$ for whatever reason?
This is a nice example of seeing what group velocity and phase velocity mean for classical waves :
Frequency dispersion in groups of gravity waves on the surface of deep water. The red square moves with the phase velocity, and the green circles propagate with the group velocity. In this deep-water case, the phase velocity is twice the group velocity. The red square overtakes two green circles when moving from the left to the right of the figure. New waves seem to emerge at the back of a wave group, grow in amplitude until they are at the center of the group, and vanish at the wave group front. For surface gravity waves, the water particle velocities are much smaller than the phase velocity, in most cases.
This wave is energy moving in space time.
The so called "matter waves" in the article are an exposition belonging to the days before quantum mechanics was established as the theory describing particles. In quantum mechanics the waves are probability waves. The wavefunction $Ψ$ of a single electron cannot be plotted as the figure above in space and time. One has to get a distribution of measurements of an electron, to measure the $Ψ^*Ψ$ distribution. The wave nature appears in experiments one electron at the time in the interference pattern seen in the accumulation.
Here is a description of the famous Tonomura double slit experiment one electron at a time
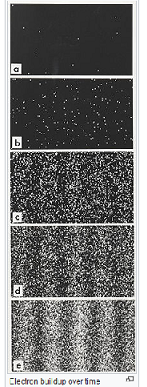
The accumulations of single hits from frame $a$ at frame $e$ show the wave nature of the accumulated electron footprints.
Group velocity for a single electron can be measured, but the phase velocity of the probability amplitude has no way of being measured for a single electron, and thus has no meaning in particle physics.
The Wiki article is mathematicaly correct, but rather misleading. There is no physical meaning to the phase velocity of a quantum particle. In the non-relativistic Shroedinger equation you can add any constant to to the potential $V$, and so change $E$ and hence $v_{phase}= E/p$, without doing anything physical. It is only $v_{group}=p/m$ that has any meaning and coincides with the classical velocity $p/m=mv_{class}/m=v_{class}$. In the relativistic case the physical velocity a particle with $p=0$ and rest energy $mc^2$ is $p/E=0$, not $E/p=\infty$.
