What Veritasium's video is describing is electromagnetic induction and EM power and signal wireless transmission. But the circuit he used in his thought experiment is wrong. Unless the voltage source is a 50-60Hz AC source and in the range of MVolts, a filament lamp or LED at 1m away from the voltage source will never light up! This path of electromagnetic energy Poynting vector is not possible or not sufficient to power up these lamps in this case described. The longer path, less resistive since he assumes in the beginning of the video that the cables have zero resistance (air is an insulator) will still need to be taken. Thus the a.c. electromagnetic field still needs 300,000 Km after the switch is closed, to travel and reach the lamp and start the oscillations of the electrons in the lamp that will start to emit visible light and heat.
So the correct ideal case answer (i.e. zero resistance wires but still a realistic small consumption incandescent lamp) for Ve's experiment setup and for an a.c. voltage source which can be turned ON/OFF for this type of lamps is 1s to actually light up and not (1/c)m thus ~3.3 ns at 1m as stated in the video. (Ve's video has too many unrealistic assumptions, zero resistance 300,000Km wires, incandescent lamp which lights up with infinitesimal small current! This circuit and specs he used is inappropriate to demonstrate the concept of wireless EM transmission of electric power).
On the other hand a fluorescent lamp with a few hundreds of KVolts a.c. voltage source will probably light up at 1 m distance and without being connected to any cables or switch.
https://www.youtube.com/watch?v=GoAPVYKqjQg
As for the case actually Ve tried to demonstrate of a d.c. voltage source and a transient signal caused by closing the switch in the circuit shown, in an ideal case scenario conditions as also described in the previous paragraph for a.c., using a realistic small consumption incandescent lamb, again, unless the voltage source is in the range of MVolts, X(L)=ωL, X(C)=1/(ωC) inductive and capacitive reactance is so large in this circuit that only a very small fraction of voltage drop is generated on the lamp by wireless or wired power transmission, not sufficient realistically to power any incandescent lamp. The power on the lamp would be completely dumped by the enormous total 600,000Km circuit loop inductance and 1m distance air dielectric tiny capacitance even assuming zero ohmic resistance wires were used. Therefore, the last choice in Ve video, thus nothing of the above and specifically the lamp will not light up is the most plausible result in this case.
As an indication, the inductance L of a 300,000Km loop is enormous about 8E9 H for a 2cm wire thickens and single loop and the distributed segment capacitance for 2cm thick parallel wires at 1m distance and air as a dielectric, is less than 3nF which is a tiny small capacitance, not sufficient to transfer the needed amount of charge to the lamp in order to light it up during the switch d.c. transient. There might be some objections at this point regarding the capacitive coupling of the transmission line demonstrated in this circuit of Ve, that a capacitor at the start of its charging behaves like a short-circuit? This is an abstract generalization and leads to misconception. Although, for very large capacitors this might be true in some extend, all capacitors have a small internal ohmic resistance R in series. Further, even if we accept Ve explanation of the capacitive coupling of the power transfer at 1m distance sufficient to light up the lamp, the realistic time will not be 1m/c~3.3ns but relative much larger, constraint by the RC time constant of the capacitive coupling where assuming for example a R=100 Ohms, ohmic resistance of the lamp and C=3nF capacitance between the two parallel wires at 1m distance, RC time delay is 330nS.
Even further, taking the Antenna transmission-reception argument for the 3.3ns signal transient period thus effectively an a.c. signal with ~λ=1m wavelength, this would result in an 3E8λ transmission antenna dipole! Meaning this kind of antenna would not transmit EM waves even at 1cm away.
In short, Ve's circuit used in his thought experiment is invalid and unsuitable IMO to begin with. Although, he has good intentions to demonstrate the wireless transfer of EM power. However, there are no lies told as he says in his video. EM fields prefer to travel always in the more conductive path or medium.
As for the specific question asked here, regarding violation of the speed limit of information transfer due to the position of the switch in the circuit, the relative position of the wire switch does not matter , because the potential difference is already transferred from the source battery at the open end leads of the switch before any attempt is taken to close this switch.
Update 1st Dec 2021:
Despite all the above mentioned and willing to adopt this time, all the conditions and assumptions of Veritasium's video thought experiment and totally ignoring any capacitive and inductive reactance delays and signal losses, I cannot take it out of my mind that the given explanation result in the Ve video is still not correct from a pure electrical-circuit theory point of view.
The question you have first to answer is, is EM field propagation in a conductive loop circuit the same as EM wave propagation from a transmitting antenna for example?
In the presented circuit thought experiment of Ve the battery is already connected to the conductive circuit prior the switch is closed. Therefore, the positive lead battery potential as shown, is already present along the whole circuit up to the switch right side connecting lead as well as the negative potential of the battery at the left side connecting lead of the open switch prior to the switch being closed.
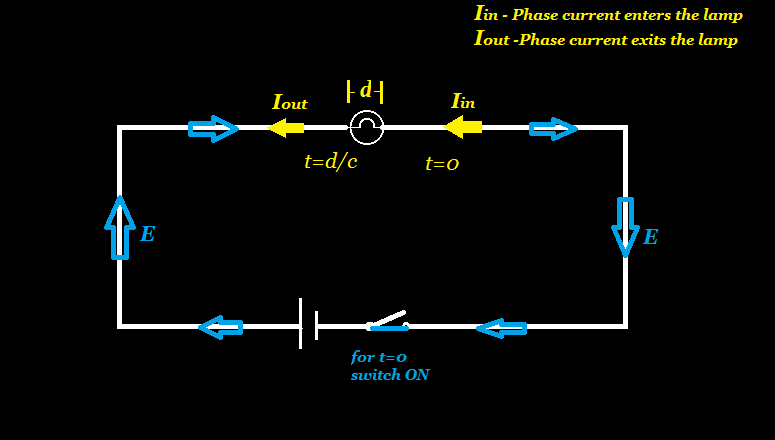
Note: Electron flow of current is used in the above schematic
Assuming our conductive circuit has ideally, inductance $L=0$ and capacitance $C=0$ and therefore $t=L/C=0$, as soon as the switch is brought in the closed position (assuming this is done instantaneous at time t=0 as shown in the figure), the potential difference is present at any segment of the circuit meaning an electric field vector E is generated simultaneously at any point of the circuit including the incandescent lamp which is actually a resistor connected in series in the circuit.
There is no time delay in this case described for the electric field to extend out from the positive lead of the battery and circulate along our circuit, as some would believe will hold also in this case described, confused with the EM waves radiation from an antenna and propagation of electromagnetic waves in space in general. The electric potential is applied as soon as the switch is closed and the d.c. transient signal due the switching is generated simultaneously at any point of the circuit shown.
There is no violation of any speed of information transfer limit c, since a potential is not energy although a potential difference between two connected points in a circuit can create an energy flow thus an electric current.
Therefore, the correct answer according to all technical circuit conditions and ideal assumptions described in Ve's video and herein is as following:
t=0, when assuming that the lamp lights up as soon as the phase current enters the lamp Iin. There is no time delay, the lamp will light up instantaneously.
t=d/c, time delay when assuming that the lamp lights up as soon as the phase current exits the lamp Iout.
Where d is the total length of the filament inside the lamp and c the speed of light.
Notice here, that in case 2 above assumption that the lamp would light up only if the phase current would pass through the total resistive path of the lamp, although the electric field is instantaneously applied to all points in the circuit as described, the force $E=qE$ on each charge still propagates form charge to charge at the speed of light c ideally assumed in our system or realistically speaking, at the speed of light inside the wire.
Conclusion: Non-intuitively, the speed of the conductive circuit path in Ve thought experiment with all the above assumptions explained herein, will be faster than the 1m air distance cap, path! Thus the lamp will start to light up continuously (i.e. steady state) at time less than 1m/c, thus t<3.3 ns. Meaning, in this scenario explained, the lamp would reach steady-state operation before the transient wireless EM pulse generated covers the 1m distance at ~1m/c time and reaches the lamp.


