Verifying conformal generators generate conformal transformations

Question

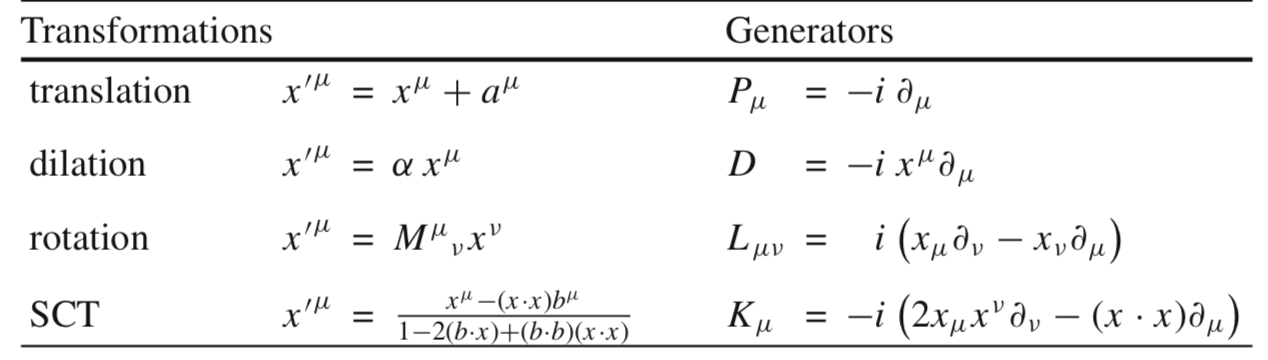
Given the finite conformal transformations

My simple, and really algebraric, question is, how do you actually compute $\text{exp}(ia^\mu P_\mu)$? What I have done: $$e^{a^\mu\partial_\mu}x^\nu=(1+a^\mu\partial_\mu+O(\partial^2))x^\nu=x^\nu+a^\mu\delta_\mu^\nu=x^\nu+a^\nu.$$ So far so good. $$e^{i\alpha D}x^\nu=(1+\alpha x^\mu\partial_\mu+O(\partial^2))x^\nu=x^\nu+\alpha x^\nu=(1+\alpha)x^\nu.$$ Is it correct that there doesn't need to be a 1-1 correspondence between generators and transformations? Like $e^{\alpha D}x^\mu$ does not generate $\alpha x^\mu$.

Answer 1

Read up on the Lagrange shift operator, essentially a formal summary of the Taylor expansion. You know its result given your summary of translations.

For just one variable, you found $$ e^{a\partial_x} f(x) = f(x+a). $$

Now note for $y\equiv \ln x$, $$ e^{ax \partial_x} f(x) ~~~\leadsto \\ e^{a \partial_y} f(e^y) =f(e^{y+a})=f(e^a~ x), $$ so your $\alpha=e^a$.

You can take it from here, appreciating D is scaling only the rotationally invariant "radius" of $x^\mu$.