0
$\begingroup$

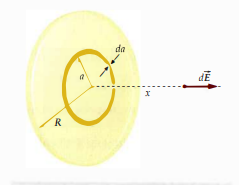
When trying to find the electric field created by a uniformly charged disc at a point P on axis of the disc, it can be done by integration.

enter image description here

We start by finding the electric field dE created by each infinitesimal disc of thickness da, as shown in the picture.

The area of each infinitesimal ring is said to be 2pia*da.

Shouldn't it be pi*(a+da)^2 -pi*a^2? Because it should be the area of the large ring of radius (a+da) minus the smaller ring inside of radius a to find the area of just the ring thickness da?

I suspect it's something to do with the expansion of what I believe it should be, because I'm not exactly sure what (da)^2 would equal? Is this the error?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Please change your post to use mathjax to display math $\endgroup$
    – Prof. Legolasov
    Commented Sep 23, 2021 at 8:35
  • $\begingroup$ Imagine using $\Delta a$ instead for a moment and comparing it to $(\Delta a)^2$ as $\Delta a \to 0$. For example what if $\Delta a \sim 10^{-10}$? Then remember that differentials are infinitesimally small in comparison. $\endgroup$
    – Triatticus
    Commented Sep 23, 2021 at 8:39

1 Answer 1

Reset to default
1
$\begingroup$

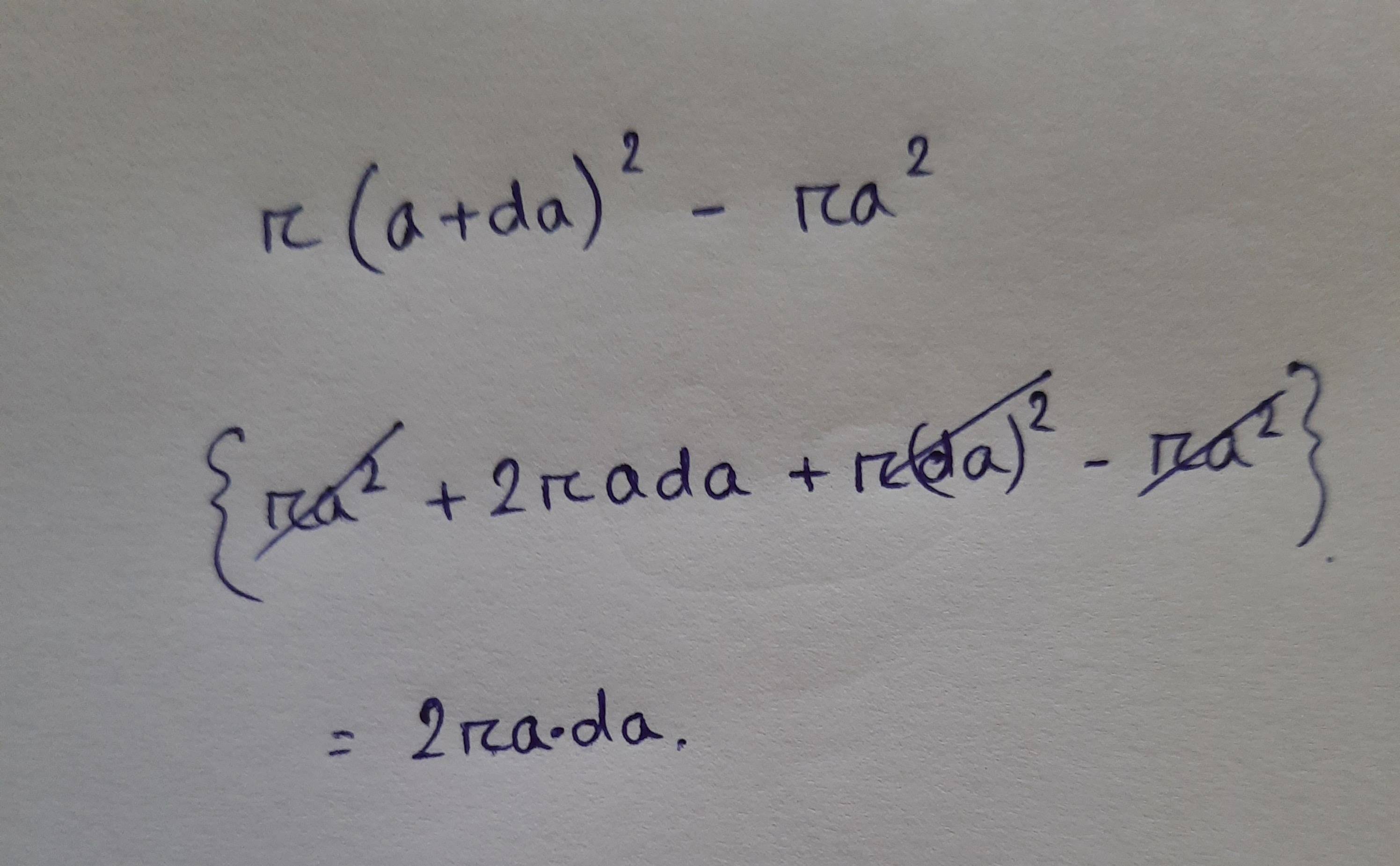Yes, see the image below enter image description here Here,π(da)² is eliminated because (da) is a very small element and it's square is even smaller and tends to 0.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Please use MathJax for formatting equations and variables. Images are not accessible to all users. $\endgroup$
    – BioPhysicist
    Commented Sep 23, 2021 at 11:27
  • $\begingroup$ Even if (da)^2 is very small, shouldn't it strictly still be used in the integral since we are dealing with infinitesimal values anyway? $\endgroup$
    – XXb8
    Commented Sep 24, 2021 at 7:23
  • 1
    $\begingroup$ da or $\Delta$a is a very small value , suppose something like $10^{-10}$, square of which is even small => $10^{-20}$ which you can assume 0. $\endgroup$
    – The Space Guy
    Commented Sep 24, 2021 at 7:34

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.