How to understand the concept of vanishing point?

Question

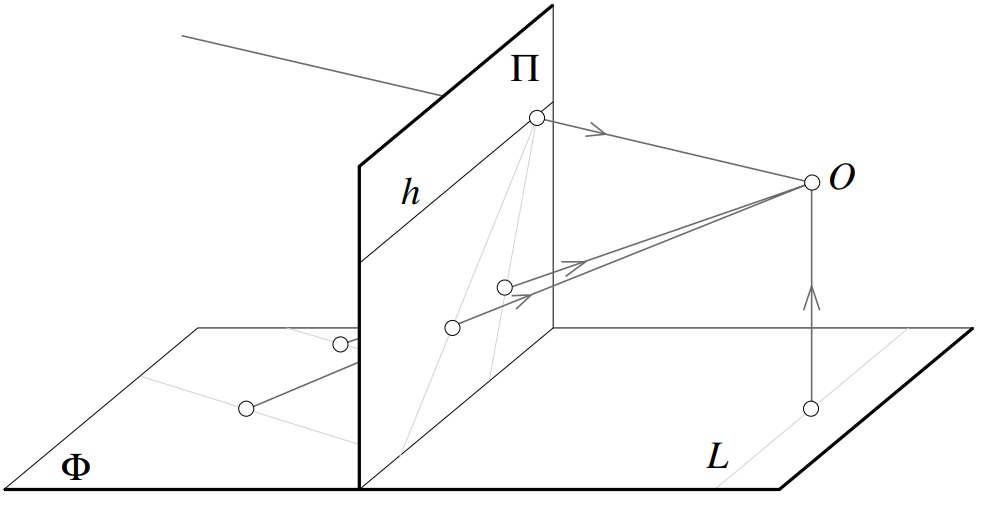
Consider the diagram below:

We can see from the plane $\Phi$ two parallel lines on its surface. Then these two lines are projected onto the plane $\Pi$ where they appear to be intersecting.

My question is how did they become intersecting? and how is the perspective defined in this figure? that is to say from what field of view is the person looking at this diagram to see this phenomena? Because I don't understand what this diagram is specifically describing when it connects all these rays to the optical center $O$.

I have attached an example of train tracks and hope someone can help me understand it as an example of this phenomena

Answer 1

In the train track picture, the rails never meet, but appear to. The point where they appear to meet is infinitely far away, but there is a definite angle to look to see the point of intersection (we must look at the middle of the picture).

In the top diagram the observer at $O$ must look in the direction $O\Pi$ to see where the two lines appear to meet (even though they never actually meet).

Answer 2

The diagram is confusing, but if you let the O be the observer and the two points in the region Φ be on two rails, then as the rails move away from the observer, the visual angle between them gets smaller. (You have a triangle with a fixed base, but an increasing height.)

Answer 3

Consider a two-dimensional case where a point on the x-axis is being projected onto the y-axis. Let's say we have a person at point P with coordinates $(P_x,P_y)$, an object at point A at coordinates $(A_x,0)$, and a point on the y-axis A' that A is projected onto at coordinates $(0,A'_y)$. We can draw a right triangle $(A_x,0),(0,0),(0,A'_y)$, and another right triangle $(A_x,0),(P_x,0),(P_x,P_y)$. The term "projection" refers to the line from P to A' being the same as the one going from P to A, so these two triangles are similar, and from that we can conclude that $\frac {P_x}{P_x+A_x}=\frac{P_y-A'_y}{A'_y}$. If we solve for $P_y-A'y$, we get $P_y-A'_y=\frac{P_xA'_y}{P_x+A_x}$. Thus, as the distance to A goes to infinity, the vertical separation between the person and the projected point goes to zero. The point with no vertical separation is the vanishing point. Since all lines are converging towards this point as they go to infinity, they also converge to each other.