How does photon-photon interaction manifest in QED?

Question

In classical electrodynamics the electromagnetic field only interacts with charged particles, but in quantum electrodynamics (QED), there is a very weak interaction between photons. The lowest order contribution comes from this Feynman diagram:

Having four vertices, we see that its contribution is extremely low compared to for example electron-electron interaction, it is non-zero though. Computing the contribution to the $S$-matrix element would give a first approximation of the scattering cross-section.

However, could we compute how this interaction would manifest itself more precisely? Will it act as an attractive or a repulsive force between photons, or even something else altogether?

Answer 1

I am fully disclosing off the bat here that I do not know of a plausible way/gimmick to attribute an "attractive-versus-repulsive-force" feature to these amplitudes, a subject discussed amply on this site, as the relevant 2-to-2 scattering cross sections cannot be used for these purposes (C-conjugation). You might have to strain to define this feature here. Photons scatter off each other in precise ways impervious to any attractive-vs-repulsive cartoon interpretations.

A state-of-the art friendly discussion of the (1936) Euler–Heisenberg effective quartic Lagrangian describing the process and the Delbrück scattering and quantum birefringence phenomena involved, is to be found in Thiescheffer's (2017) thesis, and especially the discussion around his (3.14).

The full differential cross section for $γγ\to γγ$ was calculated by Karplus & Neuman (1951), who dealt with all polarization states, angular distributions, etc... The cross-section goes as $α^4/s$, in contrast to $γγ\to e^+e^-$, which goes as $α^2/s$, so it's four orders of magnitude weaker! (α=1/137.)

You could pretend you read off the 4γ effective contact interaction from the quartic "potential" term of the E-H lagrangian, $$ \mathcal{L} = \frac{1}{2}\left(\mathbf{E}^{2}-\mathbf{B}^{2}\right)+\frac{2\alpha^{2}}{45 m_e^{4}}\left[\left(\mathbf{E}^2 - \mathbf{B}^2\right)^{2} + 7 \left(\mathbf{E}\cdot\mathbf{B}\right)^{2}\right], $$ the Maxwell quadratic one being the "kinetic" term.

Answer 2

There are already excellent answers posted here. Let me add two aspects:

• This was actually measured for the first time a few years ago at LHC https://www.nature.com/articles/nphys4208 (I was peripherally involved in a comparable CMS measurement)

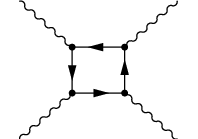
• It makes sense to talk about attraction/repulsion only if the initial state particle at least survives the interaction. That is a minimal requirement. These are basically so-called t-channel interactions. The quartic coupling is no such thing. No world line goes from initial state to final state without changing identity. This is a fully inelastic/destructive process.

EDIT: after the nice comment below I agree that the second point is too illustrative and not really correct. While the identity of initial and final state particles remains relevant, there is another very important fact: any Feynman diagram never describes a "force" or even any action. Such a diagram is just a small part of a series expansion of amplitudes (QM transition probabilities), of which only the squared sum over many (actually infinitely many) other Feynman diagrams with the same incoming and outgoing particles, gives a meaningful "transition probability".

However, it is possible to work out from these amplitudes the underlying potential energy at work, and from the signs and shapes of the potential, one can derive attractive vs. repulsive behaviour. We find that for spin-0 Yukawa exchange the potential is always attractive, for any spin-1 exchange (QED) the potential can be both attractive and repulsive, for a spin-2 exchnage (gravity) it is attractive only.

And, importantly, for a quartic coupling the potential turns out to be a delta-function meaning that the force has "no reach". It is neither attractive nor repulsive. Anything else would have been also very strange, since photons and photon-beams clearly show no signs of any repulsion nor attraction even on the largest distance scales.