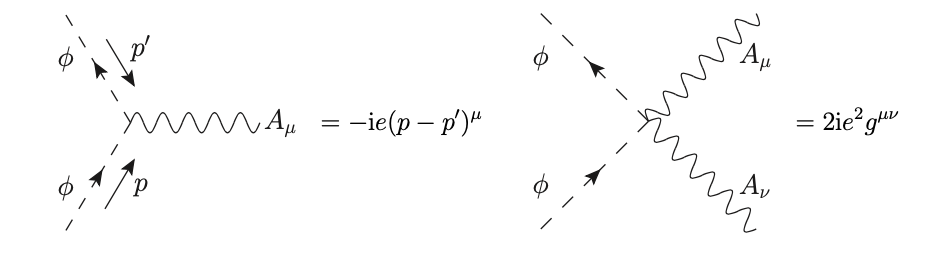Scalar QED differential cross section

Question

I have a question that I'm struggling to solve. Here is the background: In scalar QED, the electron is replaced by a charged scalar particle $\phi$, and its corresponding antiparticle $\phi^{*}$, which couple to the photon through the following interaction vertices.

I've been trying to calculate the differential cross section $\frac{d\sigma}{d\cos \theta}$ for the process $\phi \phi^* \to \phi \phi^*$, in the centre of mass frame.

In my attempt to solve this, I read about toy theory in David Griffiths' particle physics book, which is a simplified version of QED where one can disregard spin. I was thinking that this might be the way to go in order calculate the differential cross section, but I might also be wrong.

Question: Calculate the differential cross section $\frac{d\sigma}{d\cos \phi}$ for the scattering process $\phi \phi^{*}\to\phi \phi^{*}$ in the centre of mass frame, to lowest order in $e$.

Answer 1

I will outline how to link an observable such as scattering amplitude to a Feynman diagram. These topics are too long to write on this site, so for more details, please refer to basic textbooks such as Schwartz.

The following figure shows the minimum steps required. If you do not understand any part of this figure, you do not understand that step, and you should focus on that part of the figure by relying on the key words in the diagram. In other words, if you don't understand this diagram, you don't know enough to use quantum field theory to calculate scattering cross sections.

$\begin{array}{cccccc} & & &\mathrm{Observables:}{\frac{d\sigma}{d\Omega},\ \Gamma}& \\ & & &|&&\\ & & &|& \hspace{-90mm}\mathrm{By\ definition} \\ & & &\downarrow& \\ & & &\mathrm{S\textrm{-}matrix:}{\langle \beta|S|\alpha\rangle }& \\ & & &|&& \\ & & &|&\hspace{-70mm} \color{red}{\mathrm{LSZ}\ \mathrm{reduction}\ \mathrm{formula}} & \\ & & &\downarrow& \\ & & &\mathrm{n\textrm{-}point\ functions:}{\langle 0|T\phi(x_1)\cdots\phi(x_n)|0\rangle }&\\ & & &|&& \\ & & &|&\hspace{-50mm} \color{red}{\mathrm{Wick’s\ Theorem\ \&\ Feynman\ Rule}} & \\ & & &\downarrow& \\ & & &\mathrm{Products\ of \ free\ propagators:}{\langle 0|T\phi_0(x_1)\phi_0(x_2)|0\rangle }&\\ & & &|&& \\ & & &|&\hspace{-15mm} & \\ & & &\downarrow& \\ & & &\mathrm{Feynman\ Diagrams}& \end{array} $

Here is a description of each step.

First step

The first arrow follows from the definition: $$\Big(\frac{d\sigma}{d\Omega}\Big)_{\alpha\to\beta} =\int\frac{d^3k_f}{(2\pi)^3} \frac{d^3p_f}{(2\pi)^3} \frac{(2\pi)^4\delta^4(p_i+k_i-p_f-k_f)}{4E’_{p_i}E’_{p_f}4E_{k_i}E_{k_f}v } |\mathcal{T}_{\alpha\beta}|^2.$$

Above, I have written the formula for the case of two particles scattering to two particles, but for more general cases, the above integral measures, etc. are subject to change, so please refer to the appropriate textbook. Also, the specific form of this formula may differ slightly from textbook to textbook, but it is essentially the same, so one should understand one textbook first.

Also, in the above formula, $\mathcal{T}$ is $\langle \beta|S|\alpha\rangle$ minus thetrivial case where the particles do not interact at all:$\langle \beta|(S-1)|\alpha\rangle $ This is often also written as $\mathcal{M}$. Read the textbook for more details.

Second step

Essentially, all you have to do is calculate $\mathcal{M}$. For this, there is a convenient formula known as the LSZ reduction formula. This is the second arrow. See the above link for exact form of this formula. What is important is that, thanks to this formula, the quantity $\langle \beta|S|\alpha\rangle$, which is unclear how to calculate, is transformed into $\langle 0|T\phi(x_1)\cdots\phi(x_n)|0\rangle$ that is easy to calculate.

In the end, what you should calculate is the following time-ordered product: $$\langle 0|T\phi(x_1)\cdots\phi(x_n)|0\rangle$$

Once this is calculated, all you have to do is to substitute $\langle 0|T\phi(x_1)\cdots\phi(x_n)|0\rangle$ into the LSZ formula and calculate $\mathrm{M}$, and to substitute this $\mathrm{M}$ into the definition of scattering cross section. (Above I wrote $\mathcal{M}$ by $\mathcal{T}$, but they are same.) After that, you must calculate the momentum integral included in the definition of the scattering amplitude, and that is all. The calculation of this integral is also technical and tricky, but you can probably find examples in any textbook, so I won't go into it here.

Third step

Now, to return to the subject, there is the following formula for calculating the time-ordered product using the path integral.

$$\langle 0|T\phi(x_1)\cdots\phi(x_n)|0\rangle=\frac{\int D\phi \ \phi(x_1)\cdots\phi(x_n) e^{i\lambda S_{\mathrm{int}}}e^{iS_{\mathrm{free}}}}{\int D\phi e^{ iS}}\propto {\int_\mathrm{connected\ diagrams} D\phi\ \phi(x_1)\cdots\phi(x_n)\Big\{\sum_n\lambda^n\Big(\frac{S_{\mathrm{int}}^n}{n!}\Big)\Big\}e^{iS_{\mathrm{free}}}} $$ Here I wrote the perturbation parameters (coupling constants) $\lambda$ explicitly. If you consider the perturbative calculation, what you have to do here is expand the summation above up to the appropriate order of $\lambda$ and calculate the time-ordered product. This is the third step. Here, we use path integral formalism. Actually, there is another equivalent method that uses the operator form, but since I think the path integral method is easier, I will not introduce it here. See Srednicki's first few chapters for a derivation of the above equation using path integrals. If you are interested in operator forms, read Schwartz and Peskin.

Anyway, from the expression above, what we have to do is replaced by computing a conncted n-point function defined as follows $$\langle 0|T\phi(x_1)\cdots\phi(x_n)|0\rangle_\mathrm{connected}:= {\int_\mathrm{connected\ diagrams} D\phi\ \phi(x_1)\cdots\phi(x_n)e^{iS_{\mathrm{free}}}}.$$

If you don't know anything about connected diagrams, refer to some textbook. The method for calculating this time-ordered product like above is known as Wick's theorem. Using this, the above time-ordered product can be expanded in terms of the free-field propagator:

$$\langle 0|T\phi(x_1)\cdots\phi(x_n)|0\rangle_\mathrm{connected}= G_0(x_1-x_2)\cdots G_0(x_{n-1}-x_n)+(\mathrm{permutations})$$

If one uses path integrals, this formula can be easily derived by simply performing a Gaussian integral. See Srednicki for details.

Here, I will give one example. Let’s consider the scalar QED. In this case, $\mathcal{L}^{(1)}_{\mathrm{int}}\sim (ie\phi^*(\partial_\mu \phi )A^\mu+h.c.)$ and $\mathcal{L}^{(2)}_{\mathrm{int}}\sim (-e^2\phi\phi^*A_\mu A^\mu$). I don’t Check the coefficients carefully, so please check them by yourself. We are interested in the process $\phi\phi^*\rightarrow \phi\phi^*$, from the formulae above at the order of $e^2$ what we have to calculate is the following two connected n-point functions: \begin{align} &\langle 0|\phi_{x_1}\phi^*_{x_2}\Big(e^2\frac{1}{2!}(\mathcal{L}^{(1)}_{\mathrm{int}} )^2\Big)\phi_{y_1}\phi^*_{y_2}|0\rangle_{\mathrm{connected}} \\&=-\frac{e^2}{2} \langle 0|\phi_{x_1}\phi^*_{x_2} \phi^*_{z_1} (\partial_\mu \phi_{z_1}) A_{z_1}^\mu \phi_{z_2}^*(\partial_\nu \phi_{z_2} )A_{z_2}^\nu \phi_{y_1}\phi^*_{y_2}|0\rangle_{\mathrm{connected}}\\ \end{align}

Applying wick's theorem to this, we can write a connected n-point function in terms of a product of propagator and vertex. This is the forth step. A shortcut for these operations is called the Feynman rule. I recommend that you do a number of silly expansions like the one above until you get used to them, and then use the Feynman rule once you know how to do them. You can find how to derive the Feynman rule in any textbook, but I recommend Srednicki because you only need to read the first few chapters.

Also, in your example, there are one more diagram which contribute to the calculation at order $e^2$; that is $$\langle 0|\phi_{x_1}\phi^*_{x_2}\Big(-ie^2(\mathcal{L}^{(2)}_{\mathrm{int}} )\Big)\phi_{y_1}\phi^*_{y_2}|0\rangle_{\mathrm{connected}} .$$ So you must calculate this n-point function.

Anyway, I recommend you first to figure out what step of the figure above you don't understand, and study it in the appropriate textbook.

Answer 2

We have two tree level diagrams in 2'nd order of e, these are identical to the Bhabha-scattering diagrams, but with fermions exchanged for charged scalars, see Bhabha scattering for reference. You can figure out which feynman diagrams are possible for a given reaction by checking which combinations of vertices produce it.

The simplest "how to" I can give for getting the amplitude from a diagram is that you simply multiply together the contributions from the different parts of the diagram in order, e.g. in our annihilation case we have:

(outgoing $\phi$)(outgoing $\phi^*$)(vertex)(photon propagator)(vertex)(incoming $\phi$)(incoming $\phi^*$)

Where the propagator is : \begin{align} i\mathcal{D}_{ab} &= \frac{i\eta_{ab}}{(k)^2} \end{align} in the t'hooft gauge, and the ingoing and outgoing particles each contribute a multiplicative 1. Note that how the particles relate to the vertex is given by the lagrangian and is not generally (particle 1)(particle 2)(vertex).

We then get that the diagrams have the amplitudes:

\begin{align} i\mathcal{M}_1 &= (-ie(p-q)^a)\frac{i\eta_{ab}}{(p+q)^2}(-ie(p'-q')^b)=-ie^2\frac{(p-q)_a(p'-q')^a}{(p+q)^2} = -i2e^2\frac{p_a p'^a -p_aq'^a}{(p+q)^2}\\ i\mathcal{M}_2 &= (-ie(p-p')^a)\frac{i\eta_{ab}}{(p-p')^2}(-ie(q'-q)^b)= -i2e^2\frac{p_a q'^a -p_aq^a}{(p-p')^2}\\ i\mathcal{M}&=i\mathcal{M}_1+i\mathcal{M}_2 = -i2e^2(\frac{p_a p'^a -p_aq'^a}{(p+q)^2}+\frac{p_a q'^a -p_aq^a}{(p-p')^2}) \end{align}

To calculate the cross section we need the following two identities: \begin{align} \delta(f(x-a))&=\frac{1}{f'(a)}\delta(x-a)\\ E_k&= \sqrt{k^2-m_k^2} \end{align}

And the formula for the cross-section:

\begin{align} d\sigma &= \frac{(2\pi)^4|\mathcal{M}|^2}{4((p_{in1}*p_{in2})^2-m_1^2m_2^2)^{1/2}}\delta^4 (P_{incoming} - P_{outgoing})\Pi_k^{N_b}\frac{d^3k'}{(2\pi)^32E_{k'}} \end{align}

We can now calculate the cross-section as: \begin{align} d\sigma &= \frac{(2\pi)^4|\mathcal{M}|^2}{4((p_aq^a)^2-m_p^2m_q^2)^{1/2}}\delta^4 (p + q - p' - q')\frac{d^3q'}{(2\pi)^32E_{q'}} \frac{d^3p'}{(2\pi)^32E_{p'}}\\&= \frac{|\mathcal{M}|^2}{16(2\pi)^2((p_aq^a)^2-m_p^2m_q^2)^{1/2}}\delta (E_{p} + E_{q} - E_{p'} - E_{q'})\frac{d^3q'}{E_{q'}E_{p'}} \\&= \frac{|\mathcal{M}|^2}{16(2\pi)^2((p_aq^a)^2-m_p^2m_q^2)^{1/2}}\delta (E_{p} + E_{q} - E_{p'} - E_{q'})\frac{|q'|^2d|q'|d\varphi d\cos{\theta}}{E_{q'}E_{p'}}\\ &= \frac{|\mathcal{M}|^2d\cos{\theta}}{16(2\pi)((p_aq^a)^2-m_p^2m_q^2)^{1/2}}\frac{\delta (E_{p} + E_{q} - \sqrt{|q'|^2 +m_p^2} -\sqrt{|q'|^2 +m_q^2})|q'|^2d|q'|}{E_{q'}E_{p'}}\\ &= \frac{|\mathcal{M}|^2d\cos{\theta}}{16(2\pi)((p_aq^a)^2-m_p^2m_q^2)^{1/2}}\frac{|q'|^2}{E_{q'}E_{p'}}(\frac{|q'|}{E_{q'}}-\frac{|q'|}{E_{p'}})^{-1}\\ &= \frac{|\mathcal{M}|^2d\cos{\theta}}{16(2\pi)((p_aq^a)^2-m_p^2m_q^2)^{1/2}}\frac{|q'|}{E_{q'}+E_{p'}} \end{align} giving us: \begin{align} \frac{d\sigma}{d\cos{\theta}} &= \frac{|\mathcal{M}|^2}{16(2\pi)((p_aq^a)^2-m_p^2m_q^2)^{1/2}}\frac{|q'|}{E_{q'}+E_{p'}} \end{align}

Looking at the kinematics of this reaction in the center of mass frame, we can use the following parameterisation(using 4-momentum conservation):

\begin{align} p^a &= (E,|p|,0,0)\text{; }q^a = (E,-|p|,0,0)\text{; }p'^a = (E,|p|\cos{\theta},|p|\sin{\theta},0)\text{; }\\q'^a &= (E,-|p|\cos{\theta},-|p|\sin{\theta},0) \end{align} Which gives us the following expressions:

\begin{align} E &= \sqrt{|p|^2+m^2_\phi}\text{; } p_aq^a= 2E^2-m^2_\phi \text{; } p_ap'^a = E^2(1-\cos{\theta})+m^2_\phi\cos{\theta} \\ p_aq'^a&= E^2(1+\cos{\theta})-m^2_\phi\cos{\theta}\text{; } (p-p')^2 = -|p|^2((1-\cos\theta)^2+\sin^2\theta) = -2|p|^2(1-\cos\theta)\text{ ; }(p+q)^2 = 4E^2 \end{align}

Rewrite $|\mathcal{M}|^2$: \begin{align} |\mathcal{M}|^2 &= 4e^4(\frac{p_a p'^a -p_aq'^a}{(p+q)^2}+\frac{p_a q'^a -p_aq^a}{(p-p')^2})^2\\ &=4e^4(\frac{-2E^2\cos{\theta}+2m^2_\phi\cos{\theta}}{4E^2}+\frac{-E^2(1-\cos{\theta}) +m^2_\phi(1-\cos{\theta})}{-2|p|^2(1-\cos\theta)})^2 \\ &= 4e^4(\frac{-2(E^2-m^2_\phi)\cos{\theta}}{4E^2}+\frac{1}{2})^2 = e^4((\frac{m^2_\phi}{E^2}-1)\cos{\theta}+1)^2 \end{align}

And our crosssection becomes:

\begin{align} \frac{d\sigma}{d\cos{\theta}} &= \frac{|\mathcal{M}|^2}{16(2\pi)(4E^4-4E^2m^2_\phi)^{1/2}}\frac{ \sqrt{E^2-m_\phi^2}}{2E} = \frac{|\mathcal{M}|^2}{64(2\pi)E^2} \\ &= \frac{e^4((\frac{m^2_\phi}{E^2}-1)\cos{\theta}+1)^2}{64(2\pi)E^2} \end{align}

You will want to do this calculation for yourself to make sure I have not messed it up, anyway hope this helps a little!