A solution
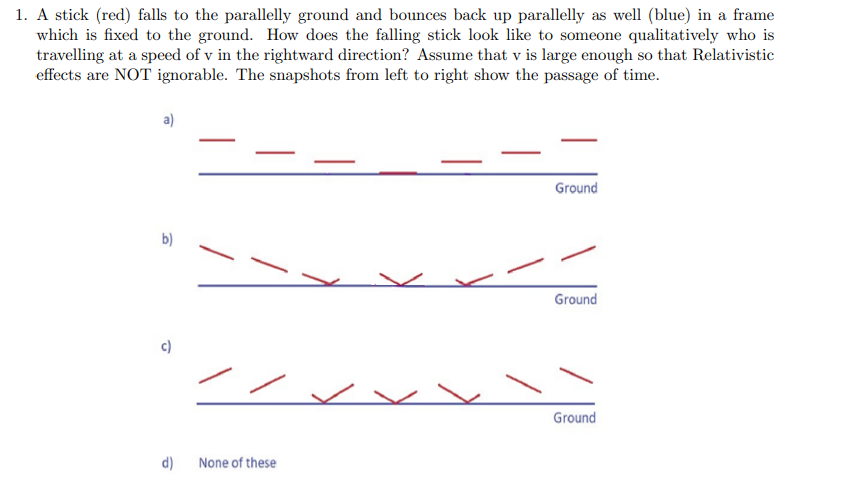
The observer moves rightwards wrt. ground. In the options are shown observer's observations, with its time increasing rightwards too.
Assuming no gravity and periodicity of snapshots, options $a$ and $d$ are ruled out based on effects of simultaneity and either $b$ or $c$ showing said effects. Moreover $b$ and $c$ differ in only which end falls first.
Even without doing any math, the right end of the rod must fall first.
To see this, initially position the moving observer at rod's midpoint$^1$. As time progresses, light from the right end would reach the observer before that from the left end. Since the observer isn't aware of its own motion and speed of light is frameless, it concludes that the right end started falling sooner
Mathematically, let the moving observer's frame be $(x',t')$ and the gorund's frame be $(x,t)$. The events
$$
\begin{align}
A&=(x_a,t_a)=\text{left end falls} \\
B&=(x_b,t_b)=\text{right end falls}
\end{align}
$$
with the constraint
$$
t_a=t_b=t_0\tag{0}
$$
would become for the observer $A'=(x'_a,t'_a)$ and $B'=(x'_b,t'_b)$ where
$$
t'_i=\gamma(t_i-\beta x_i) \tag{1}
$$
with $c=1,\beta=v_{observer},\gamma=(1-\beta^2)^{-1/2}$. Using eqns. $0$ and $1$
$$
\begin{align}
t'_b-t'_a&=\gamma(0-\beta (x_b-x_a))\\
&=-\gamma\beta L_0\\
&<0\hspace{4cm}(\beta>0,L_0>0)
\end{align}
$$
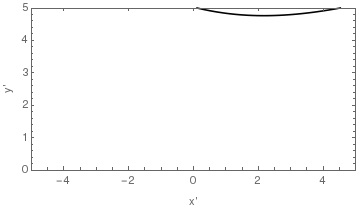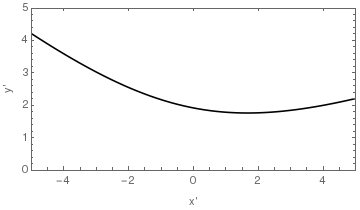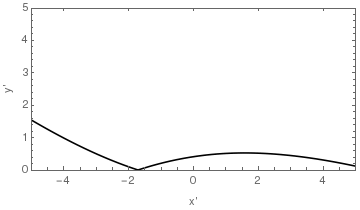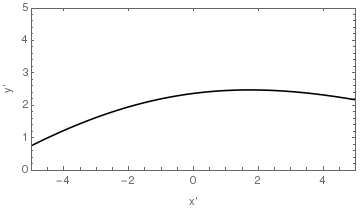
therefore $t'_a>t'_b$ and the left end is observed falling later, vindicating option $b$.
Also
Terrel rotation of a falling stick
IMHO the question isn't about Terrel rotation. That requires evaluating actual views visible to observer, requires knowledge of the observer's position, wouldn't have kept the rod straight in the shown options, and is a more meta application of STR, that seems a bit advanced for the question.
The first option is obviously incorrect.
Good for you. To me there's nothing obvious about its incorrectness. If anything its obviously correct. All my non-relativistic life has drilled the notion down into my bones of falling rigid rods not behaving like reflecting light rays, just because they move.
Now when I try to visualize the problem, it seems to me that c) should be the correct option since the left end of the stick is closer to the eye as compared to the right end.
Doesn't that depend on where the moving observer initially was? As discussed earlier, to use that argument for the current case, you must observe from a special position - the midpoint.
Now, how to approach such problems where it seems that mere visualization is needed but which apparently doesn't work (at least for me)?
Usually one can trust and straightforwardly use Lorentz transform eqns. to give and get the correct answer. Developing visual intuition for relativistic scenarios isn't easy. Even Minkowski diagrams have a element of math to them. To see the clearest non-mathematical arguing of expected relativistic consequences in simple cases, I recommend a reread of Einstein's insightful arguments in his $1905$ STR paper.
$^1$ for this argument, the moving observer must be so positioned that if it weren't moving, the falling of the rod's ends would appear simultaneous.