0
$\begingroup$

I've been dealing with a kind of problem in quantum mechanics, where they give us an arbitrary potential, and then ask us to predict the form of the probability amplitude or the wave function.

The potentials that they provide us, are usually functions of x, and thus vary slowly.

My original intuition is, to take a few points in the potential, plug that into the schroedinger equation, and find :

$$ k = \sqrt{\frac{2m(E-V(x))}{\hbar^2}} $$

Thus, I find the value of $k$ at these points, by plugging the values of $V(x)$ and $E$ into the equation. Moreover, I know that :

$$k \propto \frac{1}{\lambda} $$

Now that we have the wavelength, we also know that :

$$ A \propto \lambda $$

Thus, I've managed to find the amplitudes at these three points.

Using this knowledge of the amplitude, I'm able to select the correct option, by checking which one fits my predictions best.

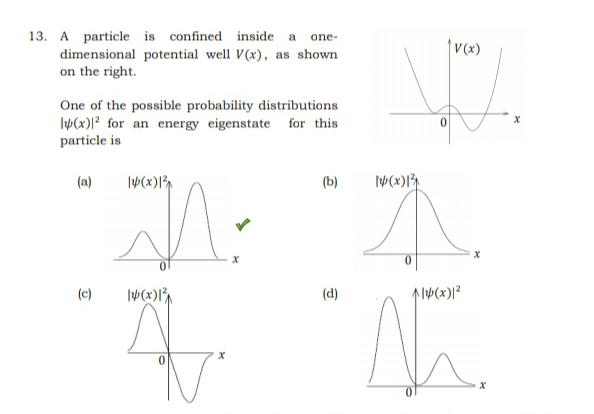
However, recently, I came across this question : enter image description here

I was unable to find the reasoning behind this particular answer. My reasoning: If we take the energy of the particle to be less than 0, then the probability distribution to the left of the origin should decay exponentially, as $k$ would be imaginary, and the wave function would decay exponentially.

Can anyone help me out on how to solve this problem?

Improve this question
$\endgroup$
6
  • $\begingroup$ Probability peaks at low potential energy. That should tell you how the left-hand side compares with the right. $\endgroup$
    – J.G.
    Commented Jun 9, 2021 at 20:16
  • $\begingroup$ @J.G. the well is deeper on the right, so the particle should move faster, and spend less time there. So, shouldn't probability be more on the left instead, where it is shallower ? $\endgroup$
    – Nakshatra Gangopadhay
    Commented Jun 9, 2021 at 20:21
  • 1
    $\begingroup$ @NakshatraGangopadhay, I don't know how useful it is to think about a particle moving faster or slower in this case. When you're talking about a wave function, sometimes particle descriptions can be counterintuitive. But if you insist, in this case, you could imagine the region of lower potential is due to the attraction of charged particles. An electron moving towards a proton would be like moving to lower potential energy. So the difference in potential results in a force that will bring the electron to sit in the lower potential well more often than not. $\endgroup$
    – GrassyNol
    Commented Jun 9, 2021 at 20:29
  • $\begingroup$ This is essentially quasiclasdical/adiabatic/WKB approximation. You can learn more by searching through qm books - they all cover it, but to a different extent. $\endgroup$
    – Roger V.
    Commented Jun 9, 2021 at 20:32
  • $\begingroup$ This is essentially quasiclasdical/adiabatic/WKB approximation. You can learn more by searching through qm books - they all cover it, but to a different extent. $\endgroup$
    – Roger V.
    Commented Jun 9, 2021 at 20:32

2 Answers 2

Reset to default
2
$\begingroup$

The question asks you to identify a possible probability distribution is for the particle. Only one of these answers is possible. Note that if the particle's energy is greater than 0, then the wave function will not decay exponentially around the local minimum in the negative x region.

However, it is still true that the wave function should be larger in the lower potential region than in the high potential region. Choice (b) is symmetric, so that's out. Choice (c) is not only symmetric, but has a negative probability (I'm assuming they mean to plot $\psi$ rather than $\psi^2$, but either way that's out). Choice (d) would imply that the particle is more likely to be found in the high potential region, which can't be the case. That leaves (a) as the only possible option.

Improve this answer
$\endgroup$
0
$\begingroup$

Let me address your expectation that $|\psi|^2$ would be higher where kinetic energy is low, i.e. where potential energy is high, which (as I and others have noted) is the opposite of what happens.

Let's consider a simpler example you've mentioned, namely the (presumably simple) harmonic oscillator, for which your reasoning would predict $|\psi|^2$ is minimal at the centre and rises away from that, so $\int_{-\infty}^\infty|\psi|^2dx$ can't be $1$.

The correct intuition is that higher potential is harder to reach - indeed, its exceeding $E$ would be literally impossible were it not for quantum mechanics - so is less probable.

As for "high KE makes it leave", the point is moot because the flow in and out are both at that KE, and so there's no bias there toward leaving.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.