We know that changing magnetic fields ($dB/dt \neq 0$) produce electric fields in circular loops. So if we keep a stationary charge in the changing magnetic field, will it experience any force due to the induced electric field?
$\begingroup$
$\endgroup$
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
2
Yes.
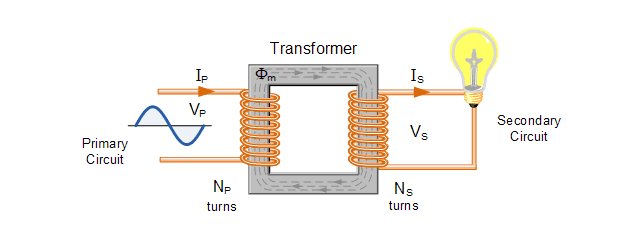
As an example from life, consider a transformer. When the primary current is zero, there is no current in the secondary circuit too. All the electrons are stationary (from a classical perspective). After you have started the primary current, the magnetic field in the core will change. This leads to an electrical field in the secondary circuit and electrons moving due to an electrical force acting on them.
-
$\begingroup$ Actually I want to ask, is it necessary to have a conducting loop of wire in which electrons move or any isolated charge will experience force? $\endgroup$ Commented Apr 28, 2021 at 20:43
-
$\begingroup$ You don't need a wire for this. It's just in everyday life charges to test an electrical force happen mostly in wires. $\endgroup$ Commented Apr 28, 2021 at 21:35
$\begingroup$
$\endgroup$
Will a stationary charge be affected by changing magnetic field?
Yes. The force on a charge by an electro-magnetic field is called the Lorentz force, and is given by the equation
$$\vec{F} = q(\vec{E} + (\vec{v} \times \vec{B}))$$
Where
- $q$ is the charge
- $\vec{E}$ is the electric field
- $\vec{v}$ is the velocity of the charge
- $\vec{B}$ is the magnetic field
Since you have specified that the charge is stationary, the force becomes
$$\vec{F} = q\vec{E}$$
Since the question asks about an electric field induced by a changing magnetic field, the electric field $\vec{E}$ is a solution to the equations
$$\nabla \times \vec{E} = -\frac{\partial \vec{B}}{\partial t}$$ $$\nabla \cdot \vec{E} = 0$$
answered Apr 28, 2021 at 11:26