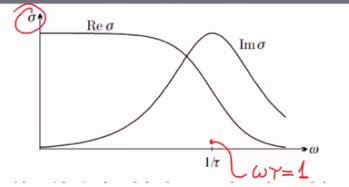
I apologize if this question has been asked before. My Solid State professor was explaining the Drude model, and what happens to a solid when we apply a time-dependent electric field. In a lecture, we derived the formula for the real and imaginary parts of the conductivity as a function of the electric field frequency as follows:
Re($\sigma(\omega$)) = $\frac{\sigma_0}{(1+(\omega\tau)^2)}$
Im($\sigma(\omega$)) = $\frac{\sigma_0\omega\tau}{(1+(\omega\tau)^2)}$
By plotting the real part of the conductivity, he deduced that, as the applied electric field increases, the conductivity will go to zero, because the drift velocity will go to zero. He argued that the electrons simply do not have time to follow the fast changes in the electric field. However, the relation between the drift velocity and the electric field is proportional.
Could someone explain to me what my professor meant, and if his reasoning is wrong, why does the conductivity go to zero for very high electric fields?
I think this might have something to do with the dissipation of energy due to collisions of electrons with other electrons or other particles in the sample, but I am not sure.
Note: we are following the book from Ashcroft and Mermin, Solid State Physics.