2
$\begingroup$

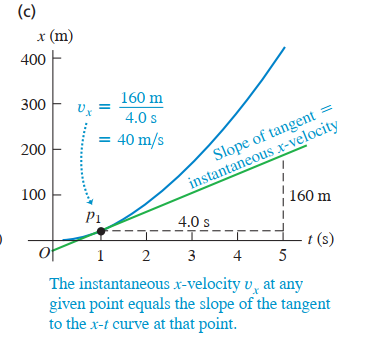
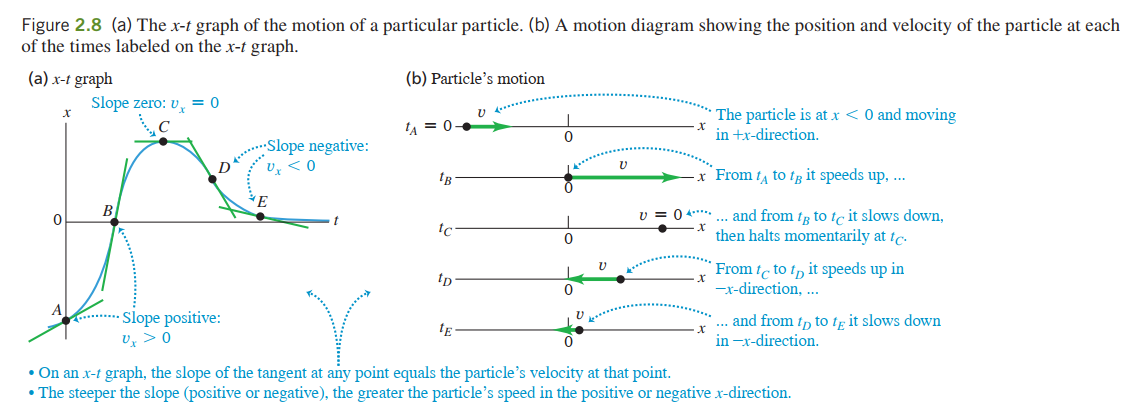
$\text{Slope= tan(angle with respect to positive X-axis)= scalar output}$

$\text{velocity= a vector }$

graph

Source: Hugh D Young_ Roger A Freedman - University Physics with Modern Physics In SI Units (2019, Pearson) Page-67

Then, doubts are;

1.Is the relation "Slope of tangent=instantaneous x-velocity" valid, as it would mean "scalar=vector"?

2.Even if I write "Slope of tangent=instantaneous x-speed", if the tangent makes obtuse angle slope will be negative, and we know that instantaneous speed is magnitude of instantaneous velocity, which makes instantaneous speed a positive term. So, what exactly does the slope give?

Extra information:enter image description here

Source: Hugh D Young_ Roger A Freedman - University Physics with Modern Physics In SI Units (2019, Pearson) Page-67

Similar confusion arises in,$\text{ "Area under a x-t graph = change in x-velocity from time 0 to time t"}$, with right hand side vector and left hand side (area) as scalar. I think answer to the original question, provides solution to this confusion as well.

Improve this question
$\endgroup$
1

1 Answer 1

Reset to default
3
$\begingroup$

Keep in mind there is a difference between a vector and a component of a vector. The velocity is a vector, the x-component of the velocity (or the "$x$-velocity" in the language your book uses) is just a component of a vector, which is a scalar.

The velocity vector can be expanded in terms of unit vectors $\mathbf{e}_x, \mathbf{e}_y, \mathbf{e}_z$ (which satisfy $\mathbf{e}_x\cdot \mathbf{e}_x=1, \mathbf{e}_x\cdot \mathbf{e}_y=0$, etc): \begin{equation} \mathbf{v}=v_x \mathbf{e}_x + v_y \mathbf{e}_y + v_z \mathbf{e}_z \end{equation} The slope of the line you wrote down gives you $v_x$, which is a scalar given by $v_x=\mathbf{v}\cdot \mathbf{e}_x$. This quantity is a scalar, so there's no problem setting it equal to a slope. It is also a signed quantity, so it's not a speed (there's no requirement that $v_x>0$).

Improve this answer
$\endgroup$
12
  • $\begingroup$ A component of a vector is a vector in its own right. Otherwise, you couldn't add vector components to arrive at a resultant. $\endgroup$
    – David White
    Commented Feb 11, 2021 at 1:34
  • $\begingroup$ @DavidWhite $v_x=\mathbf{v}\cdot{e}_x$ is a scalar, not a vector, and this is the quantity I mean by "the $x$ component of $\mathbf{v}$" (I also think this is standard usage). Of course $v_x \mathbf{e}_x$ is a vector but I would not refer to this as the $x$ component of $\mathbf{v}$. $\endgroup$
    – Andrew
    Commented Feb 11, 2021 at 1:38
  • $\begingroup$ @DavidWhite "CAUTION: Components are not vectors", I just copy-pasted from the same book mentioned in question (Page 42). In general "component of a vector" means "scalar components" and "vector components(needs to explicitly mentioned) implies "vector components", this is the term you have mentioned as "A component of a vector..." $\endgroup$
    – Sahil
    Commented Feb 11, 2021 at 2:21
  • 1
    $\begingroup$ @Sahil, if the vector component has a magnitude (it surely does) and if the vector component has a direction (it should), it qualifies as a vector. If you are just talking about the magnitude of a vector component, that is a scalar. If that quote is from your book, then the book's authors are not using the word "component" the same way that I used it during the 13 years that I was teaching physics to high school students, and they are not using that word the same way that I have seen it used in every other physics book that I have read or used. $\endgroup$
    – David White
    Commented Feb 11, 2021 at 2:59
  • $\begingroup$ @DavidWhite, From what I have learnt using the book, Magnitude is a positive entity, scalar on the other hand can be positive or negative. Vector component is a vector, scalar component(generally termed as "component") is a scalar. $\endgroup$
    – Sahil
    Commented Feb 11, 2021 at 3:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.