Classical scattering cross section and attractive potentials

Question

If I want to calculate a scattering cross section $\sigma$ for a classical central potential $V(r)$, the first thing to do is to obtain an expression for the angle

$$ \Theta=\pi-2\int_{r_*(b,E)}^{\infty}\frac{b}{r^2\sqrt{1-\frac{b^2}{r^2}-V(r)/E}}dr $$

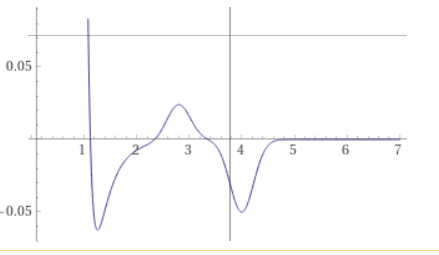
where $r_*(b,E)$ is the turning point obtained by equating the effective potential to the total energy $E$: $$ 0=1-\frac{b^2}{r_*^2}-\frac{V(r_*)}{E} $$ and $b$ stands for the impact parameter. Let's say I want to solve this numerically. The problem is that, if the potential $V$ has ranges of values for which it is attractive, there might be choices of $(b,E)$ in which the above equation might have multiple solutions for $r_*$ (imagine for instance a Lennard-Jones type potential but two attractive wells rather than one, like below):

Which value would I choose to insert in the integral for $\Theta$? Would the equation for $\Theta$ change in any way? Or, how would you go about estimating $\sigma$ numerically in a situation when I can have multiple solutions for $r_*$ for some choices of $(b,E)$?

Answer 1

The particle is coming in from infinity, so the classical turning point is the greatest solution $r_*$ to your second equation. This corresponds to the first place beyond which, if the particle continued moving toward the origin, its radial kinetic energy would be negative. (The angular part of the kinetic energy is part of the effective potential.)