Probability of forming a proton or neutron with loose quarks?

Question

If I had two unbound up quarks and a down quark or two unbound down quarks and an up quark, what is the probability, if I bring them close together, they stick and form a proton and a neutron, respectively? How much energy (in eV) would be required to do it?

Answer 1

Starting with $uud$, or even $udd$, the probability to form a proton is 100%. This is because baryon number is conserved, and neutrons decay to proton. So if you make a neutron, it's going to be a proton soon, and if you start with baryon number equal to 1, you are going to end with baryon number equal to 1.

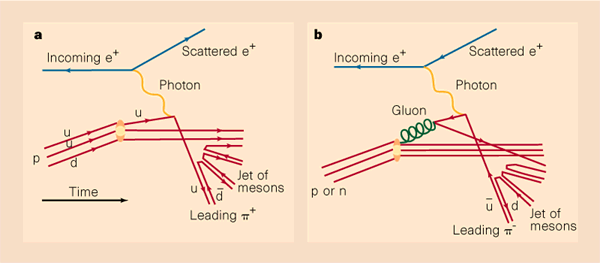
The probability to form only a proton is 0%, as you just can't have 3 free quarks around. It doesn't really makes sense to talk about it, as it cannot exist. You can make a "lone" quark that is not exactly free. This is done by deep inelastic scattering, were a scattering electron scatters off a proton:

Here, in the proton rest frame, the electron energy is much greater than the proton mass, and to 1st order, the exchanged virtual photon has a wavelength much smaller than the proton size (1 fm). It can resolve individual valence and sea quarks, and knock them out of the proton.

The problem is, as it leaves the proton, the energy of the QCD field becomes large enough to produce a $q\bar q$, with the antiquark glomming onto the quark to make a meson. (A similar process called "sparking of the vacuum" can occur in ultra strong electric fields, where it is energetically favorable to make an $e^+e^-$ pair to lower the field energy by more than $2m_ec^2$).

So, imagine simultaneously knocking out 3 quarks from various nucleons in a nucleus, all with the same (or similar) 3-momenta and location, which would give you your free-ish quarks. (Note: the probability of achieving this in the lab in effectively 0). Those quarks will from a proton, because baryon number is conserved. There will also be showers of meson (c.f., the jet).

Answer 2

Since free quarks cannot exist, we shouldn't imagine finding three quarks somewhere and bringing them close together. However, we can imagine producing three quarks in one high-energy interaction, such as those that take place at a particle collider. Your question is then completely legitimate:

If three quarks produced in a high-energy interaction are sufficiently close together* that they will form a hadron together, what are the probabilities that they will form each kind of hadron?

Unfortunately, we do not have a "first principles" understanding of hadronization, the process by which the quarks will become bound together into a hadron. By that I mean, so far no one has been able to start from the fundamental equations describing the strong force and calculate exactly how the hadronization process will play out. We therefore have to resort to phenomenological models, by which I mean descriptions of the process that are based on simple general physical principles, but whose details have to be adjusted in order to match observed data. There are a number of these models that are widely used today and happily they do quite a good job of matching real data. A review of various models can be found in Section 3 of this paper.

One model that you might consider is called the cluster model. In this model, you take a "cluster" of quarks and gluons and calculate its total mass as a single unit. That includes the masses of the quarks, plus also the kinetic energy of all the particles relative to each other. This would be the total energy of the cluster in its rest frame. This total mass will in general not equal the mass of any one hadron, so it can't turn directly into a single hadron. It will instead form two or more hadrons whose total energy equals the total mass of the cluster. The cluster model describes this process by picturing the cluster "decaying" into two hadrons. There may be many pairs of hadrons whose mass is less than the mass of the cluster. In this case, one has to assign a probability for each pair of hadrons to be the outcome of the cluster decay. In this model, this is done by taking the probability to be proportional to the phase space weight for decay to two particles with the masses of those two hadrons.

One thing you know for sure is that the quantum numbers of the system of quarks that you start with must be preserved in the hadrons that are produced. So for example, if you start with $uud$ some possible outcomes of the cluster are:

• proton ($uud$) + neutral pion ($u\bar u/d\bar d$)
• $\Delta^+$ ($uud$) + neutral pion ($u\bar u/d\bar d$)
• neutron ($udd$) + charged pion ($u\bar d$)

But you won't form a neutron ($udd$) and a neutral pion ($u\bar u/d\bar d$). These hadrons of course may themselves be unstable, in which case after some amount of time they will again decay. Those decay probabilities are tabulated in the PDG.

* The measure of how "close together" they are can be made precise in terms of what's known as their transverse momentum, but these details are beyond the scope of my answer and of the level of detail you probably need for your purpose.